- Home
- Physics Problems
- Problems
Solved Problems
A spaceship is on a straight-line path between earth and moon. At what distance from the center of Earth is the net gravitational force on the ship zero? (mass of Earth $M_E$, the mass of moon $M_M$ and $d$ is the distance between the center of Earth and the moon)
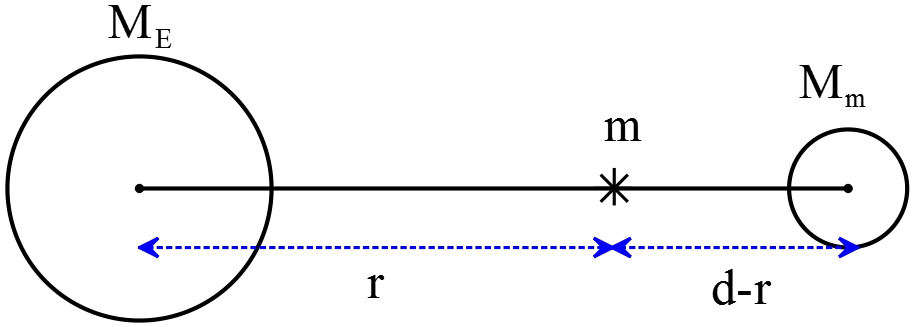
Suppose the desired point to be at distance $r$ from the center of the Earth. Balance gravitational pulls of Earth and the moon on some $m$ placed at this point:
\begin{gather*} G\frac{M_Em}{r^2}=G\frac{M_Mm}{{\left(d-r\right)}^2} \\ \to M_E{\left(d-r\right)}^{2}=M_Mr^{2} \end{gather*} We want to find $r$ in terms of the others. We can rearrange above equation to get the following \[\Big(1-\frac rd \Big)^{2}=\frac{M_M}{M_E}\Big(\frac{r}{d}\Big)^{2}\] Next let $q\equiv \frac{M_M}{M_E}\ ,\ x=\frac{r}{d}$ then we have \begin{gather*} \left(1-x\right)^2=qx^2 \\ \to \left(1-q\right)x^2-2x+1=0 \end{gather*} The above equation is quadratic one, $ax^2+bx+c=0$ with solution \[x=\frac{-a\pm\sqrt{b^2-4ac}}{2a}\]Thus, solution to the above equation is obtained as below \begin{align*} \Rightarrow x_{1,2}&=\frac{2\pm \sqrt{4-4\sqrt{1-q}}}{2\left(1-q\right)} \\ \\ &=\frac{1}{1\pm \sqrt{q}} \end{align*} After substituting, we get the following distances which one is greater than $d$ and the other is greater \[r_{1,2}=\frac{d}{1\pm \sqrt{\frac{M_M}{M_E}}}\]
Turksat $2A$ is a communication satellite, which orbits on a geostationary orbit. The period of the satellites orbiting on the geostationary orbits is about $24$ hours. Therefore, from locations on the surface of the earth, Turksat 2A appears motionless. Calculate the radius of the geostationary orbit $R_G$. (Assume this orbit is a circular orbit. $M_E\sim \ 6\times {10}^{24}{\rm kg}$ and $G=6.6\times {10}^{-11}\ {\rm N.}\frac{{{\rm m}}^{{\rm 2}}}{{\rm k}{{\rm g}}^{{\rm 2}}}$) .
The gravitational force between satellite and earth provides the centripetal force on the satellite. Therefore, apply Newton's 2${}^{nd}$ law in the radial direction:
\begin{align*} \Sigma F_r&=ma_r \\ F_G &=ma_r \\ G\frac{M_Em}{R^2}&=m\frac{v^2}{R} \\ \Rightarrow R&={\left(\frac{GM_E}{\omega}\right)}^{\frac{1}{3}}\end{align*}
Recall that in circular motion $v=R\omega=R\frac{2\pi}{T}$, where $T$ is the period of the motion.
For a satellite with period of $24$ hours, the angular velocity is
\begin{gather*} \omega=\frac{2\pi}{T}={\rm \frac{2\pi}{24\,h\times 60\,m \times 60\,s}} \\ \\ \sim \ 7.27\times {10}^{-5}\ \frac{{\rm rad}}{{\rm s}} \end{gather*}
$\Rightarrow R=42150\ {\rm km}$ from center of the Earth.
Since the radius of the earth is about $6400\,{\rm km}$, then the Turksat is about $35750{\rm \ km}$ from the surface of the earth.
The gravitational acceleration at the surface of a planet is $22.5 \, m/s^2$.
Find the acceleration at a height above the surface equal to the planet's radius.
Note: an object at a distance $h$ above a planet's surface experiences a gravitational force with the following acceleration \[a_g=G\frac{M}{{\left(R+h\right)}^2}\] which is the consequence of applying newton's second law in the rotational system.
In the above equation, $R$ and $M$ are the planet's radius and mass, respectively. $G$ is the universal gravitational constant. Hence, at the surface level the gravitational acceleration is \begin{align*} a_g&=G\frac{M}{R+h}\\ \\ &=G\frac{M}{R+0}\\ \\ &=22.5\ \end{align*}
Now insert the values to find the $a_g$ at a distance $R$ above the surface.
\begin{align*} a^{'}_g&=G\frac{M}{R+R} \\ \\ &=\frac{1}{2}\left(G\frac{M}{R}\right)\\ \\&=\frac{1}{2}(22.5)\\ \\ &=11.25 \quad {\rm \frac{m}{s^2}}\end{align*} where a prime ($'$) inserted to distinct acceleration at the surface and above the surface.
The radius of a spherical planet is $R$ and its mass is $M$. At what distance above the planet's surface will the acceleration of gravity be exactly one-tenth of its value at the surface?
The gravitational acceleration of an object with mass $M$ at distance $r$ from its center is given by $g=GM/r^2$ so \begin{align*} g_r &=\frac{1}{10}g_R \\ \\ G\frac{M}{{\left(r+R\right)}^2} &=\frac{1}{10}G\frac{M}{R^2} \\ \\ \Rightarrow 10R^2&={\left(r+R\right)}^2\end{align*}
Taking the square root of both sides, we obtain two solutions. \begin{align*} \sqrt{10R^2}&=\sqrt{{\left(r+R\right)}^2} \\ \Rightarrow \pm \sqrt{10}R&=r+R \end{align*} Considering both signs and solving for unknown $r$, we get \[\Rightarrow \left\{ \begin{array}{rcl}+\sqrt{10}R&=&r+R\Rightarrow r=R\left(\sqrt{10}-1\right)=2.16R \\ -\sqrt{10}R&=&r+R\Rightarrow r=-R(\sqrt{10}+1) \end{array}\right.\] The second result is not accepted since $r<0$ !

You are on the home planet of the Klingon Empire. You drop a ball of mass $1.24\, {\rm kg}$ from a $334\, {\rm m}$ tower and it takes $6.54\, {\rm s}$ to reach the ground. The diameter of the Klingon home world is $1.55$ times the diameter of the earth. What is the mass of the Klingon home world?
First, find the gravitational acceleration of the planet, then using the definition of the gravitational acceleration calculate the mass of the planet.
Use the displacement kinematic equation $y=-\frac 12 gt^2 +v_0t+y_0$, and set the initial velocity zero $v_0=0$ since ``the ball is dropped'' means that its initial velocity is zero. Now, solve this equation for $g$, to find its numerical \begin{gather*} y=\frac{1}{2}gt^2+v_0t+y_0\\\\ g=\frac{2y}{t^2}=\frac{2\times 334}{(6.54)^2}=15.61\quad {\rm m/s^2}\end{gather*}
Where we have chosen the initial position of the ball as the base, so $y_0=0$.
On the other side, the gravitational acceleration is defined as $g=\frac{GM}{R^2}$, where $R$ is the radius of the planet. Solving this for $M$, we will have \begin{align*} M&=\frac{gR^2}{G}\\\\&=\frac{g(1.55R_e)^2}{G}\\\\&=\frac{15.61\times {\left(1.55\times 6.37\times {10}^6\right)}^2}{6.67\times {10}^{-11}}\\\\&=2.28\times{10}^{25}\quad{\rm kg}\end{align*}
What is the speed of a satellite of mass $ m=1.0 \times 10^{4}\, {\rm kg}$ on a circular orbit around the moon at a distance of ${\rm 4}{{\rm R}}_{moon}$ from the moon's surface?
The gravitational force between the satellite and the Moon acts as a centripetal force. Recall that in the circular motion we have $F_r=ma_r=mv^2/r$. Therefore, \begin{align*} F_G&=F_r\\\\ \frac{GM_Mm}{r^2}&=\frac{mv^2}{r}\\\\ \Rightarrow v&=\sqrt{\frac{GM_M}{r}}\\\\ &=\sqrt{\frac{GM}{4R_M}}\\\\&=\frac{1}{2}(g_{moon})^{\frac 12}\end{align*}
This was a question on circular motion. For practicing more problems on this topic, read the following:
Uniform circular motion problems
Astronauts put their spaceship into orbit about a planet. They find that the acceleration of gravity at their orbital altitude is half that at the planet's surface. How far above the planet's surface are they orbiting? Answer in terms of the radius of the planet.
The surface gravity of a planet with mass $M$ and radius $R$ is given by \[a_g=G\frac{M}{R^2}\] And at the height of $h$ above its surface is
\[a_g=G\frac{M}{{\left(R+h\right)}^2}\] In this problem, we know that $a_g h=\frac{1}{2}a_g(R)$ so
\begin{gather*} G\frac{M}{(R+h)^2}=\frac{1}{2}G\frac{M}{R^2}\\\\ \Rightarrow (R+h)^2=2R^2\end{gather*}
Taking the square root of both sides, we obtain \[R+h=\pm \sqrt{2}R \] The plus sign sign, gives \[R+h=+\sqrt{2}R \Rightarrow h=(\sqrt{2}-1)R\] and the minus sign get \[R+h=-\sqrt{2} \Rightarrow h=-(1+\sqrt{2})R\] Choosing the minus sign doesn't give the correct answer since $h$ is obtained negative. Therefore, the orbital altitude of the Astronauts is $h=0.41\,R$
Consider the ring-shaped body of radius $a$ and mass $M$ shown in the figure. A particle of mass $m$ is placed a distance $x$ from center of the ring, along a line through the center of the ring and perpendicular to it.
(a) Calculate the gravitational potential energy $U$ of this system. Take the potential energy to be zero when the two objects are very far apart. Recall that we can write $dU=-\frac{Gm}{r}dM$ where $r={\left(x^2+a^2\right)}^{\frac{1}{2}}$ as we integrate around the ring.
(b) Show that your answer in part (a) reduces to the point masses result when $x$ is much larger than the radius $a$ of the ring.
(c) Use $F_x=-dU/dx$ to find the magnitude and direction of the force on the particle.
(d) Show that your answer in part (c) reduces to the expected result when $x$ is much larger than $a$. What are the values of $U$ and $F_x$ when $x=0$ and why?

(a) The gravitational potential energy of two point particles of masses $m$ and $M$ that are separated by a distance of $r$ is given by the following equation \[U=-G\frac{m_1m_2}{r^2}\]
First, calculate the gravitational potential energy of an infinitesimal element of the ring $dM$ and mass $m$ then integrate it around the ring to find the total potential.
\begin{gather*} dU=-\frac{Gm}{r}dM\\\\ U=-\frac{Gm}{r}\int{dM}=-\frac{GmM}{\sqrt{x^2+a^2}}\end{gather*}
(b) If $x\gg a$, then using the binomial theorem ${\left(1+u\right)}^n=1+nu+\dots \ $ ($\left|u\right|$ must be smaller than $1$), we obtain
\begin{align*} U&=-\frac{GmM}{\sqrt{x^2+a^2}}\\\\&=-\frac{GmM}{x{\left(1+\frac{a^2}{x^2}\right)}^{\frac{1}{2}}}\\\\&=-\frac{GmM}{x}{\left(1+\frac{a^2}{x^2}\right)}^{\frac{1}{2}}\\\\&=-\frac{GmM}{x}\left(1-\frac{a^2}{2x^2}\right)\end{align*}
(c) To find the force, we differentiate the potential energy \begin{align*} F_x&=-\frac{dU}{dx}\\\\&=GmM\left(-\frac{1}{2}\frac{2x}{{\left(x^2+a^2\right)}^{\frac{3}{2}}}\right)\\\\&=GmM\frac{x}{{\left(x^2+a^2\right)}^{\frac{3}{2}}}\end{align*}
If we assume $x\gg a$ then \begin{align*} GmM\frac{x}{{\left(x^2+a^2\right)}^{\frac{3}{2}}}&=\frac{GmM}{x^2}{\left(1+\frac{a^2}{x^2}\right)}^{-\frac{3}{2}}\\\\&=\frac{GmM}{x^2}\left(1-\frac{3}{2}\frac{a^2}{x^2}+\dots \right)\\\\ &\cong \frac{GmM}{x^2}\end{align*}
(d) \[U\left(x=0\right)=-GmM/a\]
\[F_x\left(x=0\right)=GmM\left[\frac{x}{{\left(x^2+a^2\right)}^{\frac{3}{2}}}\right]=0\]
The contribution from $dM$ around the ring cancels the net force on the particle.
MOST USEFUL FORMULA IN GRAVITY:
Newton's law of gravity:
\[F=-G\frac {m_1m_2}{r^2}\]
Universal gravitational constant:
\[G=6.67\times 10^{-11}\,\mathrm {N.m^{2}/kg^2}\]
Gravitational potential energy:
\[U(r)=-\frac{GmM}r\]
© 2015 All rights reserved. by Physexams.com
AP® is a trademark registered by the College Board, which is not affiliated with, and does not endorse, this website.
