- Home
- Physics Problems
- Problems
Solved Problems
Two horizontal forces $F_1=100\,\rm{N}$ and $F_2=25\, \rm{N}$ act on the 5 kg block as indicated in the figure. If $\theta =60^\circ $, what is the horizontal acceleration of the block and the normal force acting on it? Neglect friction.
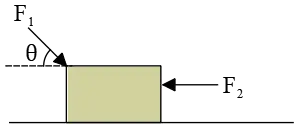
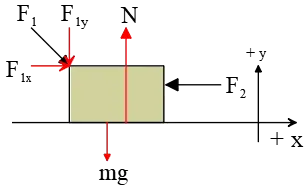
First decompose the force $F_1$ into its components $F_{1x}$ and $F_{2x}$, then apply Newton's second law to the chosen axes as follows \begin{gather*} \Sigma F_x=ma\\ F_{1x}-F_2=ma \\ \Rightarrow F_1\,\cos \theta-F_2=ma\end{gather*} Substituting the numerical values into the above relation gives us \begin{gather*} 100\,\cos 60^\circ-25=5a_x \\\\ \Rightarrow a_x=\frac{50-25}{5}=5\,{\rm m/s^2}\end{gather*} Since in the vertical direction there is no motion, so the vertical acceleration is zero, i.e., $a_y=0$. Draw a free-body diagram and apply the motion law to the vertical direction.
\begin{gather*} \Sigma F_y=ma_y \\ N-F_{1y}-mg=0 \\ N=mg+F_1\,\sin \theta=5\times 9.8+100\,\sin 60^\circ \end{gather*} Hence, \[\boxed{N=136\quad \rm{N}}\]
Refer to the following page for more problems in the AP Physics 1 exam
Consider the situation depicted below where the base of the triangular inclined  plane structure has length $\mathrm{L}$. There is no friction between the mass $m_1$ and the inclined plane. The coefficient of kinetic friction between the inclined plane (with mass $m_2$) and the ground is ${\mu }_k$.
plane structure has length $\mathrm{L}$. There is no friction between the mass $m_1$ and the inclined plane. The coefficient of kinetic friction between the inclined plane (with mass $m_2$) and the ground is ${\mu }_k$.
What is the magnitude of the force $\mathrm{F}$ necessary to have the mass $m_1$ stationary with respect to the moving inclined plane?
In the vertical direction, because the mass is neither moving up nor down: 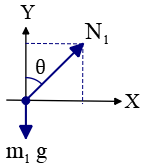 \begin{gather*} \Sigma F_y=0 \\ m_1g=N_1\,\cos \theta \\ \Rightarrow\quad N_1=\frac{m_1g}{\cos \theta}\end{gather*}
\begin{gather*} \Sigma F_y=0 \\ m_1g=N_1\,\cos \theta \\ \Rightarrow\quad N_1=\frac{m_1g}{\cos \theta}\end{gather*}
Horizontal direction: \begin{gather*} \Sigma F_x=ma \\ m_1a=N_1\,\sin \theta \\ \Longrightarrow a=g\,\tan \theta\end{gather*}
Now apply Newton's second law to the mass $m_2$
\[\mathrm{\Sigma }F_x=ma\Rightarrow \ F-{\mu }_kN_2-N_1\,{\sin \theta \ }=m_2a\]
\[N_2=N_1\,{\cos \theta \ }+m_2g\]
\[\therefore F=m_2a+N_1\,{\sin \theta \ }+{\mu }_kN_2\]
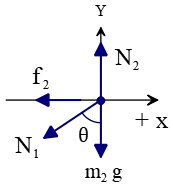
If we substitute $a\ $and $N_2$ from above into the $F$, we get \begin{align*} F &=m_2g\,\tan \theta+N_1\,\sin \theta+(N_1\,\cos \theta+m_2g)\mu_k\\&= m_2g(\mu_k+\tan \theta)+N_1\,(\sin \theta+\mu_k\,\cos \theta)\\ &= m_2g(\mu_k+\tan \theta)+m_1g(\mu_k+\tan \theta) \end{align*} Hence, we have
\[\Rightarrow F=(\mu_k+\tan \theta)(m_1+m_2)g\]
Note: in above, $N_1$ is due to Newton's third law of motion.
A car drives around a flat curve with radius $r=85\ \mathrm{m}$ at a speed of $v=20\ \mathrm{m/s}$. What is the minimum coefficient of static friction required to keep the car from slipping?
Since we want rolling without slipping and there is a circular motion so the static friction provides the centripetal force acting on the car. Apply Newton's 2${}^{nd}$ law for rotational motions to the car as $\mathrm{\Sigma }F_r=ma_r$
\[F_r=f_{s,max}\Rightarrow \ {\mu }_sN=\frac{mv^2}{r}\Rightarrow \ {\mu }_s=\frac{mv^2}{rN}\]
Since the car move in the flat circle, $N=mg$. Therefore
\[\therefore \ {\mu }_s=\frac{v^2}{rg}=\frac{{20}^2}{\left(85\right)\left(9.8\right)}=0.48\ \]
This figure illustrates a centrifuge machine.The structure consists of a horizontal and rotating circular metal disk of diameter D from which medicine of mass $M$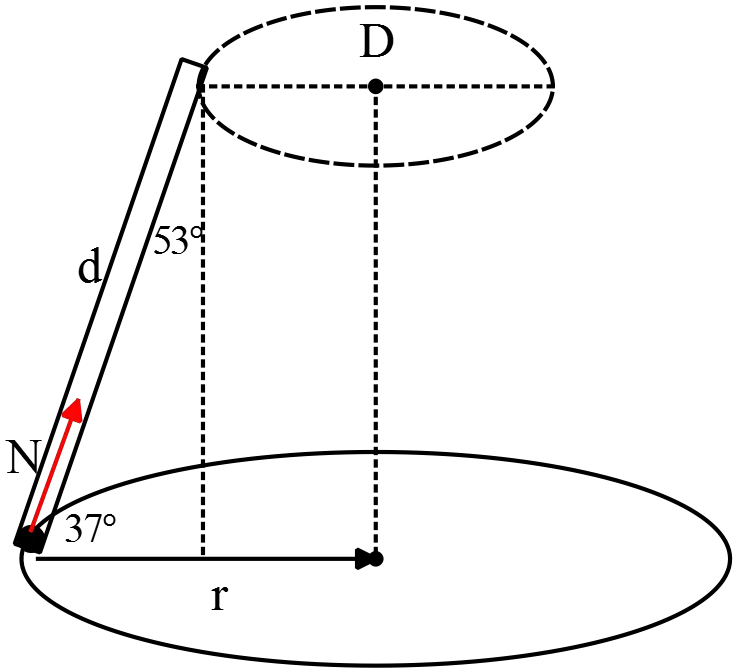 are suspended at the bottom of massless tube of length d. when the system rotates at constant speed, the tube makes an angle $\theta $ with vertical.
are suspended at the bottom of massless tube of length d. when the system rotates at constant speed, the tube makes an angle $\theta $ with vertical.
Consider $D=0.08\ \mathrm{m}$ , $d=0.1\,\mathrm{m}$ , $\theta =53{}^\circ $
(a) If the magnitude of the normal force ($N$) acting on the medicine is $0.98\ \mathrm{N}$, finding the mass $M$ of the medicine in the tube,
(b) Find the linear speed ($v$) of the rotating tube.
First, draw a free-body diagram, decompose the normal force $\vec{N}$ into 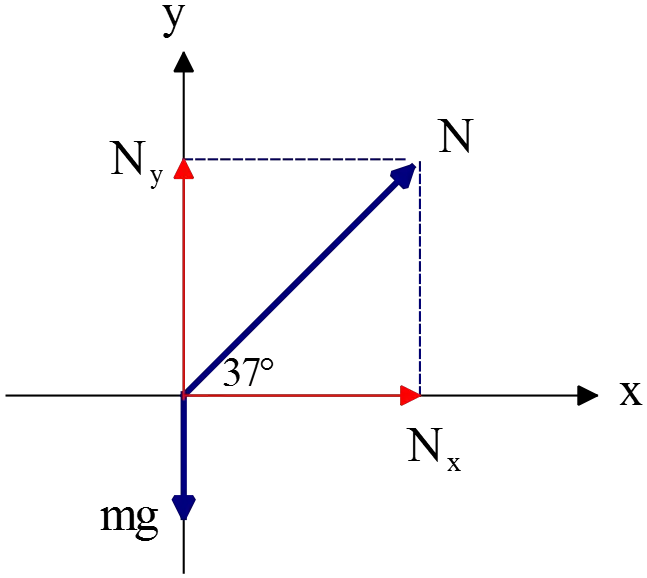 its components. Then apply Newton's second law of motion in the $x$ and $y$ direction.
its components. Then apply Newton's second law of motion in the $x$ and $y$ direction.
(a) $\mathrm{\Sigma }F_y=N\,{\sin 37{}^\circ \ }-mg=0\ $(mass doesn't move in the $y$-direction)
\[N\,{\sin 37{}^\circ \ }=mg\]
\[m=\frac{N{\sin 37{}^\circ \ }}{g}=\frac{\left(0.98\right)\left(0.6\right)}{9.8}=0.06\mathrm{kg}\]
(b) Since the mass $M$ is rotated around a circle so there is a centripetal force in the $x$ direction that is provided by the $x$ component of the normal force $\vec{N}$. Recall that the centripetal acceleration is $a_r=v^2/r$, where $r$ is the radius of the circular motion. thus
\[\mathrm{\Sigma }F_x=ma_x\]
\[N{\cos 37{}^\circ \ }=\frac{mv^2}{r}\mathrm{\to }v^2=\frac{Nr{\cos 37{}^\circ \ }}{m}\]
Here, as shown in the figure,
\[r=\frac{D}{2}+d\,{\sin \theta \ }=\frac{0.08}{2}+0.1{\sin 53{}^\circ \ }=0.04+0.08=0.12\mathrm{m}\]
Therefore $v=\sqrt{\frac{Nr\,{\cos 37{}^\circ \ }}{m}}=\sqrt{\frac{\left(0.98\right)\left(0.12\right)\left(0.8\right)}{0.06}}=1.25\ \mathrm{m/s}$
An automobile travelling $40\ \mathrm{m/s}$ goes around a curve with a radius of $200\ \mathrm{m}$.
(a) What is the acceleration of the automobile?
(b) If the road is not banked, what coefficient of friction is required to keep the automobile from sliding across the road?
(c) At what angle must the road bank to prevent sliding in the absence of friction at tis speed?
(d) At what angle calculated above, what coefficient of friction is required to keep a parked car from sliding off the road?
(e) If the moving automobile consumes $50\ $horsepower ($37.7\ \mathrm{kW}$), what is the drag force acting on it?
(a) In the circular motions, the centripetal acceleration is given by $a_r=v^2/r$. So
\[a_r=\frac{{\left(40\right)}^2}{200}=8\ \mathrm{m/s\ }\]
(b) This centripetal acceleration is provided by the static friction therefore, by the second law we have $\mathrm{\Sigma }F_r=ma_r$
\[f=ma_r\Rightarrow \mu N=ma_r\Rightarrow \mu mg=ma_r\]
\[\Rightarrow \mu =\frac{a_r}{g}=\frac{8}{9.8}=0.816\]
Since the road is not banked, so the normal force $N$ balances the downward force of gravity i.e. $N=mg$.
(c) In this case, the horizontal component of the normal force $N\,{\sin \theta \ }$ provides the centripetal acceleration so apply Newton's second law in the 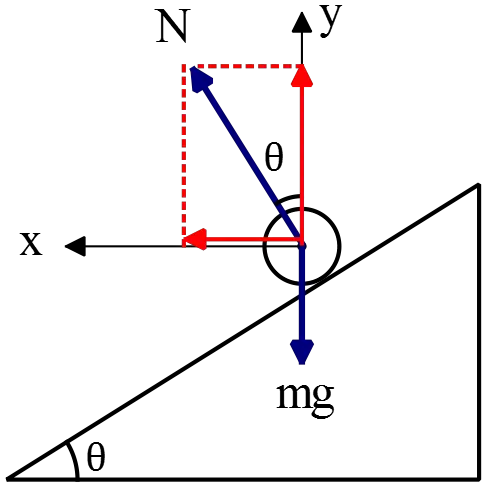 $x$ and $y$ directions:
$x$ and $y$ directions:
\[\mathrm{\Sigma }F_x=ma_r\Rightarrow N\,{\sin \theta \ }=\frac{mv^2}{r}\]
\[\mathrm{\Sigma }F_y=0\Rightarrow N\,{\cos \theta \ }=mg\]
By eliminating $N$ from above equations and solving for $\theta $, we get
\[{\tan \theta \ }=\frac{v^2}{rg}\to \theta ={{\tan}^{-1} \left(\frac{v^2}{rg}\right)\ }=39.2{}^\circ \]
(d) Let the direction of the motion to be parallel to the incline plane as the $x$ axis. We want the parked car does not slide (move) so the static friction force 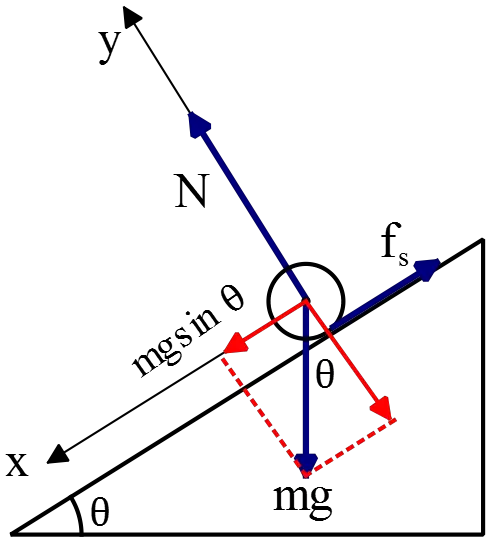 must be balanced with the parallel component of the weight with the inclined plane i.e. $mg\,{\sin \theta \ }$
must be balanced with the parallel component of the weight with the inclined plane i.e. $mg\,{\sin \theta \ }$
\[f_{s,max}=mg\,{\sin \theta \ }\to \ \mu_smg\,{\cos \theta \ }=mg\,{\sin \theta \ }\]
\[\Rightarrow \ \mu_s ={\tan \theta \ }\]
\[\mu_s ={\tan 39.2{}^\circ \ }=0.816\]
(e) When an object moves through a fluid such as water or air, the fluid exerts an opposing force, which is called drag force. The power is given by $P=\vec{F}.\vec{v}$. therefore, the power required to overcome the drag force is
\[P=F_{drag}v\Rightarrow F_{drag}=\frac{P}{v}=\frac{37300}{40}=933\ \mathrm{N}\]
A mass of $m=8\,{\rm kg}$ is being pulled up a $30\,{\rm m}$ tall elevator shaft by an engine that exerts a force of $100\,\rm{N}$. How long does it take to reach the top of the shaft if it starts at rest?
First, from Newton's second law find the acceleration of the mass and then  from kinematic relations finds the time required.
from kinematic relations finds the time required.
Let the direction of motion of the elevator be positive of the coordinate system.
\begin{gather*} \Sigma F=ma\to F-mg=ma \\\\ \Rightarrow a=\frac{F}{m}-g=\frac{100}{8}-9.8=2.7\,{\rm m/s^2} \end{gather*} Now, using the relevant kinematic equation below, to find the required time \begin{gather*} y=\frac{1}{2}at^2+v_0t+y_i \\\\ \Delta y=\frac{1}{2}at^2+(0)t\\\\ \Rightarrow t=\sqrt{\frac{2\Delta y}{a}}=\sqrt{2\times \frac{30}{2.7}}=4.7\,{\rm s} \end{gather*}
Blocks A and B each have a mass $m=10\ \mathrm{kg}$. Determine the largest horizontal force $\vec{P}$ that can be applied so that A will not slip on B. The  coefficient of static friction between A and B is ${\mu }_s=0.35$. The angle shown is$\theta =40{}^\circ $. Neglect any friction between B and C.
coefficient of static friction between A and B is ${\mu }_s=0.35$. The angle shown is$\theta =40{}^\circ $. Neglect any friction between B and C.
First, for each of the blocks draw a free body diagram and then apply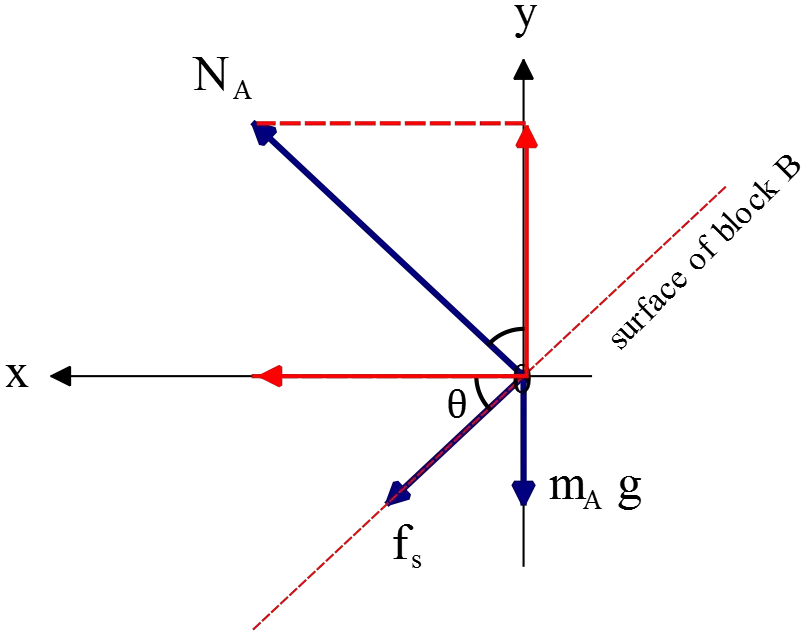 Newton's 2${}^{nd}$ law to them. Since we want block A does not slide on the B, so its acceleration must be same as that of block B i.e. $a_A=a_B=a$.When the block B moves to the left, the block A also moves to the up along the B so the static friction force between them is down along the block B. For block A we have,
Newton's 2${}^{nd}$ law to them. Since we want block A does not slide on the B, so its acceleration must be same as that of block B i.e. $a_A=a_B=a$.When the block B moves to the left, the block A also moves to the up along the B so the static friction force between them is down along the block B. For block A we have,
\[\mathrm{\Sigma }F_y=0\to N_A{\cos \theta \ }-f_s\,{\sin \theta \ }-m_Ag=0\]
\[f_{s,max}={\mu }_sN_A\to N_A\,\left({\cos \theta \ }-{\mu }_s\,{\sin \theta \ }\right)=m_Ag\]
\[\therefore N_A=\frac{m_Ag}{\left({\cos \theta \ }-{\mu }_s\,{\sin \theta \ }\right)}\]
\[\mathrm{\Sigma }F_x=m_Aa_A\to N_A\,{\sin \theta \ }+f_s\,{\cos \theta \ }=ma\]
\[\Rightarrow \ ma=N_A\,({\sin \theta \ }+{\mu }_s\,{\cos \theta \ })\]
\[\therefore a=g\frac{{\sin \theta \ }+{\mu }_s{\cos \theta \ }}{\left({\cos \theta \ }-{\mu }_s{\sin \theta \ }\right)}\ \ ,\ \ *\ \]
Now write the equations of motion for block B:
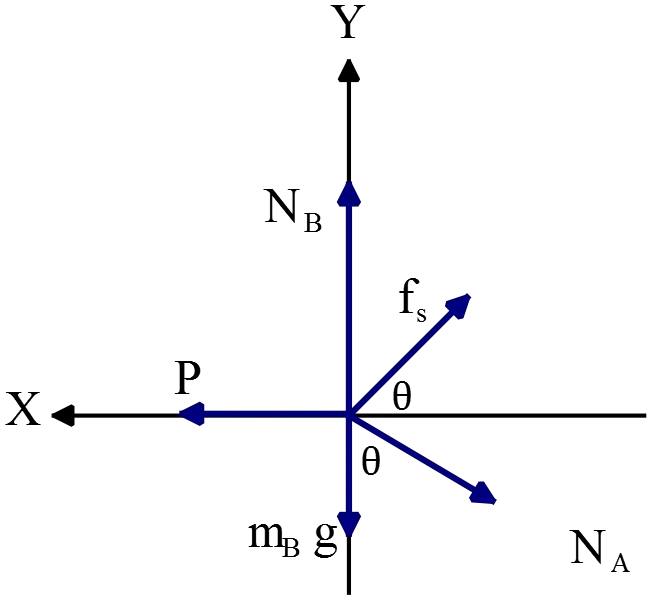
\[\mathrm{\Sigma }F_x=m_Ba_B\to \ P-f_s{\cos \theta \ }-N_A{\sin \theta \ }=m_Ba\]
\[P-N_A\left({\mu }_s\,{\cos \theta \ }+{\sin \theta \ }\right)=m_Ba\]
\[P-m_A\underbrace{g\frac{{\mu }_s\,{\cos \theta \ }+{\sin \theta \ }}{{\cos \theta \ }-{\mu }_s{\sin \theta \ }}}_{*\ \Rightarrow a}=m_Ba\]
Since $m_A=m_B=m$ so
\[P=2ma=2m\ g\frac{{\sin \theta \ }+{\mu }_s{\cos \theta \ }}{\left({\cos \theta \ }-{\mu }_s{\sin \theta \ }\right)}\]
Now substitute the given data: $m=10\ \mathrm{kg\ ,\ }\theta=40{}^\circ \ ,\mu_s=0.35$, we obtain
\[P=330\ \mathrm{N}\]
Note: $N_A$ is the normal force acting on the block A and due to the Newton's 3${}^{rd}$ law its reaction acts on the block B. (see figures)
A large block $P$ with mass $M$ attached to a light spring executes horizontal, simple harmonic motion (SHM) as it slides across a frictionless surface with a frequency $f$. Block B with mass $m$ rests on it as shown in the figure, and the 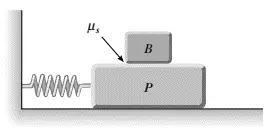 coefficient of the static friction between the two is ${\mu }_s$. Find the maximum amplitude of oscillation of the system such that the top block $B$ will not slip on the bottom block $P$?
coefficient of the static friction between the two is ${\mu }_s$. Find the maximum amplitude of oscillation of the system such that the top block $B$ will not slip on the bottom block $P$?
The maximum acceleration of the lower block cannot exceed the maximum acceleration that can be given to the block B by the friction force since the two blocks do not move against each other thus applying Newton’s 2nd law to block B gives (note: we want block B does not slip on the P, so we only have the static friction $f_{s\ max}$)
\begin{gather*} f_s\le f_{s\ max}\left({\mu }_sN\right)={\mu }_s\,mg \\\\ \Sigma F_x=ma_x \\\\ \mu_s\,mg=ma_{max}\\\\ a_{max}={\mu }_sg\quad (*) \end{gather*}
Blocks B and P have simple harmonic oscillation. From Hooke's law
\begin{align*} F&=kx\\\\ (m+M)a&=kx\\\\ \Rightarrow a_{max}&=\frac{k}{M+m}x_{max}\\\\&=\frac{k}{M+m}A\quad (**)\end{align*}
Substituting ${\mu }_sg$ for $a_{max}$,we obtain \[(*),(**)\Rightarrow A=\frac{M+m}{k}{\mu }_sg\]
Recall that $\omega =2\pi f=\sqrt{\frac{k}{M_{tot}}}$ so $k=4{\pi }^2\left(M+m\right)f^2$. Substituting this into the above expression for $A$, we will have \[A=\frac{M+m}{4{\pi }^2(M+m)f^2}{\mu }_sg=\frac{{\mu }_sg}{4{\pi }^2f^2}\]
For practicing more on friction problems, refer
Coefficient of friction force problems and solutions
In the figure on the left, a $0.5\,\mathrm{kg}$ block is suspended at the 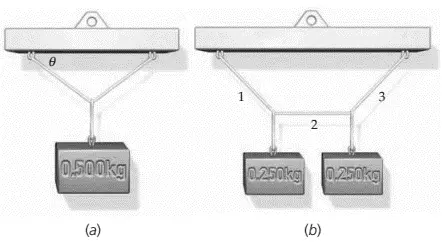 midpoint of a $1.25\,\mathrm{m}$ long string. The ends of the string are attached to the ceiling at points separated by $1.00\,\mathrm{m}$.
midpoint of a $1.25\,\mathrm{m}$ long string. The ends of the string are attached to the ceiling at points separated by $1.00\,\mathrm{m}$.
(a) What angle does the string make with the ceiling?
(b) What is the tension in the string?
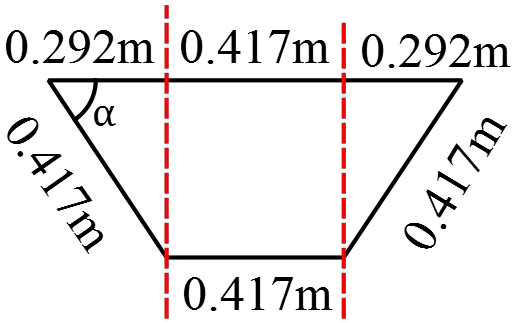
(c) The $0.5\, \mathrm{kg}$ block is removed and two $0.25\,\mathrm{kg}$ blocks are attached to the string such that the lengths of the three string segments re equal (right figure). What is the tension in each segment of the string?
(a) Use the following geometry to find the angle $\theta $ in the figure $a$ and $\alpha $ in $b$
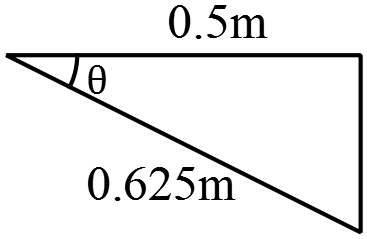
\[{\cos \theta \ }=\frac{0.5}{0.625} \Rightarrow \theta =37{}^\circ\]
\[{\cos \alpha \ }=\frac{0.292}{0.417}\Rightarrow \alpha =46{}^\circ \]
(b) Find the tension in the string by applying equilibrium condition $\mathrm{\Sigma }\vec{F}=m\vec{a}$ to the knot where the strings are 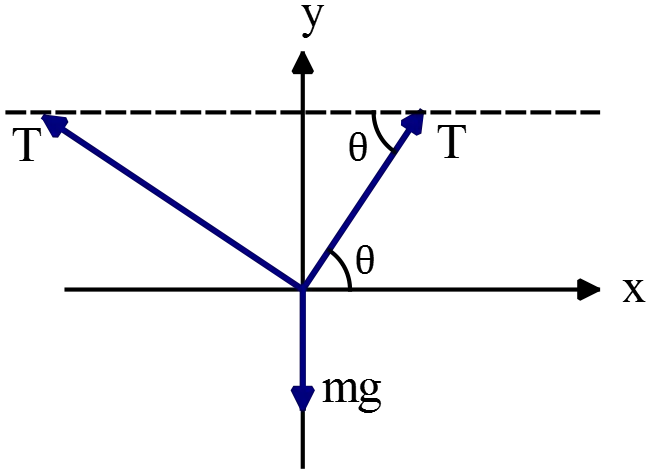 connected
connected
Decompose $T$ into horizontal and vertical components. Since there is no motion, $a=0$, apply Newton's 2${}^{nd}$ law to the knot.
\[\mathrm{\Sigma }F_x=2T{\sin \theta \ }-mg=0\]
\[T=\frac{mg}{2\,{\sin \theta \ }}=\frac{0.5\times 9.8}{2{\sin 37{}^\circ \ }}=4.1\mathrm{N}\]
(c) apply Newton's 2${}^{nd}$ law to the knot in the figure $b$
\[\mathrm{\Sigma }F_y=0\to \ T_3\,{\sin \alpha \ }=mg\to T_3=\frac{mg}{{\sin \alpha \ }}=\frac{0.25\times 9.8}{{\sin 46{}^\circ \ }}=3.4\mathrm{N}\]
\[\mathrm{\Sigma }F_x=0\to \ T_2=T_3\,{\cos \alpha \ }=\frac{mg}{{\sin \alpha \ }}{\cos \alpha \ }=2.4\mathrm{N}\]
From the symmetry of the problem, $T_1=T_3=3.4\ \mathrm{N}$
A box of mass $m_2=3.5\,\rm{kg}$ rests on a frictionless horizontal shelf and is attached by strings to boxes of masses $m_1=1.5\,\rm{kg}$ and 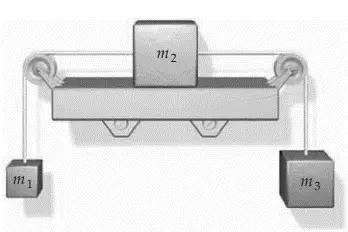 $m_3=2.5\,\rm{kg}$ as shown in the figure below. Both pulleys are frictionless and massless. The system is released from rest. After it is released, find:
$m_3=2.5\,\rm{kg}$ as shown in the figure below. Both pulleys are frictionless and massless. The system is released from rest. After it is released, find:
(a) The acceleration of each of the boxes
(b) The tension in each string
(a) All of the boxes have the same acceleration. So for each of the three 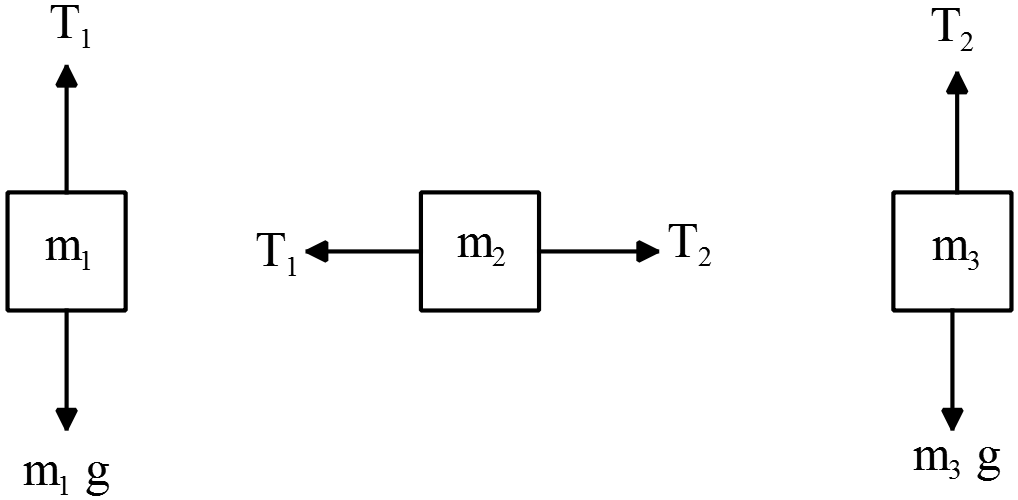 boxes apply Newton's 2nd law separately. Note that because $m_3>m_1$, we can choose the positive direction as clockwise.
boxes apply Newton's 2nd law separately. Note that because $m_3>m_1$, we can choose the positive direction as clockwise.
Box $1$:
\begin{gather*} \Sigma F_{1y}=0 \\ T_1-m_1g=m_1a \\ \Longrightarrow T_1=m_1(a+g)\end{gather*}
Box 2:
\[\Sigma F_x=m_2a \to \quad T_2-T_1=m_2a\]
Box 3: \begin{gather*} \Sigma F_{y_3}=m_3a\to \ m_3g-T_2=m_3a \\\\ \Rightarrow T_2=m_3(g-a)\end{gather*}
From the above relations we have
\[\left\{ \begin{array}{rcl} T_1 & = & m_1(a+g) \\ T_2-T_1 & = & m_2a \\ T_2 & = & m_3(g-a) \end{array}\right.\] Therefore, subtracting $T_2$ from $T_1$ and set equal to the middle equation ($m_2a$), we will have \begin{gather*} m_3(g-a)-m_1(g+a)=m_2a\\\\ \Rightarrow \quad a=\frac{m_3-m_1}{m_1+m_2+m_3}g\end{gather*}Substituting the numerical values into the above equation gives us \[a=\frac{2.5-1.5}{1.5+2.5+3.5}(9.8)=1.3\,{\rm m/s^2}\]
(b) Substitute the above acceleration $a$ into the $T$'s relations:
\begin{gather*} T_2=m_3(g-a)=(2.5)(9.8-1.3)=21\quad \rm{N}\\\\ T_1=m_1(g+a)=1.5(9.8+1.3)=17\quad {\rm N}\end{gather*}
A frictionless surface is inclined an angle of $30{}^\circ $ to the horizontal. A $m_1=270\,\rm{g}$ block on the ramp is attached to a 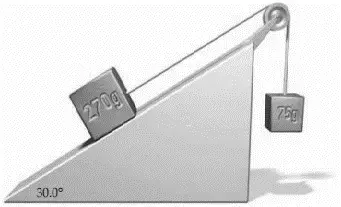 $m_2=75\,\rm{kg}$ block using a pulley, as shown in the figure below.
$m_2=75\,\rm{kg}$ block using a pulley, as shown in the figure below.
(a) Draw two free-body diagrams, one for $270\rm{g}$ block and the other for the $750\,\rm{g}$ block.
(b) Find the tension in the string and the acceleration of the $270\, \rm{g}$ block.
(c) The $270\,\rm{g}$ block is released from the rest. How long does it take for it to slide a distance of $1.00\,\mathrm{m}$ along the surface? Will it slide up the incline, or down the incline?
(a) Because $m_1g\,{\sin 30^\circ} > m_2g$ so the $270\,{\rm g}$ block slides down and thus we can choose the positive direction as counter clockwise. Forces acting on the hanging block are gravity and tension of the rope and the forces acts on the sliding block is gravity ($m_1\ g{\sin 30^\circ}$), normal force($N$) and tension ($T$).
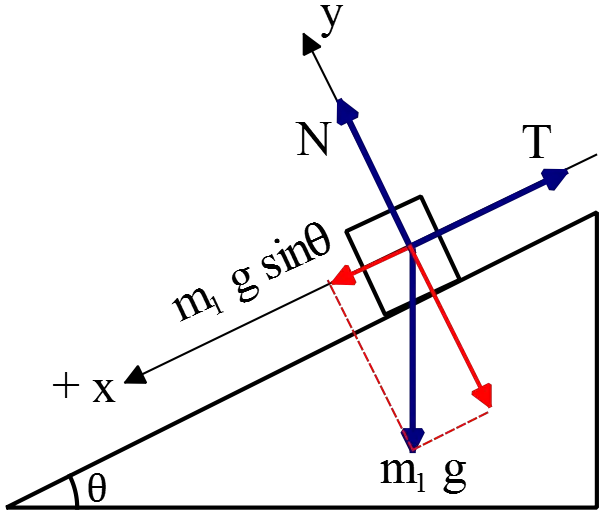
Apply the Newton's second law to $m_1$ and $m_2$.
To $m_1$:
\begin{gather*} \Sigma F_{along\ ramp}=m_1a \\\\ m_1g\,\sin 30^\circ-T=m_1a \\\\ T=m_1(g\,\sin 30^\circ-a) \, \quad (1) \\\\ \Sigma F_{normal}=0 \\\\ \to N-m_1g\,\cos 30^\circ=0 \end{gather*} To $m_2$:
\[\mathrm{\Sigma }F=m_2a\]
\[T-m_2g=m_2a\] 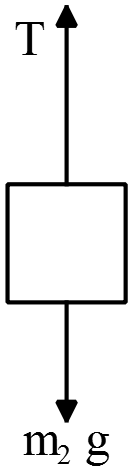
\[\therefore T=m_2(g+a)\ \ ,\ \ (2)\]
If we set the two tension equal each other, we obtain
\[\left\{ \begin{array}{rcl} T & = &m_1 (g\,\sin 30^\circ-a) \\ T & = & m_2(g+a) \end{array} \right. \] Canceling the tension $T$ from both sides, gives \[ m_1(g\,\sin 30^\circ-a)=m_2(g+a)\] Hence, solving for $a$ in the above and substituting the numerical values into it, we will have \begin{align*} a&=\frac{m_1\,{\sin 30^\circ}-m_2}{m_1+m_2}g\\\\ &=\frac{0.135-0.075}{0.270+0.075}(9.8) \\\\ &=1.7\quad {\rm m/s^2}\end{align*} Using $a=1.7\,{\rm m/s^2}$, one can determine the tension on the rod as below \begin{align*}T&=m_2(g+a) \\ &=0.075(1.7+9.8) \\ &=0.86\rm{N}\end{align*}
(b) See above.
(c) To find the elapsed time, we can use the kinematic relation such as:
\[x=\frac{1}{2}at^2+v_0t+x_0\]
In this problem $x-x_0=1\mathrm{m}$ and $v_0=0$ so:
\begin{gather*} 1=\frac{1}{2}(1.7)t^2+0 \\ \Rightarrow t=\sqrt{\frac{2}{1.7}}=1.1\quad {\rm s}\end{gather*}
A $150\,\mathrm{g}$ block is projected up a ramp with an initial speed of $7\, \mathrm{m/s}$. The coefficient of kinetic friction between the ramp and the block is $0.23$.
(a) If the ramp is inclined $25^\circ $ with the horizontal, how far along the surface of the ramp does the block slide before coming to a stop?
(b) The block then slides back down the ramp. What is the minimum coefficient static friction between the block and the ramp if the block is not slid back down the ramp?
(a) First, find the acceleration of the block then use $v^2-v^2_0=2a\mathrm{\Delta }x$ to get the distance traveled up the ramp.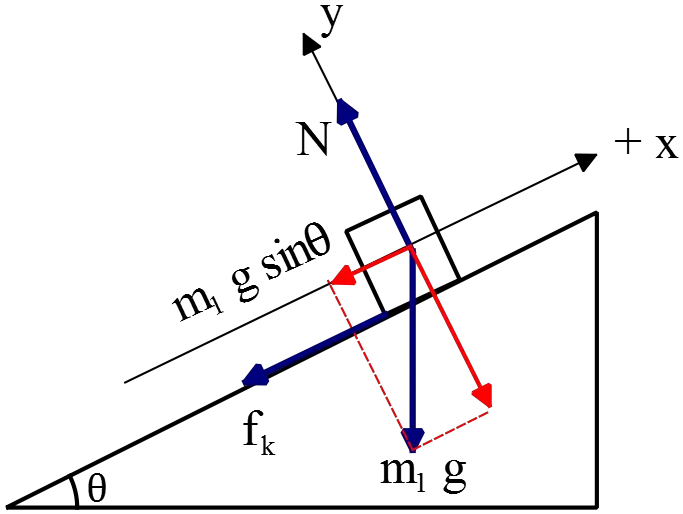
Draw a free body diagram as below:
Balance the normal forces on the ramp:
\[N-mg\,{\cos \theta}=0\Rightarrow N=mg\,{\cos \theta }\]
Apply Newton's second law along the ramp:
\[\mathrm{\Sigma }F_x=ma\to \ -mg\,{\sin \theta \ }-f_k=ma\]
Let the positive direction of motion toward the up of the ramp. (Because the forces along the ramp are in the opposite direction of the motion of the block so a negative sign is inserted!)
From the above conditions, we obtain:
\begin{gather*} N=mg\,\cos \theta \\ -mg\,\sin \theta -\mu_k N =ma \end{gather*} Thus, solving for $a$ in the above equations, gives \[ a=-g\,({\sin \theta}+\mu_k\,\cos \theta) \]
The negative indicates that the block is going to come to a stop!
\begin{align*} \Delta x&=\frac{v^2-v^2_0}{2a} \\\\ &=\frac{0-7^2}{-2(9.8)(\sin 25^\circ+0.23\,\cos 25^\circ)}\\\\& =4\quad{\rm N}\end{align*}
(b) Suppose the block does not slide back down, $a=0$ so forces on the block must balance.
\begin{gather*} N=mg\,\cos 25^\circ \\\\ mg\,\sin 25^\circ -f_{s,max}=0 \\\\ \to mg\,\sin 25^\circ -\mu_sN=0\end{gather*}
Substituting $N$ from first equation into the second, solve for $\mu_s$, we obtain \[\boxed{\mu_s=\tan 25^\circ=0.47} \]
Note: when the block reaches the highest point of the ramp, the parallel component of the weight ($mg\,{\sin \theta}$) and the friction force ($\mu_{k,s}N$) are in opposite directions. Since $mg\,{\sin \theta}$ is always downward of the ramp but friction forces oppose the relative direction of motion.
For more problems on the coefficient of friction, refer to:
A $10.0\,\rm kg$ block rests on a $5.0\,\rm kg$ bracket, as shown in the figure. The $5.0\,\rm kg$ bracket sits on a frictionless surface. The coefficients of friction between the $10.0\,\rm kg$ block and the bracket on which it rests are ${\mu }_s=0.40$ and ${\mu }_k=0.30$
(a) what is the maximum force $F$ that can be applied if the $10.0\,\rm kg$ block is not to slide on the bracket?
(b) What is the corresponding acceleration of the $5.0\,\rm kg$ bracket?
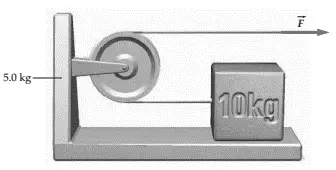
(a) If the block does not slide on the bracket, we can treat the block and bracket as one object. $F$ pulling them and gives the acceleration $F=\left(m_5+m_{10}\right)a$. Therefore, the block is accelerating to the right with $a=\frac{F}{m_5+m_{10}}$. Draw a free body diagram for the block and then apply Newton's second law to it.
\begin{gather*} \Sigma F_y=0\Rightarrow N-mg=0\\ \Rightarrow N=m_{10}g \\\\ \Sigma F_x=ma \Rightarrow f_{k,s}-T=m_{10}a\quad (*) \end{gather*}
The tension on the rope must be balanced with external force $F$, otherwise, the rope will break! So $F_{max}=T$.
Note: since we want to block and bracket moves together, so the positive direction of motion has been chosen to the right.
Note: because the$10\,{\rm kg}$ block does not move on the bracket so friction force is static $f_{k,s}=f_{s,max}={\mu }_sN$.
From ($*$), we have \begin{gather*}{\mu }_sN-F_{max}=m_{10}a\\\\ {\mu }_sm_{10}g-F_{max}=m_{10}\frac{F_{max}}{m_{10}+m_5}\\\\ \Rightarrow {\mu }_sm_{10}g=F_{max}\left(1+\frac{m_{10}}{m_{10}+m_5}\right) \end{gather*} Therefore, the maximum force is obtained as below \begin{align*} F_{max}&={\mu }_sm_{10}g\left(\frac{m_5+m_{10}}{m_5+2m_{10}}\right)\\\\&=(0.4)(9.8)\left(\frac{5+10}{5+2(10)}\right) \\\\ &=23.5\quad{\rm N}\end{align*}
(b) Since the maximum force $F$ applied to the system so both block and bracket have the same acceleration \[a_{max}=\frac{F_{max}}{m_5+m_{10}}=\frac{23.5}{5+10}=1.56\,{\rm m/s^2}\]
Practice these questions to understand friction force
Problems on coefficient of friction

A small object of mass $m_1$ moves in a circular path of radius $r$ on a frictionless horizontal tabletop. It is attached to a string that passes through a small frictionless hole in the center of the table. A second object with a mass $m_2$ is attached to the other end of the string. Derive an expression for $r$ in terms of $m_1$, $m_2$ and the time $T$ for one revolution.
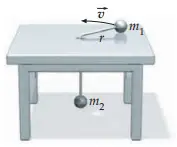
Mass $m_1$ is moving around a circle on the table, so it must have a centripetal acceleration. Since the only horizontal force on $m_1$ is the tension force $T$ on the string toward the center of the circle so applying Newton's second law in this radial direction gives us \begin{gather*} \Sigma F_{radial}=m_1a_r \\\\ \Rightarrow T=m_1\frac{v^2}{r} \\\\ \text{where} \quad v=r\omega=r\left(\frac{2\pi }{T}\right)\end{gather*} This same string is holding up $m_2$, so balance the vertical forces on $m_2$ (because $m_1$ moves around a circle with constant radius therefore $m_2$ does not move vertically) \begin{gather*} \Sigma F_{vertical}=0 \\\\ T-m_2g=0 \\\\ \Rightarrow \quad T=m_2g \end{gather*} From above results, one can write \begin{gather*} T=\frac{m_1}{r}{\left(\frac{2\pi r}{T}\right)}^2=m_2g \\\\ \Rightarrow r=\frac{m_2gT^2}{4{\pi }^2m_1} \end{gather*}
You are on an amusement park ride with your back against the wall of a spinning vertical cylinder. The floor falls away and you are held up by static friction. Assume your mass is $75\,\rm kg$.
(a) Draw a free-body diagram of yourself.
(b) Use this diagram with Newton's laws to determine the force of friction on you.
(c) If the radius of the cylinder is $4.0\, {\rm m}$ and the coefficient of static friction between you and the wall is $0.55$. What is the minimum number of revolutions per minute necessary to prevent you from sliding down the wall? Does this answer hold only for you? Will other more massive, patrons fall downward? Explain.
(a) The free-body diagram is shown below.

(b) Because there is no vertical motion, $\Sigma F_y=0\Rightarrow f_s-mg=0$. Therefore, the static friction force is \[f_s=mg=75\times 9.8=735\,\rm N\]
(c) If you are moving around a circle, you experience a centripetal force equals to $F_r=m\frac{v^2}{r}$ toward the center of the circle. In this problem, this acceleration is provided by normal force on the body, so $N=F_r=m\frac{v^2}{r}$ but the normal force ($N$) is related to the friction by $f_{s,max}={\mu }_sN$ therefore \begin{gather*} \mu_s N=mg \\\\ \to \ \mu_s \left(m\frac{v^2}{r}\right) =mg \\\\ \Rightarrow \quad v^2=\frac{rg}{\mu_s}\end{gather*} Recall that in the circular motions, tangential velocity is written as $v=r\omega =r\left(\frac{2\pi n}{T}\right)$ where $n$ is the total number of rounds.
Substituting $r\frac{2\pi n}{T}$ for $v$ in the above equation, we obtain: \begin{gather*} \left(r\frac{2\pi n}{T}\right)^2=\frac{rg}{\mu_s}\\\\ \Rightarrow \quad n^2=\frac{gT^2}{4{\pi }^2{\mu }_sr} \\\\ \Rightarrow n=\sqrt{\frac{9.8\times {60}^2}{4{\pi }^2\times 0.55\times 4}}=20.15\,\rm \frac{rev}{min}\end{gather*}
The system shown below consists of a $4.0\, \mathrm{kg}$ block resting on a frictionless horizontal ledge. This block is attached to a string that passes over a 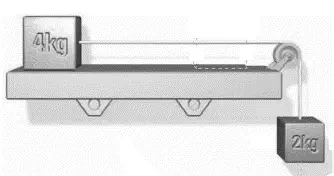 pulley, and the other end of the string is attached to a hanging $2.0\, \mathrm{kg}$ block. The pulley is a uniform disk of radius $8.0\, \mathrm{cm}$ and mass $0.6\,\mathrm{kg}$. Find the acceleration of each block and the tension in the two segments of the string.
pulley, and the other end of the string is attached to a hanging $2.0\, \mathrm{kg}$ block. The pulley is a uniform disk of radius $8.0\, \mathrm{cm}$ and mass $0.6\,\mathrm{kg}$. Find the acceleration of each block and the tension in the two segments of the string.
Note: let the positive direction of the motion as clockwise.
Draw a free body diagram for each block and the pulley then apply Newton's 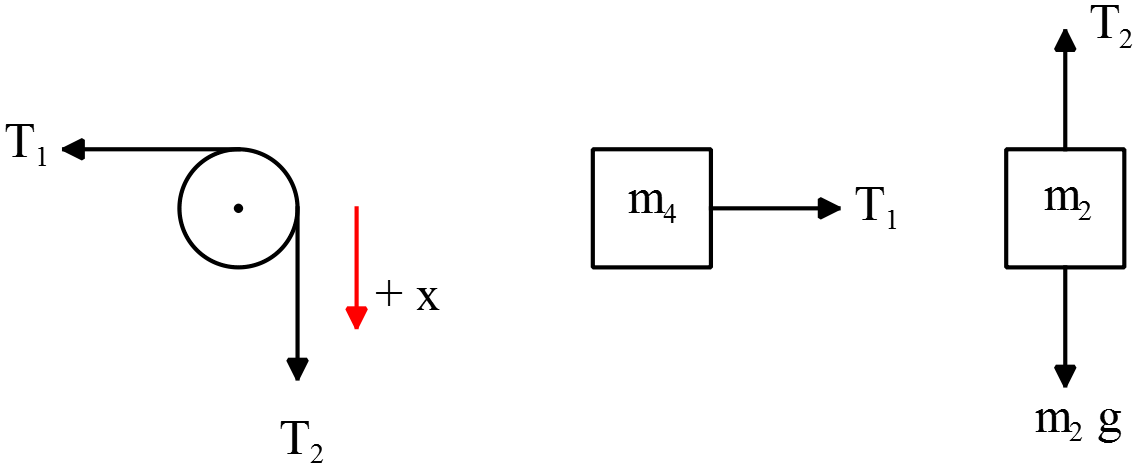 2${}^{nd}$ law to them.
2${}^{nd}$ law to them.
Note: due to the mass of the pulley, the tension on the rod is different along the two segments.
\[\mathrm{\Sigma }F=m_4a\Rightarrow T_1=m_4a\ \ ,\ \ (1)\]
\[\mathrm{\Sigma }F_y=m_2a\Rightarrow m_2g-T_2=m_2a\ \ ,\ \ (2)\]
$T_1$ and $T_2$ exert opposite torques on the pulley (Newton's 2${}^{nd}$ law for rotation) so
${\tau }_{net}=I\alpha $ where $\vec{\tau }=\vec{r}\times \vec{F}$ is net external torque on the pulley and $I$is the moment of inertia of the pulley so
\[\mathrm{\Sigma }\tau =I\alpha \mathrm{\Rightarrow }\left(T_2-T_1\right)R=I\alpha =I\frac{a}{R}\]
\[\Rightarrow T_2-T_1=\left(\frac{1}{2}m_pR^2\right)\frac{a}{R^2}\Rightarrow T_2-T_1=\frac{1}{2}m_pa\ \ ,\ \ (3)\]
(Recall that the tangential acceleration of a point on a rotating object relates to the angular acceleration via $a=R\alpha $ where $R$ is the radius of the rotation.)
\[\left(1\right),\left(2\right),\left(3\right)\Rightarrow m_2\left(g-a\right)-m_1a=\frac{1}{2}m_pa\ \]
Solving for $a$, we obtain
\[\therefore a=\frac{m_2}{\frac{1}{2}m_p+m_4+m_2}g=\frac{2}{\frac{1}{2}\left(0.6\right)+2+4}\left(9.8\right)=3.1\frac{\mathrm{m}}{{\mathrm{s}}^{\mathrm{2}}}\]
The cable supporting a $1500\,\mathrm{kg}$ elevator has a maximum strength of $21750\, \mathrm{N}$. What maximum upward acceleration can it give the elevator without breaking?
Apply Newton's second law in the upward direction, $\Sigma F_y=ma$, gives \[T-mg=ma\] Solving for $a$, gives \begin{align*} a&=\frac{T-mg}{m} \\\\ &=\frac{21750-(1500\times 9.8)}{1500}\\\\& =4.7\quad {\rm m/s^2}\end{align*}

An inverted "V'' is made of uniform boards and weights $356\,{\rm N}$. Each slide has the same length and makes a $30^\circ $ angle with vertical, as  shown below. Find the magnitude of the static frictional force that acts on the lower end of each leg of the ``V''.
shown below. Find the magnitude of the static frictional force that acts on the lower end of each leg of the ``V''.
Since the object is in equilibrium state, so apply the equilibrium conditions as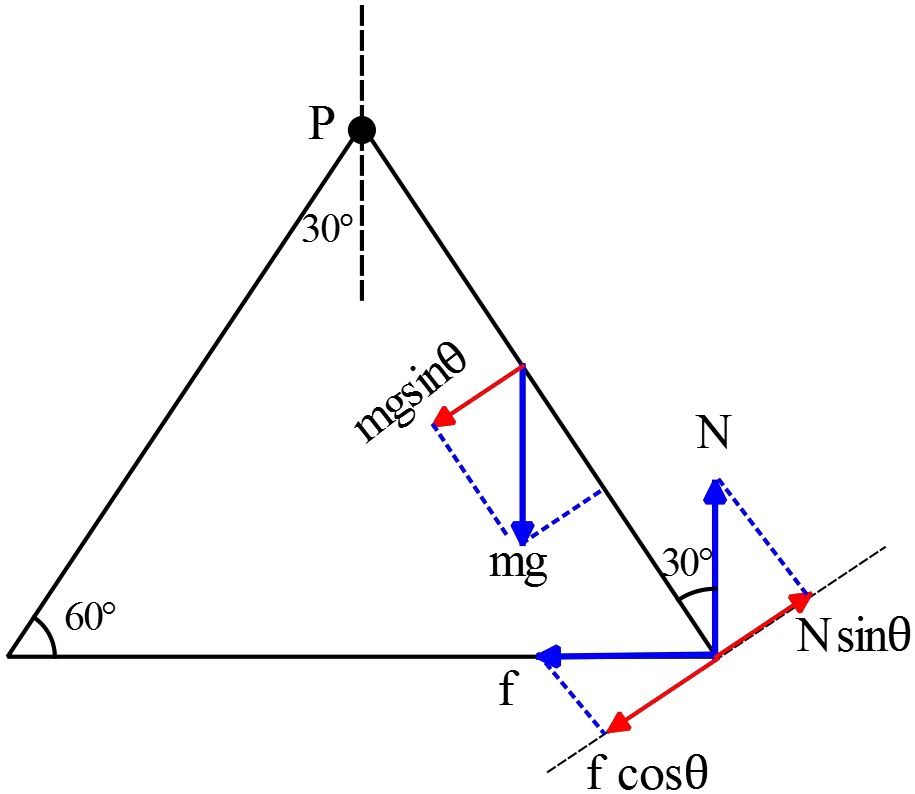
\[\mathrm{\Sigma }\vec F_x=0\ ,\ \ \mathrm{\Sigma }F_y=0\ ,\ \ \mathrm{\Sigma }\vec {\tau }_P=0\]
Now draw a diagram and show the forces acting on the object.
\[\mathrm{\Sigma }\vec F_y=0\Rightarrow N-mg=0\Rightarrow N=mg\]
The torque about a point is defined as $\vec{\tau }=\vec{r}\times \vec{F}$, in this problem choose an arbitrary point, say P, and set the torque about it equal zero to satisfy the equilibrium conditions. Let the positive direction be counter clockwise. Therefore,
\[\mathrm{\Sigma }\vec \tau =0\to {\tau }_{F_N}-{\tau }_{mg}-{\tau }_f=0\]
\[\mathrm{\Sigma }\vec {\tau }_P=0\Rightarrow l\underbrace{N}_{mg}{\sin \theta \ }-\left(\frac{l}{2}\right)mg{\sin \theta \ }-lf{\sin \left(\frac{\pi }{2}-\theta \right)\ }=0\]
\[mg\left(\frac{l}{2}\right){\sin \theta \ }=lf{\cos \theta \ }\Rightarrow f=\frac{1}{2}mg{\tan \theta \ }=\frac{1}{2}\left(\frac{356}{2}\right){\tan 30{}^\circ \ }=51.4\]
In above, we must insert the weight of one board.
Mass $m_1$ sits on a frictionless ramp at an angle of $\theta =30^\circ$. It is attached to mass $m_2$ via a rope winding over a pulley. Mass $m_2$ is 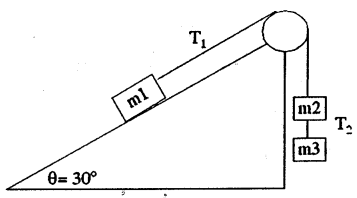 attached, via another rope, to mass $m_3$ as shown. The ropes and the pulley are massless, and the pulley is frictionless.
attached, via another rope, to mass $m_3$ as shown. The ropes and the pulley are massless, and the pulley is frictionless.
(a) Draw a free body diagram for each of the three masses.
(b) The masses are in equilibrium- when released, they do not move. Masses $m_2$ and $m_3$ are each $5.0\,\ \mathrm{kg}$. Find the tensions in the ropes, $T_1,T_2$ and the mass $m_1$.
(a)
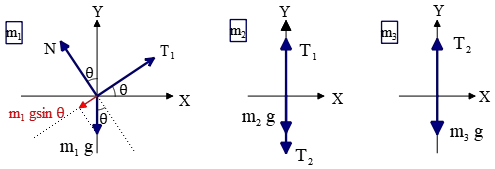
(b) Apply Newton's second law first on the $m_2$ and $m_3$ to find the tensions, then write again Newton's second law for mass $m_1$ along the ramp to find the unknown mass $m_1$.
\begin{gather*} \Sigma \vec F_3=0 \\ T_2-m_3g=0 \\ \Rightarrow T_2=m_3g=5\times 9.8=49\,{\rm N} \\\\ \Sigma \vec F_2=0 \\ T_1-T_2-m_2g=0\\ \Rightarrow T_1=49+5\times 9.8=98\, {\rm N} \\\\ \Sigma \vec F_1=0 \\ T_1-m_1g{\sin \theta}=0 \\ \Rightarrow m_1=\frac{T_1}{g\,\sin \theta}=\frac{98}{9.8\,\sin 30^\circ}=20\,{\rm kg}\end{gather*}
A box is sliding down an incline tilted at a $12.1^\circ$ angle above horizontal. The box is initially down the incline at a speed of $1.5\ \mathrm{m/s}$. The coefficient of kinetic friction between the box and the incline is $0.360$. How far does the box slide down the incline before coming to rest?
First, find the acceleration of the box with respect to the incline, then substitute it in the kinematic relation $v^2-v^2_0=2ax$ to determine the $x$.
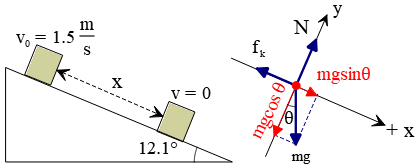
In above we have illustrated the problem and have drawn a free body diagram. Now apply the Newton's second law in the $x$ and $y$ directions i.e. $\Sigma F_y=0\, ,\, \Sigma F_x=ma$ \begin{gather*} \Sigma F_y=N-mg\,{\cos \theta}=0 \\ \Rightarrow\, N=mg\,\cos \theta \\\\ \Sigma F_x=mg\,{\sin \theta}-\underbrace{f_k}_{\mu_kN}=ma \\ \Rightarrow \, mg\,\sin \theta-\mu_k mg\,\cos\theta=ma\end{gather*}
From the second line, we get
\begin{align*} a&=g\,(\sin\theta-\mu_k\,\cos\theta) \\\\ &=(9.8)(\sin 12.1^\circ-0.360\,\cos 12.1^\circ) \\\\& =-1.395\quad {\rm m/s^2}\end{align*}
The minus sign indicates that the box is going to stop.
\begin{gather*} v^2-v^2_0=2ax \\ 0-(1.5)^2=2(-1.395)x \\ \Rightarrow x=0.806\,{\rm m=80.6\, cm}\end{gather*}
Engineers are designing a curved section of a highway. If the radius of the curvature of the curve is $146\, {\rm m}$, at what angle should the curve be banked so that a car travelling at $29\,{\rm m/s}$ will stay on the road without the aid of frictional forces?
First draw a free body diagram as below on the car
As shown in the figure, two forces acting on the car. The normal force and gravity. Since there is a circular motion, so we have a centripetal force that provided by one of the components of normal force that points to the center of the curved section. Apply Newton's second law as
\begin{gather*} \Sigma F_y=0 \Rightarrow N\cos \theta=W=mg \\\\ \Sigma F_r=ma_r\Rightarrow N\sin \theta=\frac{mv^2}{r} \end{gather*}
Dividing both equation by each other, we obtain the desired angle $\theta$
\begin{gather*} \frac{N\,\sin \theta}{N\,\cos \theta}=\frac{\frac{mv^2}{r}}{mg}\Rightarrow \tan \theta=\frac{v^2}{rg} \\\\ \Rightarrow \theta ={\tan}^{-1} \left(\frac{(29)^2}{146\times 9.8}\right)=30.44^\circ \end{gather*}
An oil droplet has a charge of $6.24\times {10}^{-18}\, \mathrm{C}$ and accelerates downward at as it drops by $1.66\ \mathrm{m/}{\mathrm{s}}^{\mathrm{2}}$ in a uniform electric field of strength $9.42\times {10}^3\, \mathrm{N/C}$ pointing upward.
(a) What is the mass of the oil droplet?
(b) How long would it take this oil drop to fall $4.24\ $$\mathrm{m}$ starting from rest?
(c) What would the E-field strength have to be for this drop to levitate (i.e. remain at constant height)?
(a) Two forces, electric and gravity, act on the droplet as shown in the free-body diagram below.

Recall that the force exerted by an uniform electric field $E$ on the charge $q$ is given by ${\vec{F}}_e=q\vec{E}$. Now apply Newton's 2${}^{nd}$ law to this droplet(let upward direction to be $+y$)
\begin{gather*} \Sigma \vec F_y=ma \\\\ F_e\hat{j}+mg(-\hat{j})=ma(-\hat{j}) \\\\ \Rightarrow mg-qE=ma \\\\ \Rightarrow \boxed{m=\frac{qE}{g-a}} \end{gather*} After substituting the numerical values, we have \begin{align*} m&=\frac{(6.24\times {10}^{-18})(9.42\times {10}^3)}{9.8-1.66} \\\\ &=7.22\times {10}^{-15}\, \rm kg \end{align*}
(b) Use the kinematic equation below to find the desired time \begin{gather*} y=\frac{1}{2}at^2+v_0t+y_0 \\\\ -4.24=\frac{1}{2}(-1.66)t^2 \\\\ \Rightarrow t=\sqrt{\frac{2\times 4.24}{1.66}}=2.66\,\rm s \end{gather*}
Note: we have chosen the reference point as the starting point so $y_0=0$ and since the droplet moving downward, so $y<0$. In the equation above, the acceleration $a$ is a vector quantity.
(c) To levitate the droplet, all forces acting on it must be in balanced so
\begin{gather*} \Sigma \vec F_y=0\Rightarrow F_e=mg \\\\ \Rightarrow qE=mg \Rightarrow \boxed{E=\frac{mg}{q}} \end{gather*} Substituting the numerical values, we will get \[E=\frac{(7.22\times {10}^{-15})(9.8)}{6.24\times {10}^{-18}}=1.13\times {10}^4 \,\rm N/C\]
Two buckets full of nails are connected by a light cord which passes over a light, frictionless pulley with a diameter of $50\ \mathrm{mm}$. Initially, the two 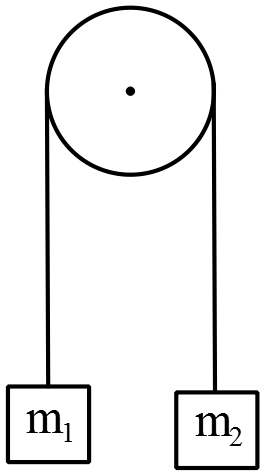 bodies are held at the same level. The buckets including the nails, have mass $m_1=500\ \mathrm{g}$ and $m_2=600\ \mathrm{g}$.
bodies are held at the same level. The buckets including the nails, have mass $m_1=500\ \mathrm{g}$ and $m_2=600\ \mathrm{g}$.
(a) The buckets are released from rest and at the same height at $t=0$. Calculate the tension of the string and the accelerations of the buckets after they are released.
(b) Where is the center of mass at the instant the buckets are released?
(c) Where is the center of mass after they are released? What is the acceleration of the center of mass?
(a) This configuration is called the Atwood's machine. We try to find its tension 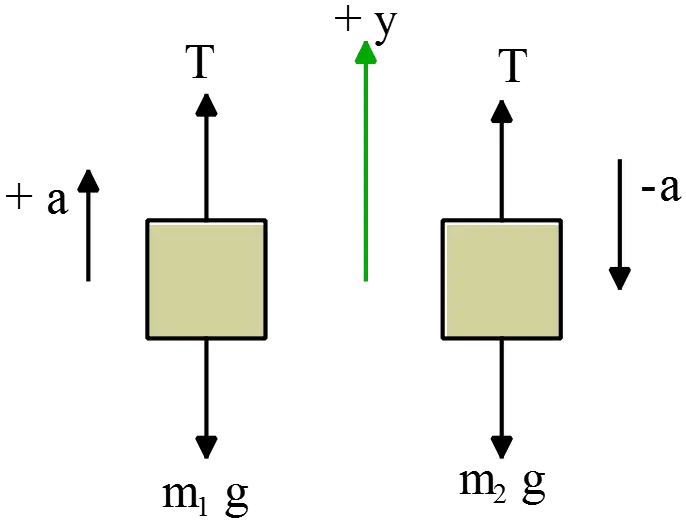 and acceleration. For this purpose, apply the 2${}^{nd}$ law of motion to each of the masses as
and acceleration. For this purpose, apply the 2${}^{nd}$ law of motion to each of the masses as
\[\mathrm{\Sigma }{\vec{F}}_{1y}=m_1\vec{a}\Rightarrow T\hat{j}+m_1g\left(-\hat{j}\right)=m_1a\hat{j}\]
\[\Rightarrow T=m_1\left(a+g\right)\]
\[\mathrm{\Sigma }{\vec{F}}_{2y}=m_2\vec{a}\Rightarrow T\hat{j}+m_2g\left(-\hat{j}\right)=m_2a\left(-\hat{j}\right)\]
\[\Rightarrow T=m_2\left(g-a\right)\]
Equal these two relations to find the acceleration of the system
\[m_1\left(a+g\right)=m_2\left(g-a\right)\Rightarrow a=\frac{m_2-m_1}{m_1+m_2}g\]
Now substitute $a$ into one of the $T$'s
\[T=m_2\left(g-a\right)=m_2\left(g-\frac{m_2-m_1}{m_1+m_2}g\right)=\frac{2m_1m_2}{m_1+m_2}g\]
Therefore, $a=\frac{0.6-0.5}{0.6+0.5}\left(9.8\right)=0.89\ \mathrm{m/}{\mathrm{s}}^{\mathrm{2}}$ and $T=5.3\ \ \mathrm{N}$
(b) By definition, the center of mass of a system of particles with masses $m_i$ is
\[M{\vec{r}}_{cm}=m_1{\vec{r}}_1+m_2{\vec{r}}_2+\dots \] 
Where $M$ is the total mass of the system and ${\vec{r}}_i$ is position vector of the $i$th particle. To find the CM of a system first establish a coordinate system. For this problem, draw a coordinate that passes through the center of the pulley, as shown:
Since at the instant of releasing the vertical components of each masses is zero, so $y_{CM}=0$. Now calculate the $x_{CM}$
\begin{align*} x_{CM}&=\frac{m_1x_1+m_2x_2}{m_1+m_2} \\\\ &=\frac{0.5(-25)+0.6(+25)}{0.5+0.6} \\\\ \Rightarrow \quad x_{CM} &=2.77\,\rm mm \end{align*} Therefore, the center of mass of this Atwood's machine is located at $(2.77\,\rm mm ,0)$.
(c) After releasing, because there is no external force on the machine (such as friction) so the horizontal component of the CM is constant and is the same as previous i.e. $x'_{CM}=2.77\,\rm mm$. To find the vertical component, use the kinematic equation $y=\frac{1}{2}at^2+v_0t+y_0$ as follows \begin{align*} y_{CM}&=\frac{m_1y_1+m_2y_2}{m_1+m_2}\\\\ &=\frac{m_1\left(\frac{1}{2}at^2\right)+m_2\left(\frac{1}{2}\left(-a\right)t^2\right)}{m_1+m_2} \\\\ &=\frac{m_1-m_2}{m_1+m_2}\left(\frac{1}{2}at^2\right) \\\\ &=-\frac{1}{2}\left(\frac{m_2-m_1}{m_1+m_2}\right)^2gt^2 \end{align*}
By comparison with $y_{CM}=\frac{1}{2}a_{CM}t^2$, we can obtain the acceleration of the CM as below \[a_{CM}=-\left(\frac{m_2-m_1}{m_1+m_2}\right)^2g\]
Put the given values of masses to determine the $y_{CM}$ and $a_{CM}$
\begin{gather*} y_{CM}=-\frac{1}{2}\left(\frac{0.6-0.5}{0.5+0.6}\right)^2(9.8)t^2=-0.04\,t^2 \\\\ \text{and} \quad a_{CM}=-0.081\,\rm m/s^2 \end{gather*} So the acceleration of the CM is downward.
Consider the pulley system as shown in the picture. The blocks have a mass of $\mathrm{1.0\ kg}$ each. Suppose the pulley is massless and there is no friction. What is the tension in the rope when the blocks accelerate due to gravity only?
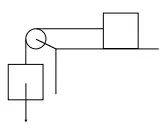
Apply Newton's 2${}^{nd}$ law to each of the masses. 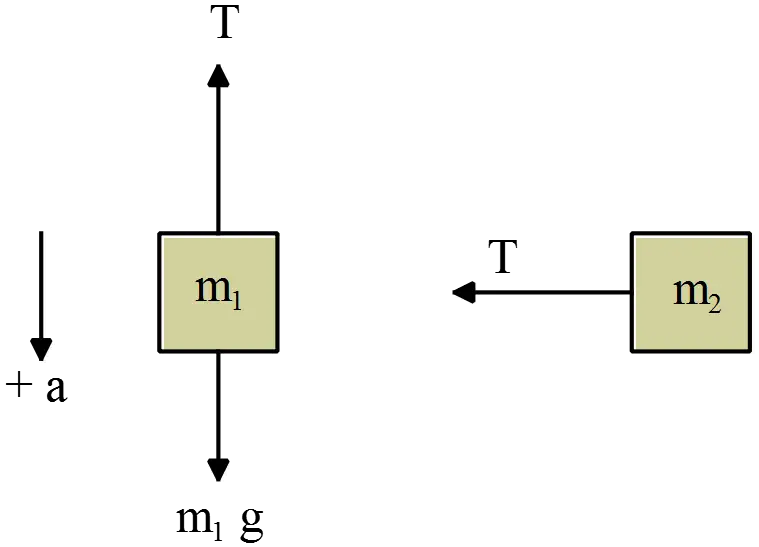
Mass $m_1$:
\[\mathrm{\Sigma }{\vec{F}}_y=m\vec{a}\to T\hat{j}+m_1g\left(-\hat{j}\right)=m_1a\left(-\hat{j}\right)\]
\[\Rightarrow T=m_1\left(g-a\right),\ \ *\]
Mass $m_2$:
\[\mathrm{\Sigma }{\vec{F}}_x=m\vec{a}\to T=m_2a\ ,\ \ \ \ \ \ \ **\]
Now set the tensions $T\ $equal to each other to find the $a\ $and then calculate the tension in the rope.
\[*\ ,\ **\ \ \Rightarrow \ m_1\left(g-a\right)=m_2a\Rightarrow a=\frac{m_1}{m_1+m_2}g\ \]
Now substitute $a$ into one of the tension relations above:
\[T=\frac{m_1m_2}{m_1+m_2}g=\frac{1\times 1}{1+1}\left(9.8\right)=4.9\frac{\mathrm{m}}{{\mathrm{s}}^{\mathrm{2}}}\]
In an amusement park ride, riders are pressed against a wall that revolves in a vertical circle of radius $r=5\, {\rm m}$ and angular velocity $\omega=1.60\,{\rm rad/s}$. Determine the force that the wall exerts on a $\mathrm{60.0\ kg}$ rider at the
(a) Top of the loop at point A.
(b) Bottom of the loop at point B.
(a) Since the riders are moving around a circle, so they experience a 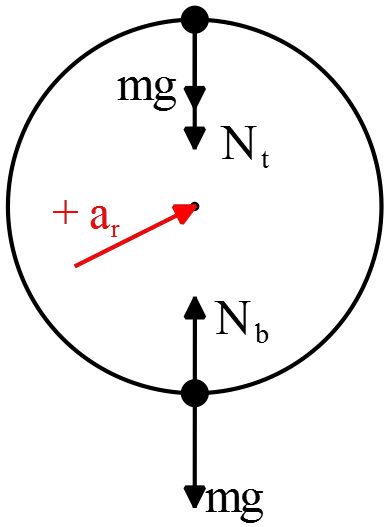 centripetal force. This force is always points toward the center of the circle i.e. $-\hat{r}$. The two forces, gravity and normal force, acting on the riders are provided the centripetal acceleration as $\mathrm{\Sigma }F_r=ma_r=mr{\omega }^2$.
centripetal force. This force is always points toward the center of the circle i.e. $-\hat{r}$. The two forces, gravity and normal force, acting on the riders are provided the centripetal acceleration as $\mathrm{\Sigma }F_r=ma_r=mr{\omega }^2$.
\[N\left(-\hat{r}\right)+mg\left(-\hat{r}\right)=mr{\omega }^2\left(-\hat{r}\right)\Rightarrow N=m\left(r{\omega }^2-g\right)\]
\[=60\left(5\times {\left(1.6\right)}^2-9.8\right)\]
\[\therefore \ N=180\ \mathrm{N}\]
(b) At the bottom of the loop, again, the normal force is points towards the center and instead gravity is away from the center. (as shown in the figure)
\[\mathrm{\Sigma }{\vec{F}}_r=m{\vec{a}}_r\to N\left(-\hat{r}\right)+mg\hat{r}=mr{\omega }^2\left(-\hat{r}\right)\Rightarrow N=m\left(g+r{\omega }^2\right)\]
\[\Rightarrow N=60\left(9.8+0.5\times {\left(1.6\right)}^2\right)=1360\ \mathrm{N}\]
Two blocks of mass $m_A=20\,\mathrm{kg}$ and $m_B=5\ \mathrm{kg}$ are 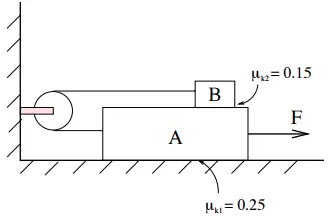 connected by a massless cable wound around a massless pulley. A force F is applied to block A causing it to slide over a horizontal surface with kinetic coefficient of friction $\mu_{k_1}=0.25$, while block B slides over block A with $\mu_{k_2}=0.15$.
connected by a massless cable wound around a massless pulley. A force F is applied to block A causing it to slide over a horizontal surface with kinetic coefficient of friction $\mu_{k_1}=0.25$, while block B slides over block A with $\mu_{k_2}=0.15$.
(a) What force is needed to move block A with constant speed?
(b) If the cable can sustain a maximum tension of $\mathrm{100\ N}$, what is the maximum force $F_{max}$ allowable without breaking the cable?
(a) As shown in the free body diagram of A, three forces act on the mass A. if  we suppose that at the instant the block A is moving, the block B is motionless, then the frictional force acting on A, due to the B is in the opposite direction of $F$. So
we suppose that at the instant the block A is moving, the block B is motionless, then the frictional force acting on A, due to the B is in the opposite direction of $F$. So
\[\mathrm{\Sigma }\vec F_A=0\ \left(constant\ speed\ ,a=0\right)\]
\[\Rightarrow F-f_{k_1}-f_{k_2}-T=0\Rightarrow F=f_{k_1}+f_{k_2}+T\]
Now apply Newton's 2${}^{nd}$ law to the mass B. Since we have supposed it is motionless so the tension in the rope is equal to the friction force between A and B.
\[\mathrm{\Sigma }\vec F_B=0\Rightarrow T-f_{k_1}=0\Rightarrow T=\mu_{k_2}m_{B}^{\vphantom{\ast}}g\]
Substituting $T$ and frictional forces in the relation of $F$, we obtain
\[F=\mu_{k_1}^{\vphantom{\ast}}\left(m_A^{\vphantom{\ast}}+m_B^{\vphantom{\ast}}\right)g+\mu_{k_2}^{\vphantom{\ast}}m_{B}^{\vphantom{\ast}}g+\mu_{k_2}^{\vphantom{\ast}}m_{B}^{\vphantom{\ast}}g\]
\[\Rightarrow F=\underbrace{\left(0.25\right)\left(5+20\right)\left(9.8\right)}_{f_{k_1}=61.25\ \mathrm{N}}+2\underbrace{\left(0.15\right)\left(5\right)\left(9.8\right)}_{f_{k_2}=7.35\ \mathrm{N}}=76\ \mathrm{N}\]
(b) In general case, the system has an acceleration. So in this case first find it and then calculate $F_{max}$. Repeat the steps above but here $\mathrm{\Sigma }F=ma$. Therefore
\[\mathrm{\Sigma }\vec F_B=m_B^{\vphantom{\ast}}a\Rightarrow T-f_{k_1}=m_B^{\vphantom{\ast}}a\Rightarrow T={\mu }_{k_2}m_B^{\vphantom{\ast}}g+m_B^{\vphantom{\ast}}a\]
It has been said the maximum tension in the string is $T_{max}=100\ \mathrm{N}$ so find the acceleration associated with this tension as
\[a_{max}=\frac{T_{max}-\mu_{k_2}^{\vphantom{\ast}}m_{B}^{\vphantom{\ast}}g}{m_B}=\frac{100-\left(0.15\right)\left(5\right)\left(9.8\right)}{5}=18.53\ \mathrm{m/}{\mathrm{s}}^{\mathrm{2}}\]
Now apply the 2${}^{nd}$ law to the mass A and substitute $a_{max}$ into it to find the $F_{max}$
\[\mathrm{\Sigma }F_A=m_Aa_{max}\Rightarrow \ F_{max}-f_{k_1}-f_{k_2}-T_{max}=m_Aa_{max\ }\]
\[\Rightarrow \ F_{max}=100+61.25+7.35+\left(20\right)\left(18.53\right)=539.2\ \mathrm{N}\]
A solid cylinder of mass $\mathrm{10\ kg}$ and radius $\mathrm{0.5\ m}$ is 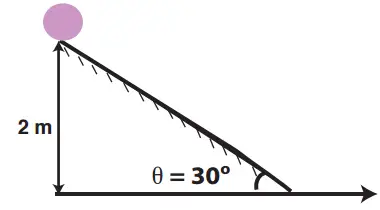 placed on the edge of a $30{}^\circ $ incline of height $\mathrm{2\ m}$. The coefficient of static friction of the incline's surface $\mu_s$ is just high enough such that the cylinder barely rolls without slipping down the incline.
placed on the edge of a $30{}^\circ $ incline of height $\mathrm{2\ m}$. The coefficient of static friction of the incline's surface $\mu_s$ is just high enough such that the cylinder barely rolls without slipping down the incline.
(a) What is $\mu_s$?
(b) How long does it take the cylinder to roll down to the bottom?
(c) What is the angular speed of the cylinder at the bottom of the incline?
(a) The friction force produces torque on the cylinder. Recall that the formula for the torque and moment of inertia are $\vec{\tau }=\vec{r}\times \vec{F}$ and $\tau =I\alpha$, respectively, where $\alpha$ is the angular acceleration that relates to that of linear via $a=\alpha r$
\begin{gather*} rF=I\alpha \Rightarrow rf_s=\frac{Ia}{r}\\\\ \to r(\mu_s mg\,{\cos 30^\circ})=\frac{\left(\frac{1}{2}mr^2\right)a}{r}\\\\ \Rightarrow \quad \mu_s=\frac{a}{2(g\cos 30^\circ)}\end{gather*}
Now apply Newton's second law to find the linear acceleration of the cylinder, then substitute it into the relation above.
\begin{gather*} \Sigma \vec F=m\vec a \\\\ \Rightarrow mg\,{\sin 30^\circ}-\underbrace{\mu_k mg\,{\cos 30^\circ}}_{f_s}=ma\\\\ \Rightarrow a=g({\sin 30^\circ}-\mu_s{\cos 30^\circ}) \end{gather*} Therefore, the coefficient of static friction gets as \begin{align*} \mu_s&=\frac{g\,({\sin 30^\circ}-{\mu }_s\,{\cos 30^\circ})}{2(g\,{\cos 30^\circ})}\\\\ &=\frac{1}{2}\left({\tan 30^\circ}-{\mu }_s\right) \\\\ \Rightarrow \mu_s&=\frac{1}{3}{\tan 30^\circ}\\\\& =0.192 \end{align*}
(b) With the given $a$, use the kinematic relation $y=\frac{1}{2}at^2+v_0t+y_0$ to find $t$. ($\Delta y=y-y_0$ is the displacement on the incline that is equal to $\Delta y=\frac{2}{\sin 30^\circ}=4\,{\rm m}$)
\begin{align*} a&=g\left({\sin 30^\circ}-{\mu }_k{\cos 30^\circ}\right)\\ &=(9.8)\left(0.5-(0.192){\cos 30^\circ}\right)\\ &=3.27\quad {\rm m/s^2} \end{align*} Hence, the displacement is \begin{align*} \Delta y&=\frac{1}{2}at^2+0\\\\ \Rightarrow \quad t&=\sqrt{2\frac{\Delta y}{a}}\\\\ &=\sqrt{\frac{2(4)}{3.27}}\\\\ &=1.56\quad {\rm s}\end{align*}
(c) In rotational motions, the angular speed is related to the angular acceleration by \begin{align*} \omega=\alpha t&=\left(\frac{a}{r}\right)t\\\\ &=\left(\frac{3.27}{0.5}\right)(1.56) \\\\ &=10.24\quad {\rm \frac{rad}{s}}\end{align*}
To practice more problems on coefficient of friction, refer to:
Problems on coefficient of friction force
Mr. Bean visits a park one afternoon. He spots a swing, pulls it back to an angle of $55{}^\circ $ w.r.t the vertical, and swings himself from rest. The combined mass of Mr. Bean and the swing is $65\ \mathrm{kg}$. The rope is approximately massless.
(a) Assuming that the rope does not break, at what angle $\theta $ is there maximum tension in the rope?
(b) If the maximum tension that the rope can withstand is $980\ \mathrm{N}$, find the angle at which the rope breaks and spoils Mr. Bean's fun?
(a) Draw a free body diagram in the general case as shown. Apply 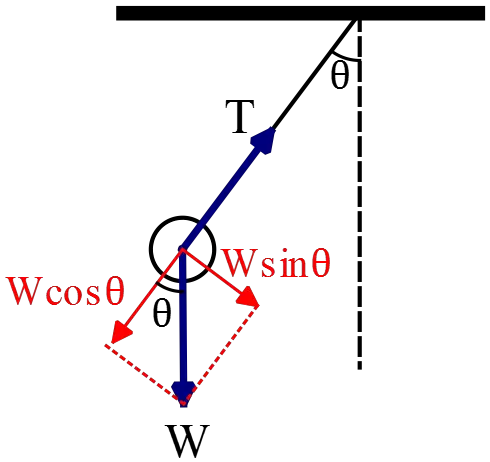 Newton's 2${}^{nd}$ law in central and tangential directions to rope.
Newton's 2${}^{nd}$ law in central and tangential directions to rope.
\[\mathrm{\Sigma }\vec{F}=m\vec{a}\]
\[\to \ \left\{ \begin{array}{rcl}
T\left(-\hat{r}\right)+W\,{\cos \theta \ }\left(\hat{r}\right) & = & ma_r\left(-\hat{r}\right)\Rightarrow T =W{\cos \theta \ }+ma_r \\
W\,{\sin \theta \ } & = & ma_x \end{array}
\right.\]
Since ${\cos \theta \ }$ in the interval $[0,\pi /2]$ decreases, so if we set $\theta =0{}^\circ $ one can get the maximum value of the tension i.e. $T_{max}=W+ma_r$.
(b) Now use the conservation of energy between $55{}^\circ $ and the angle $\theta $ at which the tension reaches its maximum and breaks. (we have 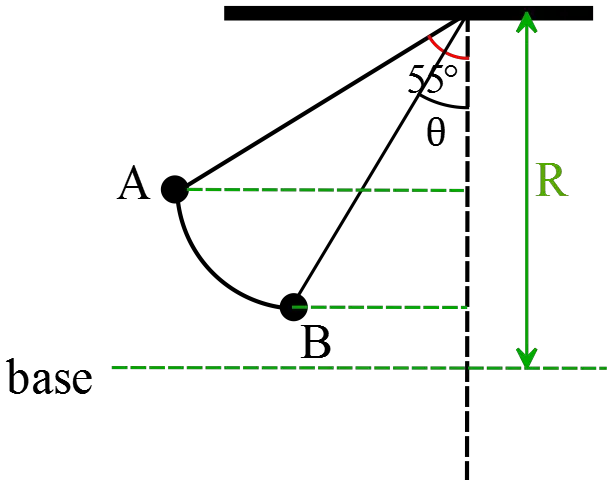 chosen the lowest point of the swing as the base)
chosen the lowest point of the swing as the base)
\[E_A=E_B\to \ \ K_A+U_A=K_B+U_B\]
\[\Rightarrow 0+mgR\left(1-{\cos 55{}^\circ \ }\right)=\frac{1}{2}mv^2+mgR\left(1-{\cos \theta \ }\right)\]
\[\Rightarrow \frac{mv^2}{R}=2mg({\cos \theta \ }-{\cos 55{}^\circ \ })\]
In part (a) we found that at any point the tension in the rope is
\[T=mg{\cos \theta \ }+\frac{mv^2}{R}\]
Where we have used the centripetal acceleration i.e. $a_r=v^2/R$. Combining the two relations give the tension of the rope as $T=mg(3\,{\cos \theta \ }-2\,{\cos 55{}^\circ \ })$. Set $T_{max}=980\ \mathrm{N}$ to find the desired angle $\theta \ $.
\[980=\left(65\times 9.8\right)\left(3\,{\cos \theta \ }-2\,{\cos 55{}^\circ \ }\right)\Rightarrow \theta =26.46{}^\circ \]
A uniform beam, $\mathrm{5.00\ m}$ long with a mass of $\mathrm{2.00\times }{\mathrm{10}}^{\mathrm{3}}\mathrm{\ kg}$, is held against a wall in the position 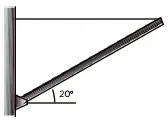 shown by a hinge and a horizontal steel wire attached to its end
shown by a hinge and a horizontal steel wire attached to its end
(a) Find the tension in the wire.
(b) Find the magnitude of the total force supplied by the hinge.
(a) Since the system does not have any acceleration so is in a static situation. 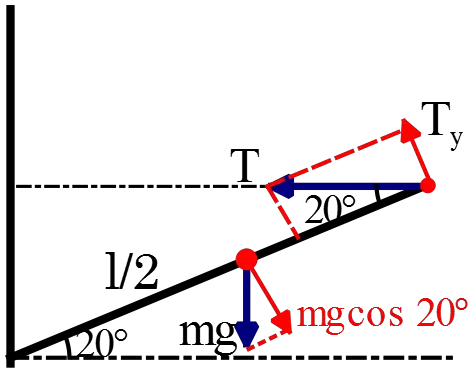 Calculate the torque acting on the system about the hinge point and set it zero.
Calculate the torque acting on the system about the hinge point and set it zero.
\[\vec{\tau }=\vec{r}\times \vec{F}\Rightarrow \ \tau =mg\left(\frac{l}{2}\right){\cos 20{}^\circ \ }-Tl\,{\sin 20{}^\circ \ }=0\]
\[\Rightarrow T=\frac{mg}{2}{\cot 20{}^\circ \ }=26.925\ \mathrm{kN}\]
(b) The total force supplied by the hinge is the normal force that lies in the direction of the rod. if we decompose it relative t the $x$ and $y$ axes and 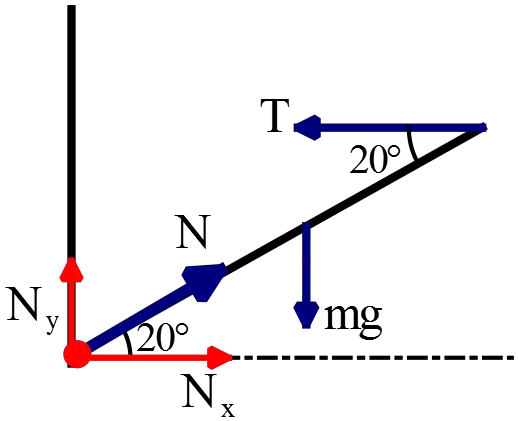 apply the $\mathrm{\Sigma }\vec{F}=m\vec{a}$ to the center of mass of the rod (CM is motionless $s=0$), we get
apply the $\mathrm{\Sigma }\vec{F}=m\vec{a}$ to the center of mass of the rod (CM is motionless $s=0$), we get
\[\mathrm{\Sigma }\vec F_x=ma_x=0\Rightarrow N_x=T=26.925\ \mathrm{kN}\]
\[\mathrm{\Sigma }\vec F_y=ma_y=0\Rightarrow N_y=mg=2000\times 9.8=19.6\ \mathrm{kN}\]
\[\left|N\right|=\sqrt{N^2_x+N^2_y}=\sqrt{{\left(26925\right)}^2+{\left(19600\right)}^2}=33.303\ \mathrm{kN}\]
A hockey puck slides frictionlessly in a horizontal circle on the inside surface of an inverted cone as shown in cross section at right. It takes $2$ seconds to complete one pass around its circular path.
(a) Identify all forces on the puck, draw a clear and complete free body diagram, and obtain the two equations that result from applying Newton's Second law to the puck.
(b) What is the radius of the circular path?
(a) The two forces, the gravity and the normal force, act on the car as shown 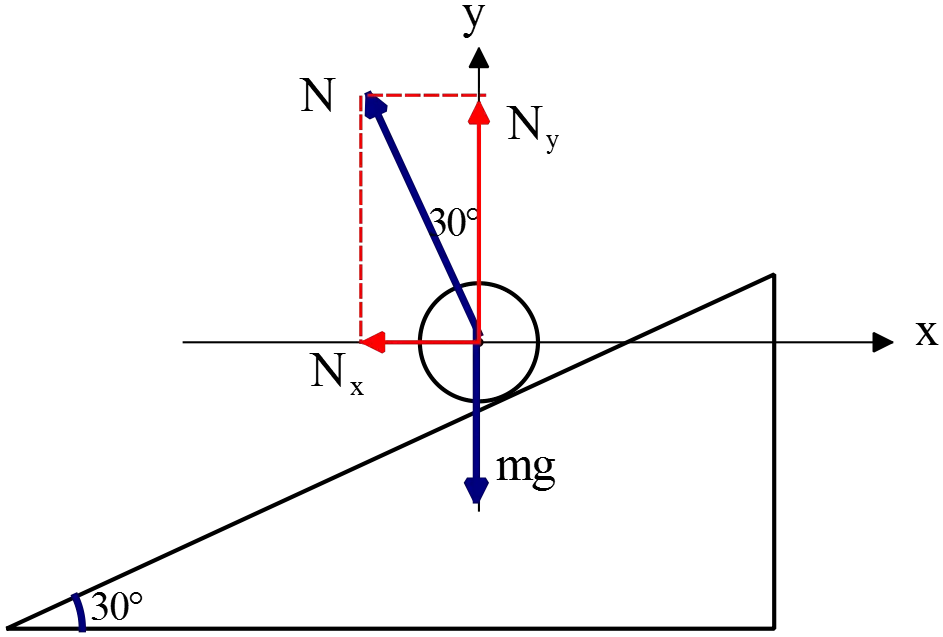 in the figure. The horizontal component of the normal force provides the centripetal force in the circular path. Apply Newton's 2${}^{nd}$ law to the car as follows
in the figure. The horizontal component of the normal force provides the centripetal force in the circular path. Apply Newton's 2${}^{nd}$ law to the car as follows
\[\mathrm{\Sigma }\vec F_x=mr{\omega }^2\Rightarrow N{\sin 30{}^\circ \ }=mr{\left(\frac{2\pi }{T}\right)}^2\]
\[\mathrm{\Sigma }\vec F_y=ma_y=0\Rightarrow N{\cos 30{}^\circ \ }=mg\]
Dividing these equations eliminates $N$ and allows us to find $r$:
\[{\tan 30{}^\circ \ }=\frac{4{\pi }^2r}{T^2g}\Rightarrow r=\frac{gT^2{\tan 30{}^\circ \ }}{4{\pi }^2}=\frac{\left(9.8\right){\left(2\right)}^2}{4{\pi }^2}\,{\tan 30{}^\circ \ }=0.573\ \mathrm{m}\]
(b) See part (a).
A block of mass $M$ hangs in equilibrium. The rope which is fastened to the wall is horizontal and has a tension of $40\, \rm{N}$. The rope, which is fastened to the ceiling, has a tension of $50\,\rm N$ and makes an angle $\theta $ with the ceiling. Find $\theta $ and mass of the block $M$.
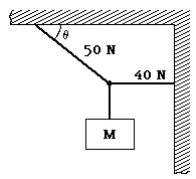
This is a static problem since there is no motion ($v=0\ ,\ a=0$). First draw a free body diagram then apply Newton's second law to the node in the figure as follows: \begin{gather*} \Sigma \vec F_x=0 \\ T_1-T_2{\cos \theta}=0 \\ \Rightarrow {\cos \theta}=\frac{T_1}{T_2} \end{gather*} Substituting the numerical value into this gives \begin{align*} \theta &={{\cos}^{-1} \left(\frac{T_1}{T_2}\right)} \\\\ &={{\cos}^{-1} \left(\frac{40}{50}\right)} \\\\ &=37^\circ \end{align*} Now write Newton's second law in the $y$ direction and find $M$ \begin{gather*} \Sigma \vec F_y=0 \\ T_2{\sin \theta}=Mg \end{gather*} Solving for $M$ and substituting the values into it yields \begin{align*} M&=\frac{T_2}{g}{\sin 37^\circ} \\\\ &=\frac{50}{9.8}(0.6) \\\\ &=3.06\,\rm kg \end{align*} In the above, we have used the well-known formula in trigonometry to find the ${\sin 37^\circ}$ \begin{gather*} {{\sin}^2 \theta}+{{\cos}^2 \theta}=1 \\\\ \Rightarrow {\sin \theta}=\sqrt{1-{{\cos}^2 \theta}} \\\\ =\sqrt{1-{(0.8)}^2}=0.6 \end{gather*}

A system comprising blocks, a light frictionless pulley, and connecting ropes is shown.
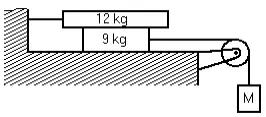
The $9\, \rm{kg}$ block is on a smooth horizontal table ($\mu =0$). The surface of the $12\, \rm{kg}$ block are rough, with $\mu =0.3$.
(a) The mass $M$ is set so that it descends at constant velocity when released. What is the mass $M$?
(b) If mass $M$ is set at $5\, \rm{kg}$ it accelerates downward when it is released. Determine its acceleration.
(a) Since $M$ is moving at a constant velocity so the acceleration of the system is zero.
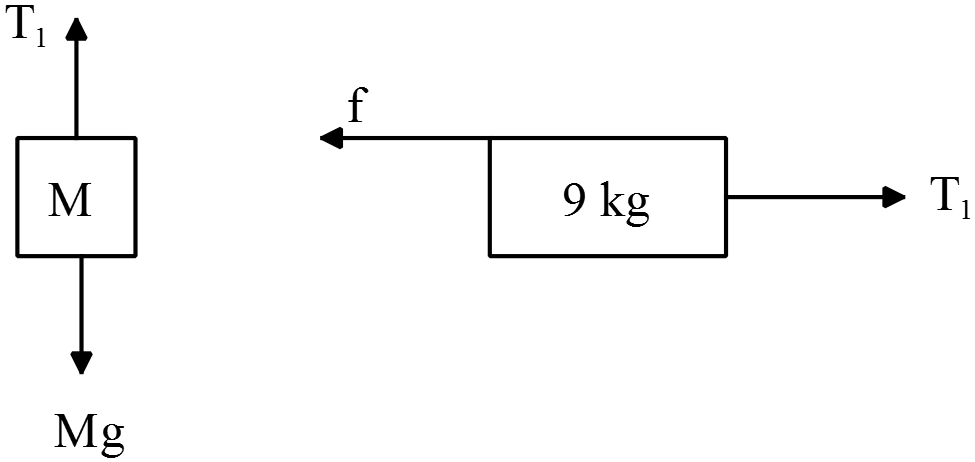
Apply Newton's 2${}^{nd}$ law to each of the blocks as follows
\begin{gather*} \Sigma \vec F_y=0 \\ Mg-T_1=0 \\ \Rightarrow M=\frac{T_1}{g} \\\\ \Sigma \vec F_x=0 \\ T_1-f=0 \\ \Rightarrow T_1=\mu m_{12} g \end{gather*}
Combining these two relations gives \[M=\mu m_{12}=0.3\times 12=3.6\,\rm kg\]
(b) In this case it is assumed the system has acceleration, so apply Newton's second law to the masses $M$ and $9\ \mathrm{kg}$ (see figures)
\begin{gather*} \Sigma \vec F_y=Ma \\ Mg-T_1=Ma \\ \Rightarrow T_1=M(g-a) \\\\ \Sigma \vec F_x=m_9 a \\ T_1-f=m_9 a \\ \Rightarrow T_1=m_9 a+\mu m_{12}g \end{gather*} Combining these two equations gives
\begin{align*} M(g-a)&=m_9\,a+\mu m_{12} g \\\\ \Rightarrow a&=\frac{(M-\mu m_{12})}{m_9+M}g \\\\ &=\frac{5-0.3\times 12}{9+5}(9.8) \\\\ &=0.98\,\rm m/s^2 \end{align*}
A $2\,\rm kg$ block is pulled up a plane inclined at an angle of $30^\circ $ by a massless string that passes over an ideal pulley and is attached to a $3\,\rm kg$ mass that accelerates downward at $2\,\rm m/s^2$ a shown.
(a) What is the tension in the string?
(b) What is the coefficient of kinetic friction between the block and the plane?
(c) What would be the acceleration if the friction were reduced to zero?
(a) Draw a free body diagram for the masses then apply the second law to them.
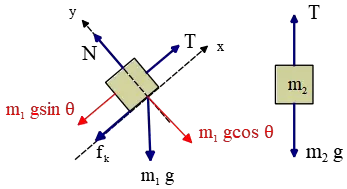
\begin{gather*} \Sigma \vec F_{1y}=0\to N=m_1g\,{\cos \theta \ } \\\\ \Sigma \vec F_{1x}=m_1a \\ \to T-m_1g\,{\sin \theta}-\underbrace{f_k}_{{\mu }_kN}=m_1a \\\\ \Sigma \vec F_{2y}=m_2a \to m_2g-T=m_2a\end{gather*}
In above, let the direction of the motion as the positive direction so we have supposed that the system moves in the clockwise. Combining these relations, we get the
\begin{gather*} T= m_1\left(g{\sin \theta}+\mu_kg\,{\cos \theta}+a\right) \\ T=m_2(g-a) \end{gather*} Substitute the information given into the second equation; we will obtain the tension in the string as \[T=m_2(g-a)=3(9.8-2)=23.4\,\rm N\]
(b) This part is a question about coefficient of kinetic friction. Using the above equations, we can obtain the coefficient of kinetic friction $\mu_k$ \begin{gather*} m_1(g \sin \theta+\mu_k g\,\cos \theta+a)=m_2(g-a) \\\\ \mu_k=\frac{m_2 (g-a)-m_1 (g \sin\theta +a)}{m_1 g \cos\theta}=0.566\end{gather*}
(c) If we set $\mu_k=0$ in the curved bracket in the part (a), and solve for $a$, we will obtain \[a=\frac{(m_2-m_1\sin\theta)g}{m_1+m_1}\] By substituting the numerical values, we get \[a=\frac{3-2\sin 30^\circ}{3+2}\times 9.8=3.92\,\rm m/s^2\]
Two blocks connected by a massless string slide across a horizontal floor with a coefficient of kinetic friction $\mu =0.4$ in response to a $30\ \mathrm{N}$ force as shown.
(a) What is the acceleration of the blocks?
(b) What is the tension $T$ in the string connecting the blocks?
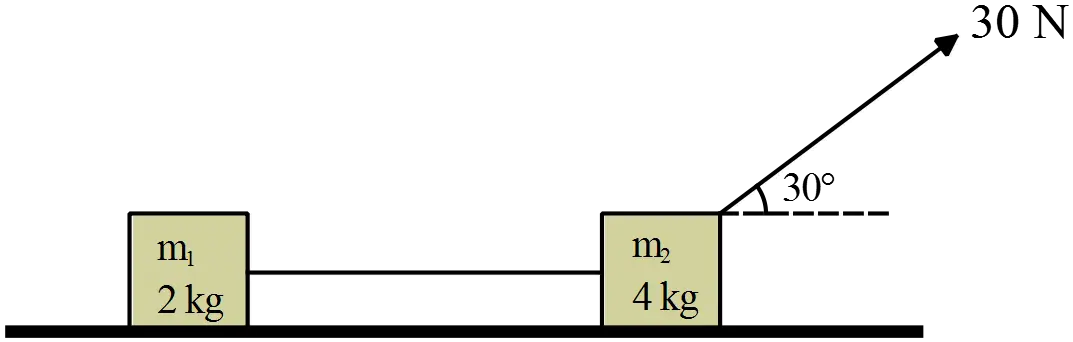
(a) Apply Newton's second law to the blocks as
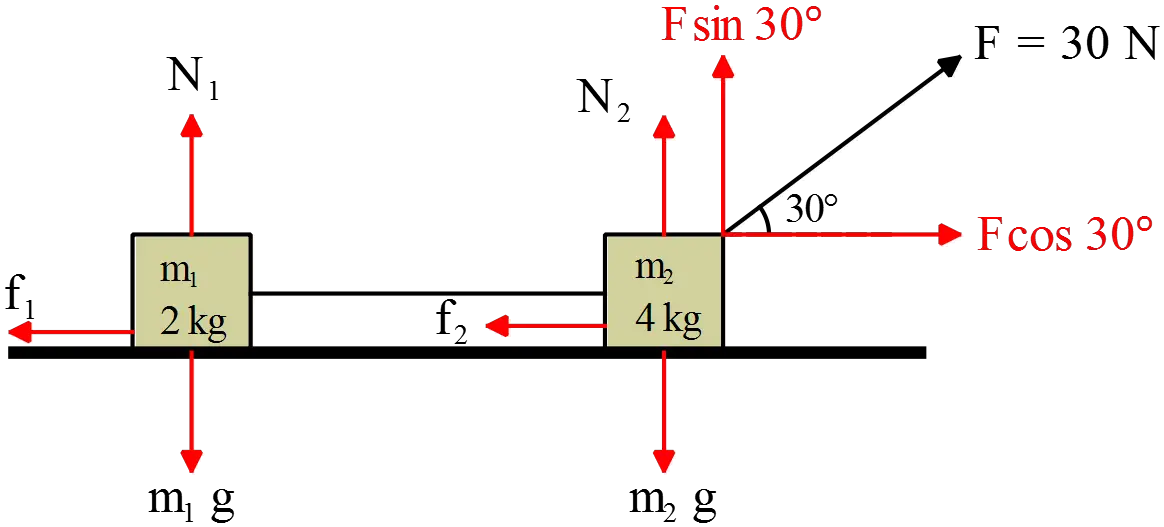
\begin{gather*} \Sigma \vec F_x=M_{tot}a \\ \to \, F{\cos 30^\circ}-f_2-f_1=(m_1+m_2)a \\\\ \Sigma\vec F_{2y}=0 \\ \to N_2+F\,{\sin 30^\circ }=m_2g \\\\ \Sigma \vec F_{1y}=0 \\ \to N_1=m_1g \end{gather*} We know that $f=\mu N$ gives the friction force so
\[F{\cos 30^\circ}-\mu (m_2g-F{\sin 30^\circ})-\mu m_1g=(m_1+m_2)a\]
Substituting the data given into the above expression, we obtain
\[\boxed{a=1.41\,\rm m/s^2}\]
Note: in the second law, all forces must be external forces. In the above, the tension on the string between the blocks is an internal force and does not contribute to calculating the total acceleration of the blocks.
(b) Now apply Newton's second law to one of the blocks, say $m_1$, as follows
\begin{gather*} \Sigma \vec F_{1x}=m_1a \\ \to T-\underbrace{\mu N_1}_{f_1}=m_1a \end{gather*}
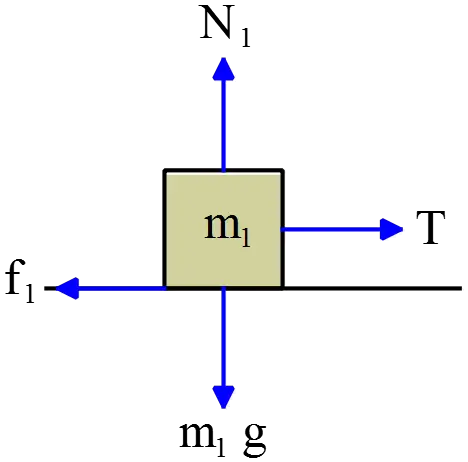
Substituting the given date, we get
\begin{gather*} T=\mu m_1g+m_1a=m_1(\mu g+a) \\\\ \Rightarrow T=2(0.4\times 9.8+1.41)=10.66\,\rm N\end{gather*}
MOST USEFUL FORMULA: NEWTON'S LAWS OF MOTION:
Force is a vector quantity so the resultant force of $F_1,F_2,\dotsc$ is
\[\vec F_{net}=F_1+F_2+\dotsc\]
Equilibrium conditions:
\[\Sigma \vec F=0 \quad , \quad \Sigma \vec \tau=0\]
Newton's second law of motion formula:
\[\Sigma \vec F=m\vec a\]
Definition of the weight of a body of mass $m$:
\[w=mg\]
Newton's third law of motion formula:
\[\vec F_{A\ on \ B}=-\vec F_{B\ on \ A}\]
Magnitude of the kinetic friction force:
\[f_k=\mu_k N\]
where $N$ is the normal force perpendicular to the surface of contact and $\mu_k$ is the coefficient of kinetic friction.
Magnitude of static friction:
\[f_{s,max}=\mu_s N\]
Acceleration in a uniform circular motion:
\[a_{rad}=\frac {v^{2}}{r}\]
© 2015 All rights reserved. by Physexams.com
