Work and Energy : Definition and Solved Examples
In physics, "work" is defined as the energy transferred to or from an object via the application of force along a displacement. The everyday meaning of work is not similar to its physics meaning.
For instance, if you hold a heavy suitcase for a long time, you get tired because the muscles in your arm do work as they are in a contracting position. This work is physiological and internal work.
Even when you walk with constant velocity across the horizontal floor while carrying the suitcase, you still do not work on it. The mechanical work is done when the force and displacement have components along each other, say, the suit is carrying up the stairs
The physical concept of work
Consider a point object (we want to ignore the rotation and other mechanical properties of solid objects) undergoes a displacement $\vec x$ along a straight line by applying a constant force $\vec F$ on it. The work done by this force on the object is defined as the dot product of the force and the displacement vector. In mathematical language is
\[W=\vec F.\vec x=|\vec F||\vec x| \cos \theta\]
Where $\theta$ is the angle between the force and the distance traveled. In the above, the distance traveled is the component of motion parallel to the direction of the force. Forces that don’t act to slow down or speed up an object do not work. For example, the forces $N$ and gravity $mg$ do not cause the change in the velocity of the block in the direction of its acceleration, which is shown by displacement $\vec S$, so their work is zero. On the other hand, since the angles between these forces and displacement are $\pm 90\,^\circ$, the work done on the object due to these forces is zero.
If the applied force is not constant and varies with position such as a stretched spring, we must use the integral form of the work as \[W=\int_{x_i}^{x_f} F_x\, dx\]
Where $F_x$ is the component of the force along the distance traveled. In the case that the graph of force versus distance given the work done is the area under the curve.
Work is a scalar quantity and the SI unit of work is Joule which can be expressed as
\[ work\, =\, force\ \times distance\]
\[=N.m=\left({\rm \frac{kg.m}{s^2}}\right).{\rm m}={\rm J}\]
Therefore, work is a form of energy.
If the force and displacement vectors are in components, say, $\vec F=F_x\, \hat{i}+F_y\,\hat{j}+F_z\,\hat{k} $ and $\vec S=\Delta x\, \hat{i}+\Delta y\,\hat{j}+\Delta z\,\hat{k}$, the work can be calculated by the rules of dot product as
\[W=\vec F.\vec S=F_x\Delta x+F_y\Delta y+F_z\Delta z\]
In summary, the work done on a point particle depends only on the force, displacement and the angle between them and is independent of the velocity and acceleration of the object.
Positive and negative work
As discussed the physics seems to contradict the common sense of work that is when the force acts on a body has a component in the same direction of the displacement (the angle between them $\theta$ to be zero through $90\,^\circ$), the work $W$ is positive since $\cos \theta>0$. When the force has a component opposite to the displacement (the angle $90\,^\circ<\theta<180\,^\circ$), the work $W$ is negative such as the work of friction and dissipative forces. In the figure below, the constant force $\vec F$ does positive, negative and zero work on the block.
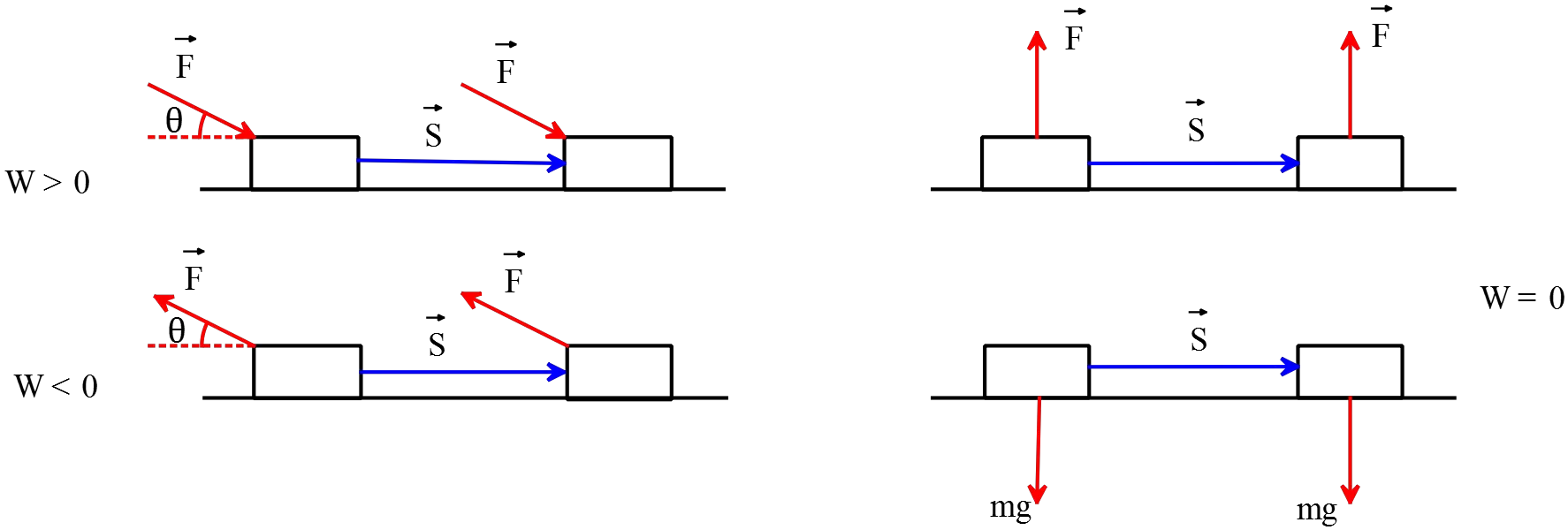
Total work
There are two ways to calculate the total work done by several forces. One way is to find the work done by each of the forces and then algebraic sum of these works gives the total work done by each of them. The other is to find the net force (vector sum of each of these forces) acts on the object and then use it in the definition of the work as \[W_{net}=\left(\Sigma \vec F\right).\vec S\]
When a total work is done on a body, the body’s position changes and subsequently its velocity can change. Let’s make this statement some quantitative. Consider a particle of mass $m$ under a constant net force $\vec F$ accelerate from initial speed $v_i$ to a speed $v_f$ over a distance $x$ along the direction of the force. Using Newton’s second law of motion and constant acceleration kinematic equation we have
\[\Sigma \vec F=m\vec a\ \to F=ma\]
\[v_f^2-v_i^2=2ax\ \to a=\frac{v_f^2-v_i^2}{2x}\]
Substituting this into the second law, we get
\[F=m\frac{v_f^2-v_i^2}{2x} \Rightarrow Fx=\frac{1}{2}m\left(v_f^2-v_i^2\right)\]
We know that the product of the force and displacement is the total work done on the body, but in this relation the new quantity which is appeared called kinetic energy $K$ of the body:
\[K=\frac{1}{2}mv^2\]
Like work, the kinetic energy of a particle is a scalar quantity and depends only on the particle’s mass and speed not its direction of the motion or velocity. Kinetic energy is always positive and when the object is at rest its value is zero. It is a measure of energy associated with a moving object. In order to change the kinetic energy of an object, one must spend some energy, say, one must do work. The equation above can be rearranged as follows
\[W_{net}=\Delta K\]
This is a general principle and has a special name: the work-kinetic energy theorem.
When one or more forces act on a particle and cause the particle displaced from initial position to a final position, the net work done on the particle by these forces can change the kinetic energy of it by $\Delta K=W_{net}$.
Power
By definition, work is a transfer of energy between the environment and a system. In general, we would like to know how quickly the energy is transferred or the work is done. We describe this in terms of power. Power is defined as the time rate of transfer of energy or of doing work. For example, we can do a work of $100\,{\rm J}$ to push a block of $100\,{\rm N}$ one meter across the floor but this work could have been done $1$ second, $1$ hour or $1$ year. Therefore, when a work $\Delta W$ done during a time interval $\Delta t$, the average work done per unit time or average power is defined as
\[P_{av}=\frac{\Delta W}{\Delta t}\]
The SI unit of power is the watt, which is defined as $1\, {\rm watt=1\, W=1\, \frac{J}{s}}$. the English unit of power is the horsepower which is defined as
\[\rm{1\ hp=746\ W}\]
Another important unit that is used in the electrical industry is kilowatt-hour ($\rm {kW.h}$). one kilowatt-hour is the total work done in an hour when the power is $1\, {\rm kW}$, so
\[\rm {1\, kW.h=\left(1000\,\frac{J}{s}\right)\left(3600\,s\right)=3.6 \times 10^6\, J=3.6\ MJ}\]
Therefore, the kilowatt-hour is a unit of work or energy not power.
When a body displaced $d\vec r$ while the force $\vec F$ acted on it, the small amount of work $dW$ is given by $dW=\vec F.d\vec r$. since we want the rate of change of work so divide both sides by $dt$ as follows
\[\frac{dW}{dt}=\vec F.\frac{d\vec r}{dt}\]
But we know the $\frac{d\vec r}{dt}$ is the velocity $\vec v$. Thus the power is found as
\[P=\vec F\cdot\vec v\]
In other words, the power delivered to the body by a force acting on it is the dot product of the force and the particle’s velocity. this is another relation for power.
