Simple Pendulum Problems and Formula for High Schools
A pendulum is a massive bob attached to a string or cord and swings back and forth in a periodic motion.
In the case of a massless cord or string and a deflection angle (relative to vertical) up to $5^\circ$, we can find a simple formula for the period and frequency of a pendulum as below \[T=2\pi\sqrt{\frac{\ell}{g}}\quad,\quad f=\frac{1}{2\pi}\sqrt{\frac{g}{\ell}}\] where $\ell$ is the length of the pendulum and $g$ is the acceleration of gravity at that place.
As you can see, the period and frequency of a simple pendulum do not depend on the mass of the pendulum bob.
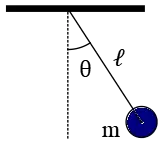
The period of a pendulum is defined as the time interval, in which the pendulum completes one cycle of motion and is measured in seconds.
In the following, a couple of problems about simple pendulum in various situations is presented.
Simple Pendulum Problems
Problem (1): In a simple pendulum, how much the length of it must be changed to triple its period? How about its frequency?
Solution: The period of a simple pendulum is related to its length $\ell$ by the following formula \[T=2\pi\sqrt{\frac{\ell}{g}}\] Here, we wish $T_2=3T_1$, after some manipulations we get \begin{align*} T_2&=3T_1\\\\ 2\pi\sqrt{\frac{\ell_2}{g}} &=3\times 2\pi\sqrt{\frac{\ell_1}{g}}\\\\ \sqrt{\ell_2}&=3\sqrt{\ell_1}\\\\\Rightarrow \ell_2&=9\ell_1 \end{align*} In the last equality, we squared both sides. Hence, the length must be nine times.
Problem (2): Find the length of a pendulum that has a period of 3 seconds then find its frequency.
Solution: The period and length of a pendulum are related as below \begin{align*} T&=2\pi\sqrt{\frac{\ell}{g}} \\\\3&=2\pi\sqrt{\frac{\ell}{9.8}}\\\\\frac{3}{2\pi}&=\sqrt{\frac{\ell}{9.8}} \\\\\frac{9}{4\pi^2}&=\frac{\ell}{9.8}\\\\\Rightarrow \ell&=9.8\times\left(\frac{9}{4\pi^2}\right)\\\\&=2.23\quad{\rm m}\end{align*} The frequency and periods of oscillations in a simple pendulum are related as $f=1/T$. Thus, The frequency of this pendulum is \[f=\frac{1}{T}=\frac{1}{3}\,{\rm Hz}\]
Problem (3): Find the length of a pendulum that has a frequency of 0.5 Hz. (Take $g=10 m/s^2$)
Solution: the frequency of a pendulum is found by the following formula \begin{align*} f&=\frac{1}{2\pi}\sqrt{\frac{g}{\ell}}\\\\ 0.5 &=\frac{1}{2\pi}\sqrt{\frac{10}{\ell}} \\\\ (2\pi\times 0.5)^2 &=\left(\sqrt{\frac{10}{\ell}}\right)^2\\\\ \Rightarrow \ell&=\frac{10}{4\pi^2\times 0.25}\\\\&=1\quad {\rm m}\end{align*}
Problems (4): The acceleration of gravity on the moon is $1.625\,{\rm m/s^2}$. How long of a simple pendulum must have there to produce a period of $2\,{\rm s}$.
Solution: The period of a simple pendulum is related to the acceleration of gravity as below \begin{align*} T&=2\pi\sqrt{\frac{\ell}{g}}\\\\ 2&=2\pi\sqrt{\frac{\ell}{1.625}}\\\\ (1/\pi)^2 &= \left(\sqrt{\frac{\ell}{1.625}}\right)^2 \\\\ \Rightarrow \ell&=\frac{1.625}{\pi^2}\\\\&=0.17\quad {\rm m}\end{align*} Therefore, a pendulum of length about 17 cm would have a period of 2 s on the moon.
Problem (5): To the end of a 2-m cord, a 300-g weight is hung. Then, we displace it from its equilibrium as small as possible and release it. Find the period and oscillation of this setup.
Solution: This configuration makes a pendulum. If displacement from equilibrium is very small, then the pendulum of length $\ell$ approximate simple harmonic motion. In this case, the period $T$ and frequency $f$ are found by the following formula \[T=2\pi\sqrt{\frac{\ell}{g}}\ , \ f=\frac{1}{T}\] As you can see, the period and frequency of a pendulum are independent of the mass hanged from it. \begin{gather*} T=2\pi\sqrt{\frac{2}{9.8}}=2.85\quad {\rm s} \\ \\ f=\frac{1}{2.85\,{\rm s}}=0.35\quad {\rm Hz}\end{gather*}
Problem (6): A pendulum, whose bob has a mass of $2\,{\rm g}$, is observed to complete 50 cycles in 40 seconds. (a) Find the frequency (b) the period and (d) its length.
Solution: (a) the number of complete cycles $N$ in a specific time interval $t$ is defined as the frequency $f$ of an oscillatory system or \[f=\frac{N}{t}\] Therefore, the frequency of this pendulum is calculated as \[f=\frac{50}{40\,{\rm s}}=1.25\, {\rm Hz}\]
(b) The period and frequency have an inverse relationship. Thus, the period is \[T=\frac{1}{f}=\frac{1}{1.25\,{\rm Hz}}=0.8\,{\rm s}\]
(c) Frequency of a pendulum is related to its length by the following formula \begin{align*} f&=\frac{1}{2\pi}\sqrt{\frac{g}{\ell}} \\\\ 1.25&=\frac{1}{2\pi}\sqrt{\frac{9.8}{\ell}}\\\\ (2\pi\times 1.25)^2 &=\left(\sqrt{\frac{9.8}{\ell}}\right)^2 \\\\ \Rightarrow \ell&=\frac{9.8}{4\pi^2\times (1.25)^2} \\\\&=0.16\quad {\rm m}\end{align*} Thus, the length of this kind of pendulum is about 16 cm.
Problem (7): There are two pendulums with the following specifications.
Pendulum A is a 200-g bob that is attached to a 2-m-long string. Pendulum B is a 400-g bob that is hung from a 6-m-long string. Which has the highest frequency?
Solution: As stated in the earlier problems, the frequency of a simple pendulum is proportional to the inverse of the square root of its length namely $f \propto 1/\sqrt{\ell}$.
To compare the frequency of the two pendulums, we have \begin{align*} \frac{f_A}{f_B}&=\frac{\sqrt{\ell_B}}{\sqrt{\ell_A}}\\\\&=\frac{\sqrt{6}}{\sqrt{2}}\\\\&=\sqrt{3}\end{align*} Therefore, the frequency of pendulum $A$ is $\sqrt{3}$ times the frequency of pendulum $B$.
Problem (8): A pendulum has a period of $1.7\,{\rm s}$ on Earth. What is its frequency on Mars, where the acceleration of gravity is about 0.37 that on Earth?
Solution: first find the period of this pendulum on Mars, then using relation $f=1/T$ find its frequency.
Recall that the period of a pendulum is proportional to the inverse of the gravitational acceleration, namely $T \propto 1/\sqrt{g}$.
Given that $g_M=0.37g$
Problem (9): Of simple pendulum can be used to measure gravitational acceleration. Consider a geologist that uses a pendulum of length $35\,{\rm cm}$ and frequency of 0.841 Hz at a specific place on the Earth. What is the acceleration of gravity at that location?
Solution: The length $\ell$ and frequency $f$ of a simple pendulum are given and $g$ is unknown. Put these information into the equation of frequency of pendulum and solve for the unknown $g$ as below \begin{align*} g&=(2\pi f)^2 \ell \\&=(2\pi\times 0.841)^2(0.35)\\&=9.780\quad {\rm m/s^2}\end{align*}
Problem (10): A clock works with the mechanism of a pendulum accurately. We move it to a high altitude. Will it gain or lose time during this movement?
Solution: Recall that the time period of a clock pendulum, which is the time between successive ticks (one complete cycle), is proportional to the inverse of the square root of acceleration of gravity, $T\propto 1/\sqrt{g}$. We know that the farther we go from the Earth's surface, the gravity is less at that altitude.
Therefore, with increasing the altitude, $g$ becomes smaller and consequently the period of the pendulum becomes larger.
Or at high altitudes, the pendulum clock loses some time.
Problem (11): A massive bob is held by a cord and makes a pendulum. We noticed that this kind of pendulum moves too slowly such that some time is losing. By how method we can speed up the motion of this pendulum?
Solution: Once a pendulum moves too fast or too slowly, some extra time is added to or subtracted from the actual time. On the other hand, we know that the period of oscillation of a pendulum is proportional to the square root of its length only, $T\propto \sqrt{\ell}$.
Thus, by increasing or decreasing the length of a pendulum, we can regulate the pendulum's time period.
In this problem has been said that the pendulum clock moves too slowly so its time period is too large. By shortening the pendulum's length, the period is also reduced, speeding up the pendulum's motion.
Problem (12): If the frequency of a 69-cm-long pendulum is 0.601 Hz, what is the value of the acceleration of gravity $g$ at that location?
Solution: The frequency of a simple pendulum is related to its length and the gravity at that place according to the following formula \[f=\frac {1}{2\pi}\sqrt{\frac{g}{\ell}}\] Solving this equation for $g$, we have \begin{align*} g&=(2\pi f)^2\ell\\&=(2\pi\times 0.601)^2(0.69)\\&=9.84\quad {\rm m/s^2}\end{align*}
Author: Ali Nemati
Page Created: 7/11/2021
© 2015 All rights reserved. by Physexams.com
AP® is a trademark registered by the College Board, which is not affiliated with, and does not endorse, this website.
