Relative Velocity Problems with Solutions: AP Physics 1

In this article, we solved some problems on relative velocities in one and two dimensions. We have chosen the problems in such a way that by solving them you can master this topic for the AP Physics 1 exam.

Relative Velocity Problems

Problem (1): A person walks across a raft at a speed of $0.5\,\rm m/s$ while his raft is traveling down the river at a speed of $1.8\,\rm m/s$ relative to the riverbank. Assuming that he walks perpendicular to the raft's motion relative to the river current, what is the person's velocity with respect to the river bank?

Solution: In this question, we are given the velocities in two directions, so it is a relative velocity problem in vector form. Let the river flows to the east and this direction is chosen as the positive $x$-direction. On the raft, the person walks due north and we choose this direction as the positive $y$-direction.

The safest method to analyze such problems is to draw a diagram and then apply the vector addition rules to obtain the desired quantity.

The river (water) flows at a speed of $1.8\,\rm m/s$ relative to the Ground (riverbank). We translate this phrase as $\vec{v}_{WG}$. On other hand, the velocity of the Person relative to the Raft is also designated as $\vec{v}_{PR}$.

The vectors in the figure below can be added together using the tail-to-tip method that yields \[\vec{v}_{PG}=\vec{v}_{PR}+\vec{v}_{RG}\] Note that this sum is of vector type.
Finding the relative velocity of a person walks across a raft while it is moving due east with respect to the ground

Tail-to-tip vector addition method: to add two vectors in this way, place the tail of the second vector on the tip of the first and connect the first tail to the tip of the second vector.

Here, the raft is moving in the direction of river flow to the right or $+x$-direction, i.e., $\vec{v}_{RG}=1.8(\hat{i}) \,\rm m/s$. On the other hand, the person is moving from one side to the other of the raft in the $+y$-direction, i.e., $\vec{v}_{PR}=0.5 (\hat{j}) \,\rm m/s $.

Therefore, the velocity vector of the person relative to the ground is written in the vector form as below \[\vec{v}_{PG}=0.5\,\hat{j}+1.8\,\hat{i}\] and its magnitude is also found using the Pythagorean theorem as follows \begin{align*} v_{PG}&=\sqrt{(1.8)^2+(0.5)^2} \\ &=1.87\,\rm m/s \end{align*} A you learned from vectors problems, the angle that this vector velocity is made with the positive $x$-axis is found using the following formula \begin{align*} \alpha &=\arctan\left(\frac{v_y}{v_x}\right) \\\\ &=\arctan\left(\frac{0.5}{1.8}\right) \\\\ &=15.5^\circ \end{align*} Therefore, from the point of view of an observer standing on the riverbank the person is walking across the raft at speed of about $1.8\,\rm m/s$.

Problem (2): A flatcar is moving to the right at a speed of $13\,\rm m/s$ relative to a person standing on the ground. Someone is riding a bicycle on the flatcar with a speed of (a) $5\,\rm m/s$ to the right, (b) $5\,\rm m/s$ to the left relative to that person on the ground. What is the velocity (speed and direction) of the bicycle relative to the flatcar?

Solution: In this problem about relative velocities along a line, three velocities are given. Let the positive $x$-direction be to the right.

The velocity of the Flatcar relative to the Person, i.e., $\vec{v}_{FP}=13 (\hat{i}) \,\rm m/s$.

The velocity of the Bicycle relative to the Person, i.e., (a) to the right (or $+x$-direction) $\vec{v}_{BP}=5(\hat{i})\,\rm m/s$ and (b) to the left (or $-x$-direction) $\vec{v}_{BP}=5(-\hat{i}) \,\rm m/s$.
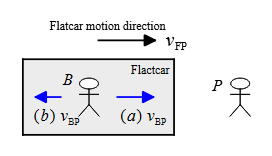
The relative velocity of a bicycle riding on a flatcar with respect to an standing observer on the ground.
We want to write the correct form of the vector addition for this case, but where should we know how to combine these velocities for one-dimensional motion?

One of the easiest methods to solve such problems is to apply the inner subscript cancellation method. Here, the Bicycle's velocity relative to the Person, $\vec{v}_{BP}$ is asked. We know that the Bicycle is riding across the Flatcar, and the Flatcar also moves relative to the ground.

Thus, adding a $F$ subscript between $BP$ leads to the following partitioning for velocities \[\vec{v}_{BF}=\vec{v}_{BP}+\vec{v}_{PF}\] On the other hand, we know $\vec{v}_{PF}=-\vec{v}_{FP}$. Gathering these two relations and substituting the numerical values into it gives for part (a) \begin{align*} \vec{v}_{BF}&=\vec{v}_{BP}-\vec{v}_{FP} \\\\&=5-13\\\\&=-8\,\rm m/s \end{align*} The minus sign indicates that the bicycle is moving to the left ($-\hat{i}$) or in the opposite direction of the flatcar's motion.

Part (b) is left to you as a practice problem.

Problem (3): A boat with a speed of $10\,\rm m/s$ relative to the water leaves the south bank in the direction of $37^\circ$ west of north. The river is $300-\rm m$-wide and flows due east at a speed of $2\,\rm m/s$. What are the (a) magnitude and (b) direction of the boat's velocity relative to the ground? (c) How long does the boat take to reach the other side of the river?

Solution: This type of relative motion problem in two dimensions requires some information about resolving vectors into unit vectors along the $x$ and $y$ axes.

Sketching a vector diagram and applying the tip-to-tail vector sum rule to find the unknown velocity is not always the right method.

In this question, we see that the two velocities are not perpendicular to each other, so the triangle that is made is not a right triangle and correspondingly, we cannot use the Pythagorean theorem or angle formula to find its magnitude and direction.

When the given velocities were perpendicular to each there, then we could use the following well-known vector addition equation \[\vec{v}_{BG}=\vec{v}_{BW}+\vec{v}_{WG}\]

Here, we clearly see that the two given velocities are not perpendicular, so the vector diagram is not a useful way and it is better to apply the method of vector addition by vector components.
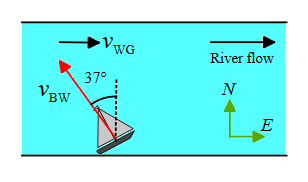
The velocity of a boat heading west of north across a river flowing due east relative to the ground. t
We are given the following information: the Boat's speed relative to the Water, i.e., $v_{BW}=10\,\rm m/s$, the speed of Water with respect to the Ground, i.e., $v_{WG}=2\,\rm m/s$. As you can see in the figure below, $\vec{v}_{BW}$ is resolved along the $x$ and $y$ axes as \[\vec{v}_{BW}=10\sin 37^\circ(-\hat{i})+10\cos 37^\circ \hat{j}\]
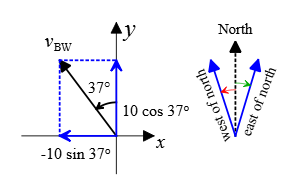
Resolving a vector along the x and y axes along with the definition of west of north and east of north.
The water flows also due east or the positive $x$-direction, i.e., $\vec{v}_{WG}=2(\hat{i}) \,\rm m/s$.

The combined velocities give us the components of the Boat's velocity relative to the Ground \begin{gather*} \vec{v}_{BG}=\vec{v}_{BW}+\vec{v}_{WG} \\\\ =\left(10\sin 37^\circ(-\hat{i})+10\cos 37^\circ \hat{j}\right)+2\,\hat{i} \\\\ =(-10\sin 37^\circ+2) \hat{i}+10\cos 37^\circ \hat{j} \\\\ =-4\hat{i}+8\hat{j} \end{gather*} Now, given this we can simply find the direction that this vector makes with the standard direction as measured counterclockwise from the positive $x$-direction by the following formula \begin{align*} \alpha&=\arctan\left(\frac{v_y}{v_x}\right) \\\\ &=\arctan\left(\frac{8}{-4}\right) \\\\ &=-63^\circ \end{align*} But the story is not over yet! Whenever a vector is in the second or third quadrant, the angle obtained by the above formula must be subtracted from the $180^\circ$ to give the true angle measured counterclockwise from the $+x$-direction.
Vector diagram for this relative velocity problem
In this case, $\vec{v}_{BG}$ lies in the second quadrant. Therefore, the correct angle is \[\beta=180^\circ-63^\circ=\boxed{117^\circ}\] If this angle is subtracted from $90^\circ$, then we can find its direction relative to the north direction. In this case, the boat's velocity relative to the ground heads $27^\circ$ west of north, as illustrated in the figure.

Finally, a lengthy question is over.

Problem (4): Ali, driving north at speed of $50\,\rm mph$, and Sara, driving east at $45\,\rm mph$, are approaching an intersection. What is Ali's speed as seen by Sara?

Solution: This problem involves two velocities in two dimensions, so it is a relative velocity question using vectors. Thus, it is better to establish a coordinate system in which the $x$ and $y$ axes correspond to the east and north directions.

Ali and Sara are driving relative to the Ground at speeds of $\vec{v}_{AG}=50(\hat{j}) \,\rm mph$ and $\vec{v}_{SG}=45(\hat{i}) \,\rm mph$. In all relative velocity problems, there are always two reference frames. In this case, the ground is the one and the other can be Ali or Sara.

Ali's velocity relative to Sara, i.e., $v_{AS}$ is unknown and needs to be determined. We only have Ali's and Sara's speeds relative to the ground, $v_{Ag}$ and $v_{Sg}$. To write the correct vector addition $v_{AS}$, we apply the inner subscript cancellation method by inserting ground (G) between Ali (A) and Sara (S) subscripts as follows \[\vec{v}_{AS}=\vec{v}_{AG}+\vec{v}_{GS}\] Now if look at this relation carefully, you notice that in the problem the velocity of Sara relative to the ground, $v_{SG}$ was given. $v_{GS}$ means the Ground's velocity relative to Sara. If Sara moves toward the right relative to the ground, the ground also moves to the left as seen by Sara.

Keep in mind, as a rule, that in all relative velocities we have the following important note about two reference frames moving relative to each other \[\vec{v}_{AB}=-\vec{v}_{BA}\] Applying this fact to the above vector sum, we have \begin{align*} \vec{v}_{AS}&=\vec{v}_{AG}+\vec{v}_{GS} \\\\ &=\vec{v}_{AG}-\vec{v}_{SG} \\\\ &= 50\hat{j}-45\hat{i} \end{align*} Given this vector, applying the Pythagorean theorem gives us his magnitude (Ali's speed) \[v_{AS}=\sqrt{50^2+(-45)^2}=67.2\,\rm mph\] And his direction as seen by Sara is found using the following formula measured counterclockwise from the $+x$-direction. \begin{align*} \alpha&=\arctan\left(\frac{v_y}{v_x}\right) \\\\ &=\arctan\left(\frac{50}{-45}\right) \\\\ &=-48.0^\circ \end{align*} There is a subtlety about using this formula to find the direction. If a vector is in the second or third quadrant, then add $180^\circ$ to the angle obtained by the above formula to find the correct angle. Here, $\vec{v}_{AS}$ is in the second quadrant. Thus, we have \[\beta=180^\circ-48^\circ=\boxed{132^\circ}\]

Problem (5): Two cars are approaching at right angles to a corner. Car $1$ moves due east at a speed of $v_{1g}=45\,\rm m/s$ with respect to the ground and car $2$ due north at $v_{2g}=35\,\rm m/s$.
(a) What is the relative velocity of car $1$ as seen by car $2$?
(b) What is the relative velocity of car $2$ as seen by car $1$?

Solution: Assign a coordinate system in which the east and north directions correspond to the $x$ and $y$ axes. We simplify our known as below:
$\vec{v}_{1g}=45(\hat{i}) \,\rm m/s$ and $\vec{v}_{2g}=35(\hat{j}) \,\rm m/s$.

(a) We label the velocity of car $1$ relative to car $2$ by $\vec{v}_{12}$, add ground g as an inner subscript between $12$, and write the vector addition equation as below \begin{align*} \vec{v}_{12}&=\vec{v}_{1g}+\vec{v}_{g2} \\\\ &=\vec{v}_{1g}-\vec{v}_{2g} \\\\ &= 45\hat{i}-35\hat{j} \end{align*} This velocity vector lies in the fourth quadrant whose magnitude with the positive $x$ direction is found as \begin{align*} \alpha&=\arctan\left(\frac{v_y}{v_x}\right) \\\\ &=\arctan\left(\frac{-35}{45}\right) \\\\ &=\boxed{-38^\circ} \end{align*} The negative indicates an angle below the horizontal.
The Pythagorean theorem gives its magnitude (speed) \[v_{12}=\sqrt{45^2+(-35)^2}=57\,\rm m/s\] In summary, from the point of view of car $2$, car $1$ moves with a speed of $57\,\rm m/s$ at $38^\circ$ angle below horizontal or $38^\circ$ south of east.

(b) Similar to the previous part, solve this section.

\item Problem (6): A boat is traveling due north at $6\,\rm m/s$ while a cruise ship heads $45^\circ$ north of east at $4\,\rm m/s$. What are the $x$ and $y$ components of the velocity of the boat relative to the cruise ship?

Solution: Take the north and east as positive $y$ and $x$ directions. North of east means you first stand facing east then turn leftward. The cruise ship's velocity vector lies in the first quadrant with the following components \[\vec{v}_{CG}=4(\cos 45^\circ\hat{i}+\sin 45^\circ\hat{j})\] The Boat's velocity relative to the Ground is also given as $\vec{v}_{BG}=6(\hat{j}) \,\rm m/s$. Now to find the cruise's velocity relative to the boat, we can either draw a vector diagram and use it to write the correct form of vector addition or use the inner subscript cancellation method.

Since the two velocities are not perpendicular to each other, the vector diagram isn't a helpful method.

We are asked $v_{BC}$. The inner subscript cancellation method tells us to add the Ground G between the $BC$ subscripts (since we are given only $v_{BG}$ and $v_{CG}$) as below \[\vec{v}_{BC}=\vec{v}_{BG}+\vec{v}_{GC}\] Now using the fact that $\vec{v}_{GC}=-\vec{v}_{CG}$, we have \begin{align*} \vec{v}_{BC}&=\vec{v}_{BG}-\vec{v}_{CG} \\\\ &=6\hat{j}-4(\cos 45^\circ\hat{i}+\sin 45^\circ\hat{j}) \\\\ &=-2\sqrt{2} \hat{i}+(6-2\sqrt{2}) \hat{j} \end{align*} The Pythagorean theorem gives us its magnitude or the speed of Boat relative to the cruise ship. \begin{align*} v_{CG}&=\sqrt{\left(-2\sqrt{2}\right)^2+\left(6-2\sqrt{2}\right)^2} \\\\ &=4.2\,\rm m/s\end{align*} Hint, take $\sqrt{2}=1.4$ and use a calculator to solve this relation. Finally, its direction measured counterclockwise from the positive $x$-axis is \begin{align*} \alpha&=\arctan\left(\frac{v_y}{v_x}\right) \\\\ &=\arctan\left(\frac{6-2\sqrt{2}}{-2\sqrt{2}}\right) \\\\ &=-66^\circ \end{align*} Again, $\vec{v}_{BC}$ is in the second quadrant, so the true angle is \[\beta=180^\circ+(-66^\circ)=\boxed{114^\circ}\]

Problem (7): A person in a basket of a hot-air balloon throws a ball horizontally into the air with a speed of $6\,\rm m/s$. As seen by an observer standing on the ground, what initial velocity does the ball have if, the balloon is
(a) rising at $2\,\rm m/s$ relative to the ground, (b) descending at $2\,\rm m/s$ relative to the ground?

Solution: We are given the ball's velocity relative to the Balloon, say to the right horizontally, i.e., $\vec{v}_{bB}=6(\hat{i}) \,\rm m/s$. For this problem, we adopt a coordinate system in which up and right correspond to the $\hat{j}$ and $\hat{i}$, respectively.

(a) In this part, the Balloon's velocity (magnitude and direction) with respect to the ground is given, i.e., $\vec{v}_{BG}=2(\hat{j}) \,\rm m/s$. The best and easiest method to solve every relative motion question in two dimensions is using the $\hat{i}$ and $\hat{j}$ approach.

The unknown is the ball's velocity relative to the ground, i.e., $\vec{v}_{bG}$. We can simply combine these velocities using the cancellation of inner subscripts method as follows \begin{align*} \vec{v}_{bG}&=\vec{v}_{bB}+\vec{v}_{BG} \\\\ &=6\hat{i}+2\hat{j} \end{align*} Pay attention that the ball moves relative to the Balloon and the Balloon is also moves relative to the Ground, so we can add Balloon as a middle subscript between $v_{bG}$ and break it into two parts as $v_{bB}$ and $v_{BG}$. Given the velocity vector $\vec{v}_{bB}$, one can find its magnitude \[v_{bB}=\sqrt{6^2+2^2}=6.3\,\rm m/s\] and its direction as measured counterclockwise from $+x$-direction \begin{align*} \alpha&=\arctan\left(\frac{y-component}{x-component}\right) \\\\ &=\arctan\left(\frac{2}{6}\right) \\\\ &=18.5^\circ \end{align*} Therefore, from the point of view of an observer standing on the ground, he/she sees that the ball is thrown with an initial speed of $6.3\,\rm m/s$ at an angle of about $18^\circ$ above the horizontal.

(b) In this case, the Balloon is descending at $2\,\rm m/s$ relative to the Ground, i.e., $\vec{v}_{BG}=2(-\hat{j}) \,\rm m/s$. Similar to the previous part, the ball's velocity relative to the Ground is written in vector addition form as below \begin{align*} \vec{v}_{bG}&=\vec{v}_{bB}+\vec{v}_{BG} \\\\ &=6\,\hat{i}+2(-\hat{j}) \end{align*} Given this, its magnitude and direction are found as below \begin{align*} v_{bB}&=\sqrt{6^2+(-2)^2}=6.3\,\rm m/s\end{align*} and \begin{align*} \alpha&=\arctan\left(\frac{v_y}{v_x}\right) \\\\ &=\arctan\left(\frac{-2}{6}\right) \\\\ &=-18.5^\circ \end{align*} Thus, the observer standing on the ground, sees the ball is thrown away from the balloon at about $18^\circ$ below the horizontal with the same speed as before.

Problem (8): An aircraft is flying in a crosswind due north while its speed indicator shows $350\,\rm km/h$ relative to the air. The wind also blows from west to east at a constant speed of $50\,\rm km/h$ relative to the ground. What is the direction and speed of the aircraft relative to the ground?

Solution: First of all, draw a vector diagram and illustrate all the given velocities on it then apply the tip-to-tail vector sum rule to write the correct form of the combined velocities.

In this question, it's said explicitly that the two velocities make a right angle with each other, one is moving to the north and the other to the east.

The given information is the plane's speed relative to the air, i.e., $v_{PA}=350\,\rm km/h$, and the Air's speed with respect to the Ground, i.e., $v_{AG}=50\,\rm km/h$.

The vector diagram above illustrates that the correct form of vector addition reads \[\vec{v}_{PG}=\vec{v}_{PA}+\vec{v}_{AG}\] Applying the Pythagorean theorem gives us the plane's velocity relative to the ground \begin{align*} v_{PG}&=\sqrt{(350)^2+(50)^2} \\\\ &=354\,\rm km/h \end{align*} The vector addition, in this case, form a right triangle so we don't need the velocity components to find the direction (a long way). In such cases, according to the figure of the right triangle, we use the definition of one of the trigonometry functions.

The direction of the plane, as seen from the point of view of an observer standing on the ground, is found by applying the definition of the tangent function and then taking its inverse as below \begin{align*} \alpha&=\arctan\left(\frac{50}{350}\right) \\\\ &=8^\circ \end{align*}

Problem (9): A $500-\rm m$-wide river flows due south at a constant speed of $3\,\rm m/s$. A man in a motorboat travels across the river due east at $5\,\rm m/s$.
(a) What is the velocity (magnitude and direction) of the boat relative to the earth (ground)?
(b) How much time is required for the man to cross the river?
(c) Starting from the south bank, how far will he reach the opposite bank?

Solution: Again, sketch a vector diagram and indicate all the velocity vectors on it. Take east as the positive $x$-direction, and north as the positive $y$-direction.

The river flows southward or the negative $y$-direction relative to the ground (or an observer standing on the riverbank), so it has no $x$ component, i.e., $\vec{v}_{RG}=3\,\rm m/s\, (-\hat{j})$.

On the other hand, the boat travels eastward or the positive $x$-direction relative to the river flow (water), i.e., $\vec{v}_{BW}=5\,\rm m/, \, (\hat{i})$.

(a) The boat's velocity with respect to the ground, $\vec{v}_{BG}$, is the vector sum of its velocity relative to the water, $\vec{v}_{BW}$, plus the velocity of the water with respect to the ground (river bank), $\vec{v}_{WG}$ \begin{align*} \vec{v}_{BG}&=\vec{v}_{BW}+\vec{v}_{WG} \\\\ &=5\,\hat{i}+3\,(-\hat{j}) \end{align*}
(a) As indicated in the vector diagram above, $\vec{v}_{BW}$ is perpendicular to $\vec{v}_{WG}$, so applying the Pythagorean theorem gives us $v_{BG}$ \begin{align*} v_{BG}&=\sqrt{v_{BW}^2+v_{WG}^2} \\\\ &=\sqrt{5^2+(-3)^2} \\\\ &=5.8\,\rm m/s \end{align*} To find the direction either we can use the figure and the definition of the $sin$ function or use the standard formula once the components are given.

From the figure, we have \[\sin\theta=\frac{v_{WG}}{v_{BG}}=\frac{3}{5.8}\] Taking the inverse sine, $\arcsin$ or $\sin^{-1}$, from both sides, gives \[\theta=\sin^{-1}\left(\frac{3}{5.8}\right)=31.1^\circ\]
(b) The time required for the man (or motorboat) to cross the river is obtained using the definition of average velocity, $v=\frac{D}{t}$. Given the river's width $D=500\,\rm m$, and the corresponding velocity in this direction $v_{BW}$, we have \[t=\frac{D}{v_{BW}}=\frac{500}{5}=100\,\rm s\] Thus, it takes $100\,\rm s$ or about $1.7$ minutes for the man to travel the width of the river.

(c) During the time the boat crosses the river's width, the water stream with a speed of $v_{WG}$ will displace the boat downstream, a distance of \[ d=v_{WG}t=3\times 100=300\,\rm m\]

Summary:

When object A moves relative to object B with velocity $\vec{v}_{AB}$, and B also travels relative to object C (or an observer) with $\vec{v}_{BC}$, then the velocity of object A with respect to object C, designated by $\vec{v}_{AC}$, is determined by the following vector addition equation \[\vec{v}_{AC}=\vec{v}_{AB}+\vec{v}_{BC}\]

Author: Dr. Ali Nemati
Published: Feb 2, 2023