Magnetic Flux Formula with Solved Problems
In this tutorial, we show you how to use the magnetic flux formula in physics to find flux through loops, solenoids, coils, and other geometries.
Definition of magnetic flux:
The amount of magnetic field lines passing through a loop of area A which is tilted at angle $\theta$ from the field is defined as magnetic flux and is found by the below formula \[\Phi_m=BA \cos \theta \] This definition is similar to that of electric field flux.
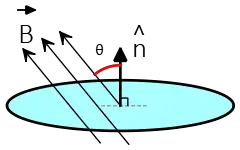
The SI unit of magnetic flux is the weber which can be seen from the formula above as \[1 \,weber={\rm 1\, Wb =1\, T\cdot m^2}\] $\theta$ in the magnetic flux formula is the angle between magnetic field vector $\vec{B}$ and the normal (perpendicular) $\hat{n}$ to the plane of the loop.
The above magnetic flux formula is similar to the vector scalar (dot) product of two vectors as $\vec{A}\cdot\vec{B}=AB\cos\theta$.
Thus, the equation for magnetic flux can be written as a dot product of two vectors magnetic field vector $\vec{B}$ and area vector $\vec{A}$ \[\Phi_m=\vec{B}\cdot\vec{A}\] where $\vec{A}$, area vector, is defined as a vector perpendicular to the plane of the loop with magnitude equal to the area of the loop $A$.
The SI unit of the area vector is $m^2$.
One of the most important applications of magnetic flux is in Faraday's law of induction.
That law states a changing magnetic flux with time creates a voltage.
Below, using the magnetic flux formula some magnetic flux problems are solved for more understanding.
Magnetic flux formula Problems
Problem (1): A square loop of side 3 cm is positioned in a uniform magnetic field of magnitude 0.5 T so that the plane of the loop makes an angle of $60^\circ$ with the magnetic field. Find the flux passing through the square loop.

Solution: Putting the known values into the magnetic flux equation $\Phi_m=BA\cos \theta$, we get \begin{align*}\Phi_m&=BA\cos \theta\\&=(0.5)(0.03\times 0.03)\cos 30^\circ\\&=0.39\quad {\rm mWb}\end{align*} Note that the $\theta$ in the formula above is the angle between $\vec{B}$ and a unit vector perpendicular to the surface.
In this example, the given angle $60^\circ$ is with the surface of the loop not with a vector perpendicular to the surface that is $\hat{n}$.
Problem (2): A circular loop of area $200\,{\rm cm^2}$ sits in the $xz$-plane. Then, a uniform magnetic field of $\vec{B}=0.3\,\hat{i}+0.4\,\hat{j}\, {\rm T}$ applied on it. Determine
(a) Magnitude of the magnetic field
(b) Magnetic flux through the square loop?
Solution:
(a) The magnitude of a vector such as $\vec{R}=R_x \hat{i}+R_y\hat{j}$ is found by formula \[R=\sqrt{R_x^2+R_y^2}\] so strength (magnitude) of the magnetic field is determined as \[B=\sqrt{(0.3)^2+(0.4)^2}=0.5\quad {\rm T}\]
(b) This circular loop is positioned at the right angle with the $y$ axis so a unit vector perpendicular to it is written as $\hat{n}=\hat{j}$. Now we use the scalar definition of magnetic flux as $\Phi_m=\vec{B}\cdot\hat{n}A$ to find it as below \begin{align*}\Phi_m&=\vec{B}\cdot\hat{n}A\\&=(0.3\hat{i}+0.4\hat{j})\cdot (\hat{j})\left(200\times 10^{-4}\right)\\&=(0.3\,\hat{i}\cdot\hat{j}+0.4\,\hat{j}\cdot\hat{j})(0.2)\\&=0.4\times 0.2\\&=0.08\quad {\rm T}\end{align*} Where in above we used the definition of the scalar product of Cartesian unit vectors. In addition, the SI unit of area, squared meter, is determined by the following conversion rule \[\rm{1\, cm^2=10^{-4}\,m^2}\]
Problem (3): A rectangular loop of dimensions 3 cm by 5 cm is placed perpendicular in a uniform magnetic field of magnitude 0.1 T. Find the magnetic flux through the loop.
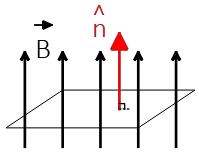
Solution: magnetic flux is defined as the product of magnetic field, surface area, and the angle between B and a unit vector perpendicular to the surface with the formula $\Phi_m=BA\cos \theta$.
Here, the loop is positioned perpendicular to the magnetic field $B$ namely the angle between $B$ and a unit vector normal to the surface $\hat{n}$ is zero $\theta=0^\circ$. Putting the values in the magnetic flux formula, we get \begin{align*} \Phi_m&=BA\cos \theta \\&=(0.1)(0.03\times 0.05)\cos 0^\circ\\&=15\times 10^{-5}\quad{\rm Wb}\\ or &=0.15\quad{\rm mWb}\end{align*}
Problem (4): Find the magnetic flux through a 100-turn coil of a radius of 10 cm that carries a current of 3.00 A.
Solution: the magnetic flux through one loop of the coil is $\Phi_1=BA\cos \theta$ where B is the magnetic field produced by the loop.
Recall that the magnitude of the magnetic field of a loop is $B=\frac{\mu_0 i}{2 R}$ which is perpendicularly directed into or out of the plane of the loop. Thus, the angle between $\vec{B}$ and the normal to the plane of the loop is $0^\circ$.
Substituting these values into the magnetic flux equation above, we get the flux through one loop as \begin{align*} \Phi_1&=BA\cos \theta \\ \\&=\frac{\mu_0 i}{2 R}\left(\pi R^2\right)\cos 0^\circ\\ \\&=\frac{4\pi\times 10^{-7}\times 3}{(2)(0.10)}\left(\pi\times(0.1)^2\right)\\ \\&=6\pi^2 \times 10^{-8}\quad {\rm Wb}\end{align*} This is the flux passes through one loop of a coil with $N$ such loops, so the total flux through the coil is \begin{align*}\Phi_m&=N\Phi_1\\&=100\times \left(6\pi^2\times 10^{-8}\right)\\&=0.6 \quad {\rm \mu Wb}\end{align*}
Problem (5): Find the magnetic flux through a 1000-turn solenoid of radius 10 cm and a length equal to 30 cm so that a current of 3 A passes through it.
Solution: Recall that the magnetic field inside a tightly wrapped long solenoid (neglecting the end effects) is uniform with magnitude $B=\mu_0 \frac{N}{L}i$ where $N$ and $L$ are the number of turns and the length of the solenoid, respectively. \begin{align*}B&=\mu_0 \frac NL i\\&=(4\pi\times 10^{-7})\left(\frac{1000}{0.3}\right)(3)\\&=12.56\quad{\rm mT}\end{align*}
On the other hand, each loop of the long solenoid is perpendicular to its uniform magnetic field so $\theta =0^\circ$. The area of one loop is also \[A=\pi r^2=\pi(0.1)^2=0.0314\quad {\rm m^2}\] Substituting the given values above into the equation for magnetic flux, we have \begin{align*} \Phi_1&=BA\cos \theta\\&=(12.56\times 10^{-3})(0.0314)\cos 0^\circ\\&=0.4\times 10^{-3}\quad {\rm Wb}\end{align*}This is the total magnetic flux through $N$ turns of a long solenoid.
Problem (6): A circular loop of a radius of 10 cm is placed perpendicular to a uniform magnetic field with an unknown strength. The magnitude of the flux through the loop is $5\times 10^{-3}\,{\rm T.m^2}$.
(a) What is the magnitude of the magnetic field?
(b) Now, rotate the magnetic field B such that it is parallel to the plane of the loop. How many magnetic field lines pass through the loop?
Solution: the product of magnetic field, surface area, and the angle between $\vec{B}$ and the direction perpendicular to the plane of the surface $\hat{n}$ gives the magnetic flux formula, $\Phi_m=BA\cos \theta$.
(a) $B$ is perpendicular to the surface, so the angle between them is zero, $\theta =0$. Substituting the values into the equation above and solving for magnetic field $B$, we get \begin{align*} \Phi_m&=BA\cos \theta\\ 5\times 10^{-3}&=B (\pi\times (0.1)^2)\cos 0\\ \Rightarrow B&=\frac{5\times 10^{-3}}{\pi \times 10^{-2}}\\ \\&= 0.16 \quad {\rm T}\end{align*}
(b) $B$ is parallel to the loop, namely, it makes an angle of $\theta=90^\circ$ with a vector perpendicular to the loop surface. In this case, the flux through the loop is \begin{align*} \Phi_m&=BA\cos \theta\\&=(0.16)(\pi\times(0.1)^2)\cos 90^\circ\\&=0\end{align*}
Problem (7): A square coil of side length $a=2\,{\rm m}$ is placed in the $xy$-plane in a uniform magnetic field of magnitude 0.1 T as shown in the figure. The direction of the magnetic field is toward the $y$ direction. Find the total magnetic flux through the coil.
Solution: magnetic flux is the scalar product of the magnetic field and the area vector with formula $\Phi_m=\vec{B}\cdot\vec{A}$. As you can see, all factors involved in this equation are vectors.
In this example, the magnetic field vector and area vectors are written as $\vec{B}=0.1\,\hat{j},{\rm T}$ and $\vec{A}=4\,\hat{i},{\rm m^2}$. Putting these vectors in the formula above and using the definition of scalar product, we get \begin{align*} \Phi_m&=\vec{B}\cdot\vec{A}\\&=(0.1\,\hat{j})\cdot (4\,\hat{i})\\&=0.4\,(\hat{j}\cdot\hat{i})\\&=0.4\times 0\\&=0\end{align*}
Problem (8): A uniform magnetic field of magnitude B is passing through the base of a hemisphere with radius R. How many field lines penetrate the spherical surface of the hemisphere?
Solution: magnetic flux is a measure of how many magnetic field lines pass through a surface which is computed by the formula $\Phi_m=BA\cos \theta$.
The magnetic flux through the base of the hemisphere is \begin{align*}\Phi_m&=B(A)\cos 180^\circ\\&=-B(\pi R^2)\end{align*} To find the flux through the spherical surface we can use directly the definition of magnetic flux in spherical coordinates (which is hard) or use Gauss's law for magnetism as \[\oint\vec{B}\cdot \hat{n}\,dA=0\] According to this law, the magnetic flux through ANY closed surface is always ZERO.
A hemisphere is composed of two parts, one is a flat base and the other is a spherical part. Total flux $\Phi_m$ through it, which is the sum of the fluxes through the base $\Phi_{m-b}$ and the curved surface $\Phi_{m-S}$, must be zero so the flux passing through the curved surface is obtained as below \begin{align*} \Phi_m&=\Phi_{m-b}+\Phi_{m-S}\\ 0&=-B\pi R^2 +\Phi_{m-S} \\\Rightarrow \Phi_{m-S}&=+B\pi R^2 \end{align*}
Author: Dr. Ali Nemati
Published: 2/7/2021
© 2015 All rights reserved. by Physexams.com
AP® is a trademark registered by the College Board, which is not affiliated with, and does not endorse, this website.
