- Home
- Physics Problems
- Problems
Solved Problems
A $12\, {\rm cm}$ diameter, $2\,{\rm kg}$ uniform circular disk, which is initially at rest, experiences the net torque shown in the figure below. What is the disk's angular velocity at $t=12\, \mathrm{s}$? The disk rotates about an axis perpendicular to the plane of the disk and through its center. Note: $I_{disk}=\frac{1}{2}MR^2$

\[\tau =I\alpha =I\frac{d\omega }{dt}\ \Longrightarrow \frac{d\omega }{dt}=\frac{\tau }{I}\]
\[\omega =\frac{1}{I}\underbrace{\int^t_0{\tau \left(t\right)dt}}_{area}\]
Instead of evaluate the above integral, compute the area under the $\tau -t$ diagram
\[\omega =\frac{1}{I}\left(area\right)=\frac{1}{0.5\times 2\times {\left(0.06\right)}^2}\left(\frac{1}{2}\left(0.2\right)\left(8\right)-\frac{1}{2}\left(-0.1\right)\left(4\right)\right)=170\ \frac{\mathrm{rad}}{\mathrm{s}}\]
A uniform rectangular beam of length $L=5\, \mathrm{m}$and mass $M=40\, \mathrm{kg}$ is supported but not attached to the two posts which are length $D=3\, \mathrm{m}$ apart. A child of mass $W=20$ kg starts walking along the beam.
(a) Assuming infinitely rigid posts, how close can the child get to the right end of the beam without it falling over? [Hint: The upward force exerted by the left on the beam cannot be negative --this is the limiting condition on how far the child can be to the right. Set up the static equilibrium condition with the pivot about the left end of the beam.]
(b) Suppose the left end of the beam is attached to the left post although it can still freely pivot about it. The right post is not attached as in part a). If the left post is infinitely rigid while the Young's modulus for the right post which is of length $s=10\, {\rm m}$ is $Y={10}^{10}\, \mathrm{N/}{\mathrm{m}}^{\mathrm{2}}$ and its cross sectional area is $A=\frac{1}{2}\,{\mathrm{m}}^{\mathrm{2}}$, how much does its length differ between the situation when the child is not present and when the child is at the rightmost edge of the beam?
(a) Take the pivot about the left end. Identify all of the forces that act on the beam. Since we want the beam to be in static equilibrium after the child reaches the desired point so apply this condition to the beam:
\[\Sigma \vec F_y=0\to \ mg+wg=F_1+F_2\]
Where $F_1$ and $F_2$ are the normal forces acted by the posts on the beam.

All of the above forces create the following torque about left post:
\[\Sigma \vec \tau=\vec r \times \vec F=0 \Rightarrow F_1D=Mg\,\frac{L}{2}+wg\left(L-x\right)\]
Since $F_2\ge 0$ and $F_1D\le \left(Mg+wg\right)D$ then
\[Mg\,\frac{L}{2}+wg\left(L-x\right)\le \left(M+w\right)gD\Rightarrow \ -\frac{mg}{wg}\left(D-\frac{L}{2}\right)+\left(L-D\right)\le x\ \]
\[\Longrightarrow x\ge 1\ \mathrm{m}\]
(b) Since now the beam is attached to the left post, the force on the left end of the beam can point down. Hence, the child can easily be all the way to the right.
$F_1D\cong Mg\frac{L}{2}+wgL\Rightarrow \ F_1\approx Mg\ \left(\frac{1}{2}\frac{L}{D}\right)+wg\frac{L}{D}$
Let $\mathrm{\Delta }S$ be the compression of post 2. Hence $\mathrm{\Delta }S_{child\ on}\approx \left(\frac{F_1}{A}\right)\frac{S}{Y}$.
With the child off, $F^{'}_1\approx Mg\ \left(\frac{L}{2D}\right)$ and $\mathrm{\Delta }S_{child\ off}\approx \left(\frac{F^{'}_1}{A}\right)\frac{S}{Y}$
\[\therefore \mathrm{\Delta }S_{child\ on}-\mathrm{\Delta }S_{child\ off}\approx \frac{\left(F_1-F^{'}_1\right)}{A}\frac{S}{Y}=\left(\frac{wg\frac{L}{D}}{A}\right)\frac{S}{Y}=\left(\frac{327}{\frac{1}{2}}\right)\frac{10}{{10}^{10}}\approx 7\times {10}^{-7}\mathrm{m}\]
A grindstone spinning at the rate of $8.3\ \mathrm{rev/s}$ has what approximate angular speed?
The angular speed in the rotational motions with period $T$ and frequency $f$ is defined by
\[\omega =\frac{2\pi }{T}=2\pi f\]
Where $T$ is in seconds and $f$ in Hertz thus $\omega $ has unit $\mathrm{rad/s}$. So only convert $\mathrm{rev/s}$ to $\mathrm{rad/s}$.
\[1\ rev=2\pi \ \mathrm{rad}\]
\[\omega =8.3\frac{\mathrm{rev}}{\mathrm{s}}\left(2\pi \frac{\mathrm{rad}}{\mathrm{1\ rev}}\right)=52\frac{\mathrm{rad}}{\mathrm{s}}\]
The figure below represents a $2.0\, {\rm m}$ long bar pinned at point A. compute the net torque on the bar with respect to point A due to the forces $F_1=50\, \mathrm{N}$ applied 1.0 m from point A, and $F_2=100\,\mathrm{N}$ as shown in the figure below.

The torque about an axis or point is defined as the cross product of moment arm distance-vector $\vec{r}$ and force $\vec{F}$ which produce rotation.
\[\vec{\tau }=\vec{r}\times \vec{F}\]
Note: the moment arm is the minimum distance between the pivot point and the line of action (the line along which the forces act)
First, find the magnitude of the torques due to the forces $F_1$ and $F_2$ about the pivot point $A$.
\[\left|{\vec \tau }_1 \right|=r_1F_1\,{\sin \theta \ }=\left(\frac{L}{2}\right)F_1\,{\sin 90{}^\circ \ }=50\ \mathrm{\ N}\]
Where $\theta $ is the angle between the lever arm and force vector $\vec F$.
\[\left|{\vec \tau }_2\right|=r_2F_2\,{\sin \theta \ }=LF_2\,{\sin 60{}^\circ \ }=2\times 100\times \frac{\sqrt{3}}{2}=100\sqrt{3}\]
The torque is a vector quantity so use the right-hand rule to find its direction. Curl your fingers of the right hand from the direction of $\vec r$ into the direction of $\vec F$. Then your right thumb points in the direction of torque $\vec \tau$ (or the direction of curling the right fingers shows the direction of torque as clockwise or counterclockwise). Let us consider the positive direction to be out of the page (counterclockwise) and vice versa. So \[{\vec \tau }_1=-50\ \mathrm{N} \ \ and \ \vec \tau_2=+100\sqrt{3}\ \mathrm{N}\]
The total torque exerted on the pivot point $A$ is the sum of them
\[\mathrm{\Sigma }{\vec \tau }_A=+100\sqrt{3}-50=+123.2\ \mathrm{N}\]
Thus the total torque is counter clockwise.
A physical pendulum has a shape of a disk of radius $r$ and mass $m$. The pendulum swings about an axis perpendicular to the plane of the disk and at a distance $L$ from the center of the disk.
(a) What is the moment of inertia of the disk about the axis where the pendulum swings?
(b) What is the oscillation frequency of this pendulum?
(c) For what value of $L$ is this frequency at a maximum?
($I_{disk}$ about its center of mass is $\frac{1}{2}mr^2$- assume that the oscillation amplitude is small and small angle approximation is ${\sin \theta \ }\sim \theta $ and ${\cos \theta \ }\sim 1-\frac{{\theta }^2}{2}$ )

(a) Note: parallel axis theorem tells us that the moment of inertia is minimal when the rotation axis passes through the center-of-mass(CM) and increases as the rotation axis are moved further from the (CM). i.e. $I=I_{cm}+mh^2$, where $h$ is the distance from the CM to the parallel axis of rotation.
So, $I=\frac{1}{2}mr^2+mL^2$
(b) The radial part of $mg$ will be balanced by the tension on the cord (so there is no radial acceleration).
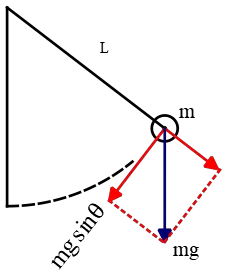
The tangential part of it provides the torque on the pendulum.
\begin{gather*} \vec{\tau }=I\alpha =I\frac{d^2\theta }{dt^2}\\\\ \Rightarrow \quad -mgL\,{\sin \theta}=I\frac{d^2\theta}{dt^2} \\\\ \therefore \frac{d^2\theta}{dt^2}+\frac{mgL}{I}{\sin \theta}=0\end{gather*} Thus, the small angle approximation gives us \[\frac{d^2\theta}{dt^2}+\frac{mgL}{I}\theta \approx 0\]
Note: In above the minus sign is there because the torque opposes the angular displacement from equilibrium.
By considering $\frac {mgL}{I}$ as the squared angular momentum $\omega^2$, the above equation have similar form of an SHM equation, therefore
\begin{gather*}{\omega}^2=\frac{mgL}{I}=\frac{mgL}{mL^2+\frac{1}{2}mr^2} \\\\ f=\frac{\omega}{2\pi} \end{gather*}
Practice more problems on simple harmonic motions (SHM).
(c) To find the extremes of a function, we must take the derivative of it.If $\omega $ is an extreme, so ${\omega }^2$ is also, therefore we can examine $d{\omega }^2/dL$ rather $d\omega /dL$
\begin{gather*} \frac{d{\omega }^2}{dL}=1-\frac{r^2}{L^2}\frac{1}{1+r^22L^2}=0\\\\ \Longrightarrow \quad L=R/\sqrt{2}\end{gather*}
It is less than $R$!
More related article:
Simple pendulum problems
Two astronauts, each having a mass $M$, are connected by a rope of length $d$ having negligible mass. They are isolated in space, orbiting their central mass at speeds $v$. Treating the astronauts as particles, calculate
(a) The angular momentum of the system
(b) The rotational energy of the system
By pulling on the rope, one of the astronauts shortens the distance between them to $d/2$.
(c) What is the new angular momentum of the system?
(d) What are the astronauts' new speeds?
(e) What is the new rotational energy of the system?
(f) How much work does the astronaut do in shortening the rope?
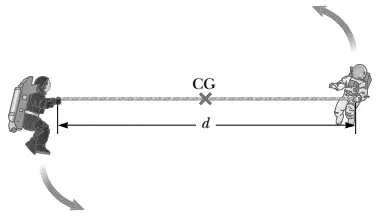
(a) Recall that the angular momentum of a rotational object is given by $\vec{L}=\vec{r}\times \vec{p}$. So the magnitude of the angular momentum about CM for this case is
\[\left|\vec{L}\right|=Mv\left(\frac{d}{2}\right)+Mv\left(\frac{d}{2}\right)=dMv\]
By the Right hand rule, the direction of it is out of page.
(b) The rotational energy of a rotating system defines as $K_r=\frac{1}{2}I{\omega }^2$, where $\omega $ is angular velocity $\vec{v}=\vec{\omega }\times \vec{r}$ and $I=\Sigma m_ir_i^2$ is the moment of inertia of the system.
In this problem, $\vec \omega \bot \vec r\ \Rightarrow \omega =\frac{v}{r}$
\[K_r=\frac{1}{2}\left(Mr^2\right){\left(\frac{v}{r}\right)}^2=\frac{1}{2}Mv^2\]
\[K_{r,tot}=2K_r=2\left(\frac{1}{2}Mv^2\right)=Mv^2\]
(c) Because there is no external torque acting on the system (in space effects due to friction and air resistance are negligible), so the total angular momentum is constant i.e.
\[\vec {\tau }_{net\ ext}=\frac{d{\vec{L}}_{sys}}{dt}=0\Rightarrow \ L_i=L_f=dMv\]
(d) $L_f=dMv=2\left(Mv_f\frac{d}{4}\right)\Rightarrow v_f=2v$
(e) $K^{'}_{r,tot}=2\frac{1}{2}\left(I_f{\omega }^2_f\right)=M{\left(\frac{d}{4}\right)}^2{\left(\frac{v_f}{\frac{d}{2}}\right)}^2=Mv^2_f=M{\left(2v\right)}^2=4Mv^2$
\[\Rightarrow \ K^{'}_{r,tot}=4K_{r,tot}\]
(f) Use the work - energy theorem $\Delta K=W$
\[K_f-K_i=W\Rightarrow 4Mv^2-Mv^2=W\]
\[\therefore W=3Mv^2\]
The upper end of the string wrapped around the cylinder in the figure below is 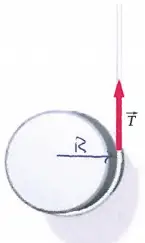 held by a hand that is accelerated upward so that the center of mass of the cylinder does not move as the cylinder spins up. Find:
held by a hand that is accelerated upward so that the center of mass of the cylinder does not move as the cylinder spins up. Find:
(a) The tension in the string
(b) The angular acceleration of the cylinder
(c) The acceleration of the hand.
(a) Draw a free body diagram as below and apply Newton's 2${}^{nd}$ law to 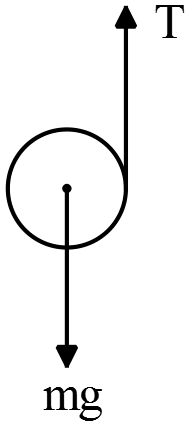 it:
it:
\[\Sigma \vec F_y=ma_{lin}=0\]
\[T-mg=0\Rightarrow T=mg\]
Where $a_{lin}$ is the acceleration of the center of mass of the cylinder that is stated does not move.
(b) The free body diagram shows that the tension of the string causes the rotation of the cylinder ($mg$ acts at the center of mass, so it does not provide a torque about the center of mass) so Newton's 2${}^{nd}$ law for rotating objects states:
\[\Sigma \tau =I\alpha \Rightarrow \ TR=I\alpha \Rightarrow \alpha =\frac{TR}{I}=\frac{TR}{\frac{1}{2}mR^2}=\frac{2T}{mR}\]
(c) The angular acceleration is related to the tangential acceleration of the cylinder via
\[a=\alpha R\Rightarrow a=\frac{2T}{m}\]
A force $\vec{F}=3\hat{i}-2\hat{j}\ \mathrm{(N)}$ acts at a location $\vec{r}=1\ \hat{i}+2\hat{j}\ \mathrm{(m)}$ on an object. What is the torque that this force applies about an axis through the origin perpendicular to the $xy$ plane?
By definition, the torque acts on an object is $\vec{\tau }=\vec{r}\times \vec{F}$. There are two solutions, using a determinant method or direct product method.
Determinant method:
\begin{align*} \vec{\tau }&=\vec{r}\times \vec{F}\\\\&=\left| \begin{array}{ccc} \hat{i} & \hat{j} & \hat{k} \\ 1 & 2 & 0 \\ 3 & -2 & 0 \end{array} \right|\\\\&=\hat{i}\left(2\left(0\right)-\left(0\right)\left(-2\right)\right)-\hat{j}\left(1\left(0\right)-\left(0\right)\left(3\right)\right)\\\\&+\hat{k}\left(\left(1\right)\left(-2\right)-\left(2\right)\left(3\right)\right)\\\\&=-8\hat{k}\quad \mathrm{(N.m)}\end{align*}
Direct product:
\begin{align*} \vec{\tau }=\vec{r}\times \vec{F} &=\left(1\ \hat{i}+2\hat{j}\right)\times \left(3\hat{i}-2\hat{j}\right)\\\\ &=\left(1\right)\left(3\right)\left(\underbrace{\hat{i}\times \hat{i}}_{0}\right)+\left(1\right)\left(-2\right)\left(\underbrace{\hat{i}\times \hat{j}}_{\hat{k}}\right)\\\\&+\left(2\right)\left(3\right)\left(\underbrace{\hat{j}\times \hat{i}}_{-\hat{k}}\right)+\left(2\right)\left(-2\right)\left(\underbrace{\hat{j}\times \hat{j}}_{0}\right)\\\\&= -2\hat{k}+6\left(-\hat{k}\right)=-8\hat{k}\quad {\rm N.m}\end{align*}
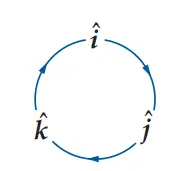
Note: an easy way to remember the cross product of unit vectors are shown in the figure below.
By going around this figure in the clockwise direction, take the positive cross product and vice versa. That is
\[\hat{i}\times \hat{j}=\hat{k}\ \ and\ \ \hat{j}\times \hat{i}=-\hat{k}\]
\[\hat{k}\times \hat{i}=\hat{j}\ \ \ and\ \ \hat{i}\times \hat{k}=-\hat{j}\]
And so on. The cross product of the same unit vectors with each other is always zero.
\[\hat{i}\times \hat{i}=\hat{j}\times \hat{j}=\hat{k}\times \hat{k}=0\]
A man of mass $M=75\,{\rm kg}$ lowers himself down from the top of a building by using a rope wound on a drum (a hollow cylinder of radius $r=0.50\,{\rm m}$ 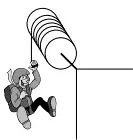 and mass $2M=150\, {\rm kg}$), as shown in the picture. The man and the drum start at rest.
and mass $2M=150\, {\rm kg}$), as shown in the picture. The man and the drum start at rest.
(a) Find the angular acceleration of the drum.
(b) What is the velocity of the man when he has dropped $\mathrm{20\ m}$?
(a) Use Newton's 2${}^{nd}$ law for linear and rotational motion as follows
\[\mathrm{\Sigma }\vec F_y=Ma\Rightarrow T\hat{j}+Mg\left(-\hat{j}\right)=Ma\left(-\hat{j}\right)\Rightarrow T=M(g-a)\]
Where $a$ is the linear acceleration of the man. Recall that in the rotational motion linear acceleration (if the string around the pulley does not slip) is related to the angular acceleration by $a=\alpha r$.
The tension of the rope acts a torque on the cylinder about the horizontal axis passing through the central axis of the cylinder. So $\tau =I\alpha $, where $I$ is the moment of inertia and $\alpha $ is the angular acceleration. By definition of the torque $\vec{\tau }=\vec{r}\times \vec{F}$ we obtain
\[\tau =I\alpha \to rT=I\alpha \Rightarrow \ \alpha =\frac{rT}{I_{cylinder}}\]
Now substitute the tension on the rope into the above relation:
\[\alpha =\frac{rM\left(g-a\right)}{I_{cyli}}=\frac{rM\left(g-\alpha r\right)}{I_{cyli}}\to \alpha \left(I_{cyli}+Mr^2\right)=rMg\]
\[\Rightarrow \alpha =\frac{Mgr}{I_{cyli}+Mr^2}=\frac{Mgr}{\left(2M\right)r^2+Mr^2}=\frac{g}{3r}=6.5\ {\mathrm{s}}^{\mathrm{-}\mathrm{2}}\]
Where we have use the $I_{cylinder}=mr^2$ about central axis.
(b) Use the following kinematic equation to find his speed.
\[v^2-v^2_0=2a\mathrm{\Delta }y\]
Since the man start at rest, $v_0=0$. If we choose the starting point as the base, the man has reached $-y$ below the base. Therefore,
\[v=\sqrt{2\underbrace{a}_{\mathrm{is\ negative}}\mathrm{\Delta }y}=\sqrt{2\left(-\alpha r\right)\mathrm{\Delta }y}=\sqrt{2(-0.5\times 6.5)(-20)}=11.4\ \frac{\mathrm{m}}{\mathrm{s}}\]
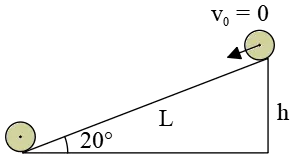
A hoop is released from rest at the top of a plane inclined at $20{}^\circ $ above horizontal. How long does it take the hoop to roll $12.0\ \mathrm{m}$ down the plane? ($I_{hoop}=mr^2$)
In this problem we have a rotational motion. So use the conservation of mechanical energy between initial and final points to find the velocity of the center of mass of the hoop at the bottom of the incline plane.
\[E_{top}=E_{bottom}\]
\[mgh=\underbrace{\frac{1}{2}mv^2_c}_{ \begin{array}{c} trans.\ \ kinetic \\ energy \end{array} }+\underbrace{\frac{1}{2}I{\omega }^2}_{ \begin{array}{c}rotational\ kinetic \\ energy \end{array} }\]
We know that in the rotational motions, the angular and tangential velocities are related together via $v=r\omega $.
By substituting the given values, we obtain
\[mgh=\frac{1}{2}mv^2_c+\frac{1}{2}\left(mr^2\right){\left(\frac{v_c}{r}\right)}^2\Rightarrow v_c=\sqrt{gh}=\sqrt{g(L\,{\sin 20{}^\circ \ })}\]
From the geometry, we have substituted $L\,{\sin 20{}^\circ \ }$ for height of the incline plane $h$.
Now using the kinematic equation $v^2-v^2_0=2ax$, find the acceleration of the CM and then use the equation $y=\frac{1}{2}at^2+v_0t$ to determine the required time.
\[v^2-\underbrace{v^2_0}_{0}=2a\underbrace{x}_{L}\Rightarrow \ a=\frac{gL}{2L}{\sin 20{}^\circ \ }\]
If we suppose the axis parallel to the incline plane as the $y$ axis then $y=L$
\[y=\frac{1}{2}at^2+\underbrace{v_0}_{0}t\to L=\frac{1}{2}at^2\]
\begin{align*} \Rightarrow t & =\sqrt{\frac{2L}{a}}=\sqrt{\frac{2L}{\left(\frac{gL}{2L}\,{\sin 20{}^\circ \ }\right)}}=\sqrt{\frac{4L}{g{\sin 20{}^\circ \ }}}\\& =\sqrt{\frac{4\left(12\right)}{9.8\,{\sin 20{}^\circ \ }}}=3.78\ \mathrm{s}\end{align*}
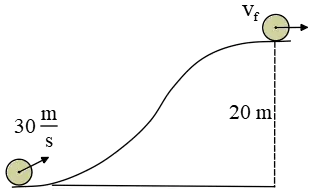
A ball begins rolling up a hill as shown, with no slipping or loss of mechanical energy. Its initial velocity in the horizontal flat region is $30\ \mathrm{m/s}$. The moment of inertia of a solid sphere with mass $M$ and radius $R$ is given by $I=\frac{2}{5}MR^2$.
(a) Calculate its velocity $v_f$ just as it rolls horizontally off the edge of the cliff, which is $20\ \mathrm{m}$ above the initial position.
(b) Calculate the time $t$ that it takes to hit the ground after falling off the cliff.
(c) Calculate the distance from the base of the cliff to the point where the ball hits the ground.
(d) Calculate the (center of mass) velocity that the ball has when it hits the ground.
(a) By using the conservation of mechanical energy, we have
\begin{gather*} E_i=E_f \\\\ \frac 12 Mv_0^2 + \frac 12 I\omega^2 =\frac 12 Mv_f^2 +\frac 12 I \omega^2 +Mgh \end{gather*}
The required condition for rotation without slipping is $v=r\omega $, where $v$ is the tangential velocity of the ball and $r$ is its radius. So \begin{gather*} \frac 12 Mv_0^2+\frac 12 \left(\frac 25 Mr^2 \right) \left(\frac {v_0}r \right)^2 = \frac{1}{2}Mv^2_f+\frac 12 \left(\frac 25 Mr^2\right){\left(\frac{v_f}{r}\right)}^2+Mgh \\\\ \frac{7}{10}Mv^2_0=\frac{7}{10}Mv^2_f+Mgh \\\\ \Rightarrow v_f=\sqrt{v^2_0-\frac{10}{7}gh} \end{gather*} Substituting the numerical values into that, we get \[ v_f=\sqrt{{30}^2-\frac{10}{7}(9.8\times 20)}=25\,\rm m/s \]
(b) After the ball leaves the cliff, it moves along a projectile motion path (with $\alpha =0^\circ $). In the free-fall stage, use the following projectile motion formula \[y=-\frac{1}{2}gt^2+\underbrace{v_0\,{\sin \alpha\ }}_{v_{0y}}t+y_0\]
Here $v_0$ is the initial velocity at the moment of free fall but at the end of the cliff, we have $v_0=v_f$. If above the cliff is chosen as the base then the hitting coordinate is $y=-20\ \mathrm{m}$.
\[-20=-\frac{1}{2}(9.8)t^2+0t+0 \Rightarrow t=2.02\,\rm s\]
(c) In the projectile motion, for finding the traveled horizontal distance, we must use the following kinematic equation
\[x=\underbrace{v_0\,{\cos \alpha \ }}_{v_{0x}}t\Rightarrow x=24.9\times {\cos 0{}^\circ \ }\times 2.02=50\,\rm m\]
(d) In the projectile motion, the projectile has no horizontal acceleration so the only acceleration is the downward free fall acceleration $-g$. Therefore, at any moment first, find the components of the velocity as follows
\[v_x=v_{0x}=v_0\,{\cos \alpha \ }=25\, \rm{m/s}\]
\[v_y=\underbrace{v_{0y}}_{v_0\,{\sin \alpha \ }}-gt=-9.8\times 2.02=-20\ \rm{m/s}\]
Now use the following expression to determine the magnitude of the velocity
\[v=\sqrt{v^2_x+v^2_y}=\sqrt{{25}^2+{\left(-20\right)}^2}=32\ \mathrm{m/s}\]
A block of mass $m$ is being whirled around on a frictionless surface (as 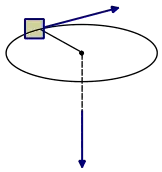 shown), in a circular path of radius $r$.
shown), in a circular path of radius $r$.
(a) Its initial velocity is $v$. If it is pulled in so that the new radius is $r/2$, what is its new velocity?
(b) The initial tension in the string is $T$. What is the final tension after the block is pulled into the new radius of $r/2$?
(a) There are no external torques that act about the vertical axis passing through the hole since the surface is frictionless. So the total angular momentum of the system is conserved. By definition, the angular momentum is $\vec{L}=\vec{r}\times \vec{P}$. Therefore, we have
\[L_i=L_f\Rightarrow rmv=\left(\frac{r}{2}\right)mv^{'}\Rightarrow v^{'}=2v\]
(b) The tension in the string provides the radial acceleration of the block. So the initial tension is
\[T=\frac{mv^2}{r}\]
After the string reaches the new radius $r/2$, the tension in it is
\begin{gather*}T'=\frac{m{v'}^{2}}{r'}=\frac{m{\left(2v\right)}^2}{\frac{r}{2}}=8\frac{mv^2}{r}\\\\ \Rightarrow T'=8T\end{gather*}
A solid disk has a radius of $R=0.1\ \mathrm{m}$ and mass of $M=2.0\ \mathrm{kg}$. it begins rolling up a slope without slipping. The slope is $\theta =20{}^\circ $ above the horizontal, and the disk has an initial speed of $v_0=3.0\ \mathrm{m/s}$. How long it will take for the disk to come to a stop?
First, draw a sketch and show the forces that act on the disk. Consider the direction of the motion of the disk to be the positive direction. Apply Newton's second law in component form for the $x$ axis.

\[\Sigma F_x=ma_{cm}\Rightarrow \ -f_k-mg\,{\sin \theta \ }=ma_{cm}\]
Now apply Newton's second law for the rotational motion about a horizontal axis through the center of mass and perpendicular to ${\vec{v}}_{cm}$.
\[\Sigma {\tau }_i=I_{cm}\alpha \]
\[f_kR=I_{cm}\alpha \]
Other forces ($N, mg\,{\sin \theta \ }$) do not exert torques on the ball since they act on the center of mass or axis of rotation.
Relate $\alpha $ and $a_{cm}$ using the nonslip condition that is $a_{cm}=\alpha R$. Combining these equations, gives
\[\left\{ \begin{array}{rcl}f_k & = &-mg\,{\sin \theta \ }-ma_{cm} \\ f_kR & = & I_{cm}\alpha \\ \alpha & = & \frac{a_{cm}}{R} \end{array}\right.\]
\[\Rightarrow \ -\left(mg\,{\sin \theta \ }+ma_{cm}\right)R=I_{cm}\left(\frac{a_{cm}}{R}\right)\]
Solving for $a_{cm}$, we obtain
\[\Rightarrow \ a_{cm}=-\frac{mg{\sin \theta \ }}{\frac{I_{cm}}{R}+mR}\]
With $a_{cm}$ given by the above equation, use the following kinematic equation to find the time required for the disk to come to a stop ($v=0$).
\[v=v_0+at\Rightarrow 0=v_0+a_{cm}t\Rightarrow t=-\frac{v_0}{a_{cm}}\]
\[\Rightarrow t=\frac{\left(\frac{I_{cm}}{R}+mR\right)v_0}{mg{\sin \theta \ }}\]
The moment of inertia for disk is $I_{cm}=\frac{1}{2}mR^2$, by substituting it into above, we get
\[t=\frac{3v_0}{2\left(g{\sin \theta \ }\right)}=\frac{3\times 3}{2\times 9.8\times {\sin 20{}^\circ \ }}=1.34\ \mathrm{s}\]
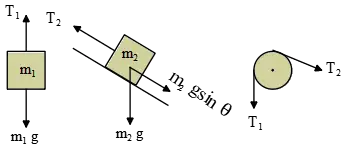
Consider the masses configured on a frictionless inclined plane as shown in the  figure. The pulley is frictionless, has a moment of inertia $I$ and a radius of $R$. Determine the correct expression for the acceleration of $m_1$ in terms of the other quantities. Take the positive direction for the acceleration of $m_1$ to be down.
figure. The pulley is frictionless, has a moment of inertia $I$ and a radius of $R$. Determine the correct expression for the acceleration of $m_1$ in terms of the other quantities. Take the positive direction for the acceleration of $m_1$ to be down.
Apply Newton's second law to each of the masses and pulley in the figure. Since the pulley has the mass (moment of inertia), so the tension on the string is different on each side of the pulley.
\[\Sigma \vec F_1=m_1a\to m_1g-T_1=m_1a\]
\[\Sigma \vec F_2=m_2a\to T_2-m_2g\,{\sin \theta \ }=m_2a\]
Now apply Newton's second law for rotational motion of the pulley as follows
\[\Sigma {\tau }_{ext}=I\alpha \to \ R\left(T_1-T_2\right)=I\left(\frac{a}{R}\right)\]
Where the angular acceleration $\alpha $ is related the linear (center of mass) acceleration by $\alpha =a/R$. Substituting the tensions $T_1$ and $T_2$ into the above equation, we obtain
\[R\left(\left(m_1g-m_1a\right)-\left(m_2a+m_2g{\sin \theta \ }\right)\right)=I\left(\frac{a}{R}\right)\]
\[g\left(m_1-m_2{\sin \theta \ }\right)-a\left(m_1+m_2\right)=\frac{Ia}{R^2}\]
\[a=g\frac{m_1-m_2{\sin \theta \ }}{m_1+m_2+\frac{I}{R^2}}\]
A uniform disk of mass $M=10\ \mathrm{kg}$ and radius $R=0.500\ \mathrm{m}$ is rolling without slipping on a horizontal surface. The rolling disk has a linear (or translational) velocity of $v_{cm}=5\ \mathrm{m/s}$ to the right, as shown in the figure.
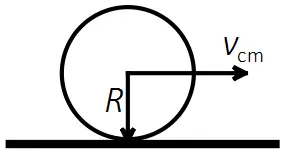
The moment of inertial of the disk rotating about its center of mass is $I=\frac{1}{2}MR^2$.
(a) What is the angular velocity of the rolling disk about its center of mass?
(b) What is the kinetic energy of the rolling disk?
(c) If this disk rolled up a hill, what is the maximum height (above its starting point) that it would reach?
(d) If instead of a rolling disk, you were given a square sliding mass ($m=10\ \mathrm{kg}$) with the same initial linear velocity $v_{cm}=5\ \mathrm{m/s}$, what maximum height could the sliding mass reach? (assuming all surfaces were frictionless)
(a) The angular velocity $\omega $ of a rolling object is related to its tangential velocity by $v=R\omega $. Thus
\[v_{cm}=R\omega \to \omega =\frac{v_{cm}}{R}=\frac{5}{0.5}=10\ \mathrm{rad/s}\]
(b) A rolling object has two kinetic energy, translational and rotational. Therefore,
\[K_{tot}=K_{tran}+K_{rot}=\frac{1}{2}Mv^2_{cm}+\frac{1}{2}I_{cm}{\omega }^2=\frac{1}{2}Mv^2_{cm}+\frac{1}{2}\left(\frac{1}{2}MR^2\right){\omega }^2\]
\[\Rightarrow K_{tot}=\frac{1}{2}\left(10\right){\left(5\right)}^2+\frac{1}{4}\left(10\right){\left(0.5\right)}^2{\left(10\right)}^2=188\ \mathrm{J}\]
(c) Use the conservation of the mechanical energy as $E_i=E_f$.
\[U_i+K_i=U_f+K_f\to \ Mgh_i+K_{i,tot}=MgH+K_{f,tot}\]
Let the lowest point of the hill be the base of the gravitational potential so $U_i=0$. At the final point the rolling object comes to a stop, $K_{f,tot}=0$. Therefore
\[0+188=\left(10\right)\left(9.8\right)H+0\to H=\frac{188}{98}=1.92\mathrm{\ m}\]
(d) Use again the conservation of mechanical energy, but in this case, there is no rotational kinetic energy so
\[E_i=E_f\to mgh_i+\frac{1}{2}mv^2_{cm}=mgH+K_f\]
\[\Rightarrow 0+\frac{1}{2}mv^2_{cm}=mgH+0\Rightarrow H=\frac{v^2_{cm}}{2g}=\frac{5^2}{2\left(9.8\right)}=1.28\ \mathrm{m}\]
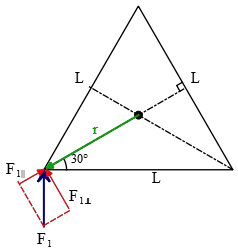
A uniform density metal plate in the shape of an equilateral triangle with each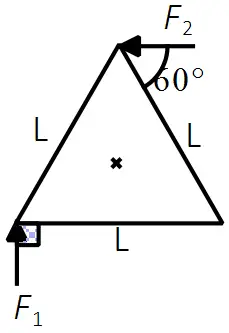 side of length$L=2.00\ \mathrm{m}$, is pivoted about an axis perpendicular to the plate and located at its center. Two small rocket engines are attached to the tips of the triangle that act to create two forces on the triangle $F_1=100\ \mathrm{N}$ and $F_2=100\ \mathrm{N}$ as shown in the figure.
side of length$L=2.00\ \mathrm{m}$, is pivoted about an axis perpendicular to the plate and located at its center. Two small rocket engines are attached to the tips of the triangle that act to create two forces on the triangle $F_1=100\ \mathrm{N}$ and $F_2=100\ \mathrm{N}$ as shown in the figure.
(a) Calculate the torque about the pivot axis from the force $F_1$.
(b) Calculate the torque about the pivot axis from force $F_2$.
(c) Calculate the total(or net) torque about the pivot axis.
(d) You now do an experiment and find that starting from rest, the triangle rotates about its center through one complete revolution in $4.00$ seconds. Using this information, calculate the moment of inertia about its center.
(a) From the geometry first find the moment arm $r$, which is the distance from the pivot point to the point where the force $F$ acts.

\[{\cos 30{}^\circ \ }=\frac{\frac{L}{2}}{r}\Rightarrow r=\frac{L}{2{\cos 30{}^\circ \ }}\]
Now use the definition of the torque $\vec{\tau }=\vec{r}\times \vec{F}=rF\,\sin\ \theta $ to find its magnitude. $\theta $ is the angle between the radial direction and the direction of the force.
\begin{align*}\left|{\vec {\tau }_1}\right|=rF_1\,{\sin \theta \ } &=\left(\frac{L}{2{\cos 30{}^\circ \ }}\right)\left(\underbrace{F_1{\cos 30{}^\circ \ }}_{F_{\bot }}{\sin 90{}^\circ \ }+\underbrace{F_1{\sin 30{}^\circ \ }}_{F_{\parallel }}{\sin 0{}^\circ \ }\right)\\&=\left(\frac{2}{2{\cos 30{}^\circ \ }}\right)\left(100\times {\cos 30{}^\circ \ }\times 1\right)\\& =100\ \mathrm{N.m}\end{align*} Now put your fingers of the right hand along with the moment arm then curl them in the direction of the applied force. The thumb points in the direction of torque (right-hand rule). In this case, the torque is directed in the plane or is clockwise.
(b) Similar as part (a)
\begin{align*} \left|{\vec \tau }_2 \right|&=r_2F_2{\sin \theta \ }\\\\&=\left(\frac{L}{2{\cos 30{}^\circ \ }}\right)\left(\underbrace{F_2{\sin (30+60){}^\circ \ }}_{F_{\bot }}\right){\sin 90{}^\circ \ }\\\\&=\frac{100}{\frac{\sqrt{3}}{2}}\\\\&=\frac{200}{\sqrt{3}}\quad{\rm N.m}\end{align*}
Using the right hand rule, we obtain its direction out of the plane or counter clockwise. Let us assigning a minus sign for clockwise and positive for counter clockwise that is
\[{\vec{\tau }}_1=-100\ \mathrm{N.m\ \ and\ \ } \vec{\tau }_2=+\frac{200}{\sqrt 3}\ \mathrm{N.m}\]
(c) Therefore, the total torque due to the forces $F_1$ and $F_2$ is
\begin{align*} {\vec{\tau }}_{tot}&={\vec{\tau }}_1+{\vec{\tau }}_2\\\\&=-100+\frac{200}{\sqrt{3}}\\\\ &\cong +15\quad \mathrm{N.m\ counter\ clockwise}\end{align*}
(d) The total torque acting on a body equals the product of the moment of inertia of its and its angular acceleration. This is Newton's second law for rotation.
\[{\tau }_{net}=I\alpha \]
Where $\alpha $ is related to the angular coordinate by $\theta ={\theta }_0+\frac{1}{2}\alpha t^2+{\omega }_0t$, which is analogous to those for straight-line motion with constant linear acceleration.
In the problem is said that the body rotates one complete cycle so $\theta =2\pi \ \mathrm{rad}$. Now find the angular acceleration of the triangle as
\begin{align*} \theta &={\theta }_0+\frac{1}{2}\alpha t^2+{\omega }_0t\to 2\pi \\\\&=0+\frac{1}{2}\alpha {\left(4\right)}^2+0\left(4\right)\\\\ \Rightarrow \quad \alpha &=\frac{\pi }{4}\quad {\rm rad/s^2}\end{align*}
Where we have supposed that the initial angular coordinate and velocity be zero.
By substituting $\alpha $ into the equation ${\tau }_{net}$ , we get
\[{\tau }_{net}=I\alpha \Rightarrow I_{cm}=\frac{15}{\frac{\pi }{4}}=\frac{60}{\pi }\ \mathrm{kg.}{\mathrm{m}}^{\mathrm{2}}\]
A cylindrical log of mass $M$ rolls without slipping along the ground. Its center moves along at speed $V$. What is the kinetic energy of the log in terms of $M$ and $V$?
In the rotational motions, the kinetic energy has two contributions one is due to the translational motion and the other is due to the pure rotational motion. Thus
\[K=\underbrace{\frac{1}{2}mV^2}_{trans}+\underbrace{\frac{1}{2}I{\omega }^2}_{rotation}\]
Where $I$ is the moment of inertial of the object.
In the special motion of rolling without slipping, the point of the wheel in contact with the ground is momentarily at rest and the wheel rotates about a rotation axis through the contact points. In this motion, there is a relationship that is unique that is $v=r\omega $ where $r$ is the radial distance from the rotation axis to any point of the wheel.
In the case of wheel or cylinder the geometric center of them is coincident with the center of mass. So the nonslip condition is satisfied as $v_{CM}=R\omega$, where $R$ is the radius of them. Therefore,
\[K=\frac{1}{2}mV^2_{CM}+\frac{1}{2}I{\omega }^2=\frac{1}{2}mV^2_{CM}+\frac{1}{2}\left(\frac{1}{2}mR^2\right){\left(\frac{V_{CM}}{R}\right)}^2=\frac{3}{4}mV^2_{CM}\]
In above, we have used the fact that the moment of inertia of the cylinder about the central axis is $I_{cyl}=\frac{1}{2}mR^2$.
MOST USEFUL FORMULA IN ROTATIONAL MOTION:
Relationship between linear and angular speeds:
\[v=r\omega\]
Angular speed $\omega$ must be measured in radians per second ($\mathrm {rad/s}$).
Tangential acceleration of a point on a rotating object:
\[a_{tra}=\frac {dv}{dt}=r\frac {d\omega}{dt}=r\alpha\]
Definition of moment of inertia:
\[I=m_1r_1^2+m_2r_2^2+\dotsc=\Sigma m_ir_i^2\]
Kinetic energy of a rotating body:
\[K=\frac 1 2 I\omega^2\]
Parallel-Axis theorem:
\[I_P=I_{cm}+Md^2\]
where $d$ is the distance between the center of mass and the axis of rotation.
Definition of torque:
\[\vec \tau=\vec r \times \vec F\]
Rotational analog of Newton's second law:
\[\Sigma \tau=I\alpha\]
Angular momentum of a particle w.r.t a point:
\[\vec L=\vec r\times \vec p=\vec r\times m\vec v\]
Angular momentum of a rotating body around an axis:
\[\vec L=I\vec \omega \]
© 2015 All rights reserved. by Physexams.com
AP® is a trademark registered by the College Board, which is not affiliated with, and does not endorse, this website.
