Position vs. Time Graph: Worked Examples for High Schools
The position vs. time graph aims to analyze and identify the type of motion. In addition, using a position-time graph, one can find displacement, average speed and velocity, and acceleration of motion.
In this article, we want to answer these questions with plenty of worked examples
First of all, we study the displacement vector which is the core concept in studying kinematics.
How to find displacement on a position vs. time graph
As its name indicates, the position-time graph shows the position of a moving object relative to the starting point at each instant of time.
In such graphs, the first quantity, position, is placed on the vertical axis and the other quantity, here time, is on the horizontal axis.
Remember that the difference of initial and final positions of a moving object is defined as displacement in physics, or precisely in math is written as below, \[\Delta x=x_f-x_i\] where $x_f$ is the final position, and $x_i$ is the initial position of the object.
To find the displacement of a moving object on a position-time graph, first, locate two points on the graph, and specify them as initial and final points at that time interval, then find their corresponding positions from the vertical axis.
Next, subtract the initial position from the final one to get the displacement vector using a position vs. time graph.
Example (1): In this example, we want to know how to read a position-time graph. Consider the graph below, and answer the following questions.
(a) When did the object reach 12 m beyond the starting point?
(b) Where was the object after 2 seconds?
(c) Find the displacement in the time interval $3\,{\rm s}$ to $4\,{\rm s}$.
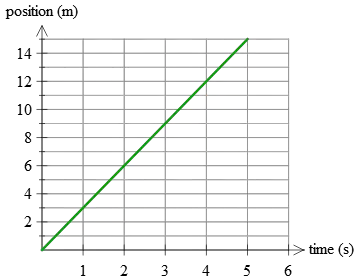
Solution: in a $x-t$ graph, the vertical axis is the position of the moving object at any instant of time relative to the starting point. Here, the object starts its motion from the origin $x=0$ at time $t=0$.
(a) As you can see from the graph, at position $y=12$, the time is exactly $t=4\,{\rm s}$.
(b) Inversely, a point in the horizontal axis (time) is given and wants the corresponding point on the $y$ axis (position). At $t=2\,{\rm s}$, the position is exactly 6 meters.
(c) First, locate the initial and final points as below. initial point has coordinate $(x=9\,{\rm m},t=3\,{\rm s})$ and final point is $(x=12\,{\rm m},t=4\,{\rm s})$. By definition, displacement is subtraction of initial position from final position so \[\Delta x=x_f-x_i=12-9=3\,{\rm m}\]
Now that you learned how to relate the things on a $x-t$ graph together, we want to know how to compute the slopes of a position-time graph.
In the next section, we want to identify the type of motion using its position-versus-time graph.
Overall, we have two types of motion.
Uniform motion, in which the object's velocity is constant at all times. The other is an accelerated motion, in which the object's velocity is steadily increases or decreases.
To determine the type of motion by a position-time graph, we should first know how slope means in such graphs.
What does the slope of a position vs. time graph represent?
In a position-time graph, the position of a moving object relative to the starting point is represented on the vertical axis, and the x-axis shows time. Remember that, the slope of a straight line is defined as the change in vertical axis $\Delta y$ over the change in horizontal axis $\Delta x$, that is, \[Slope = \frac{\text{change in vertical axis}}{\text{change in horizontal axis}}\] On the other side, in physics, the average velocity is also defined as the change in displacement over a specific time interval,\[\bar{v}=\frac{\Delta x}{\Delta t}\] In a position-time graph, the change in the vertical axis is the same as the change in positions, which is displacement, so $\Delta y=\Delta x$. Similarly, the change in the $x$ axis also corresponds to a change in time, $\Delta x=\Delta t$. If we put these substitutions into the ``slope'' formula, we arrive at a geometric definition of average velocity, which is stated below
The slope of the position-time graph is the average velocity
In the following, we want to clarify this statement with some solved examples.
Position vs. Time Graph Examples:
Example (2): In the position-time graph below, find the slope of
(a) The line connecting the points $A$ and $B$.
(b) The line segment of $BC$.
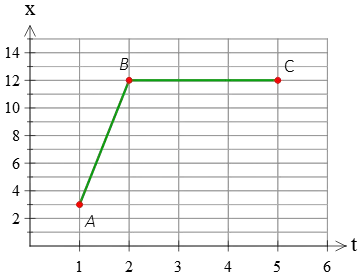
Solution: The slope is defined as the ratio of change in the vertical axis to the change in the horizontal axis.
The ``change'' is also defined as subtraction of the initial point from the final point, say the change in position from point $A$ to $B$ is written as $\Delta x=x_f-x_i$.
(a) From the graph, the vertical coordinates of point B and A are $x_B=12$ and $x_A=3$, respectively. Their corresponding horizontal coordinates are also $t_B=2$ and$t_A=1$. Therefore, the change in vertical axis is \[\Delta x=x_B-x_A=12-3=9\] And the change in horizontal axis is $\Delta t=t_B-t_A=2-1=1$.
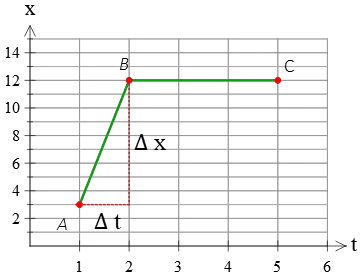
Hence, the ratio of those two changes, gives us the slope between the points A and B \[Slope=\frac{\text{vertical change}}{\text{horizontal change}}=\frac{9}{1}=9\]
(b) Similarly, the slope of line segment of $BC$ is the change in vertical axis $x_C-x_B=12-12=0$ divided by the change in horizontal axis (time) $t_C-t_B=5-2=3$. Thus, the slope is \[Slope=\frac{0}{3}=0\] The slope for this time interval is zero. Thus, we conclude that the slope of any line segment parallel to the horizontal axis is always zero.
Example (3): The position vs. time graph of a runner along a straight line is plotted below. Find:
(a) The average velocity during the first 2 seconds of motion.
(b) The average velocity during the next 1 second of motion.
(c) The average velocity for the next 3 seconds of motion.
(d) The average velocity of the runner over the total travel.
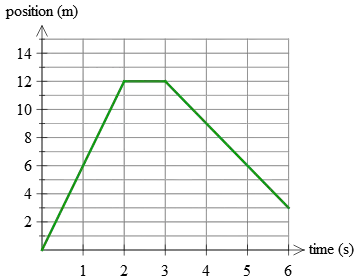
Solution: We know that the slope of the position vs. time graph gives us the average velocity. According to this rule, we must find the slope of lines in each the given time interval.
(a) The slope of the line joining the points $A$ and $B$ is the average velocity in the time interval of the first 2 seconds of motion.
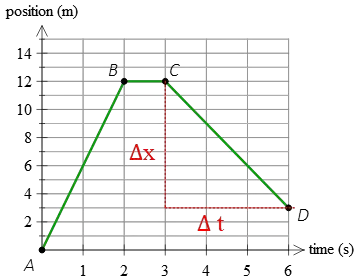
As the graph shows, the change in the vertical axis (position) is $\Delta x=12-0=12\,{\rm m}$, and the change in horizontal axis (time) is $\Delta t=2-0=2\,{\rm s}$. Thus, by definition of slope, we have \begin{align*} Slope &=\frac{\text{change in vertical axis}}{\text{change in horizontal axis}}\\\\&=\frac{12}{2}\\\\&=6\quad {\rm m/s}\end{align*} Hence, the runner's average velocity in this time interval is 6 m/s.
(b) The $x-t$ graph, shows that for the next 1 seconds of motion, there is no change in the vertical axis so its corresponding value is zero. \begin{align*} Slope &=\frac{\text{change in vertical axis}}{\text{change in horizontal axis}}\\\\&=\frac{0}{1}\\\\&=0\end{align*} During this time interval, the slope is zero , and consequently, the average velocity is zero.
In the other words, during this time, the object remains motionless.
(c) Similarly, the slope of the line segment of $CD$ is the ratio of the change in vertical axis (position) $Delta x=x_D-x_C=3-12=-9\,{\rm m}$ to the change in horizontal axis (time) $\Delta t=t_D-t_C=6-3=3\quad{\rm s}$. \[Slope = \frac{-9}{3}=-3\quad{\rm m/s}\] This slope is the same as average velocity. Note that, here, a negative is appeared.
Recall that, the average velocity is a vector quantity in physics.
Its absolute value gives us the magnitude of the velocity, called speed, and its direction along a straight line is shown by a plus or minus sign.
Usually, the minus sign indicates that the moving object moves toward the negative $x$ axis.
(d) The average velocity for the total time interval is the slope of the line connecting the initial point $(x_A=0,t_A=0)$ to the final point $(x_D=3,t_D=6)$. Thus, the slope is \begin{align*} \bar{v}\equiv Slope&=\frac{x_D-x_A}{t_D-t_A}\\\\&=\frac{6-0}{3-0}\\\\&=2\quad{\rm m/s}\end{align*} where $\equiv$ stands for ``is defined as''.
Now, we will address the points that we learned in the above example.
If the slope or average velocity in the time intervals of $[0,1\,{\rm s}]$ and $[{\rm 1\,s, 2\,s}]$ are computed, you will see that the slopes or average velocities are equal. This observation tells us that, between any two arbitrary points in the time interval of 0 to $2\,{\rm s}$, the average velocity is equal.
This observation is also valid for any two points lie in the time interval $[\rm{2\,s,3\,s}]$ and similarly, during 3 seconds to 6 seconds.
When average velocity is constant and unchanging during a time interval, it is said that the motion is uniform.
On the other hand, in each given time interval above, motion is described by a straight line. Combining these two statements, we arrive at the following result:
An object's motion is uniform if and only if its velocity along the motion does not change or its position vs. time graph is composed of straight lines.
Thus, this runner has a uniform motion for the total trip, because its position-time graph has composed of many straight lines in each time interval.
In the next example, we want to introduce another type of motion that is recognized by a position-time graph.
Example (4): The position of a moving car at any instant of time is plotted in a position vs. time graph as below. Find the average velocities in the time intervals of first and next 1 second of motion.
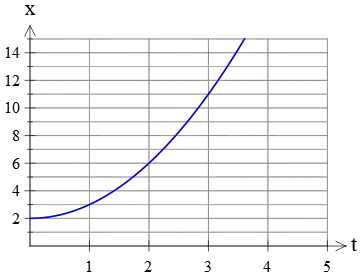
Solution: Here, the position-versus-time graph is not a straight line. How does this mean?
(a) In a position-time graph, the average velocity is the slope of the line between two points.

The slope between points A and B is computed as \begin{align*}\bar{v}_{AB}\equiv slope&=\frac{\Delta x}{\Delta t}\\\\&=\frac{6-3}{2-1}\\\\&=3\quad{\rm m/s}\end{align*} And similarly, the slope between points B and C, which is the same as the average velocity at that time interval, is calculated as \begin{align*}\bar{v}_{BC}\equiv slope&=\frac{\Delta x}{\Delta t}\\\\&=\frac{11-6}{3-2}\\\\&=5\quad{\rm m/s}\end{align*} As you can see, during the two successive equal-time intervals, their corresponding average velocities are not equal.
In other words, as time goes the average velocities are changing. So, we are facing a non-uniform motion.
Recall that the change in velocity over a time interval is defined as an average acceleration.
Combining these two expressions, we arrive at the following important rule:
Curved lines in a position-time graph, indicating an accelerated motion.
In the next example, we want to answer the following example in the form of an example.
How to find instantaneous velocity on a position-time graph?
In the previous example, find the instantaneous velocity at the moment of $t=1\,{\rm s} $
Solution: In the previous example, we found out that the car's velocity is changing. So, a reasonable question is that, what is the velocity at each instant of time, or what is the car's instantaneous velocity?
It is proved that ``the slope of a tangent line to the position-time graph at any instant of time is defined as the instantaneous velocity at that point''.
Thus, to find the instantaneous velocity at a given time from a position vs. time graph, it is sufficient to draw a tangent line at that point and find its slope. But how?
The tangent line is shown in blue color. The slope of this line is the ratio of vertical change $\Delta x$ to the horizontal change $\Delta t$. So \[slope=\frac{9-3}{4-1}=2\quad{\rm m/s}\]
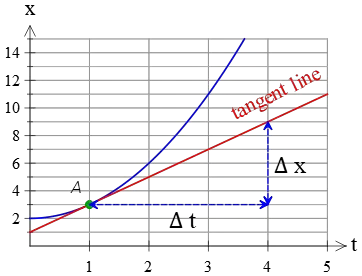
Consequently, in this example, we find that when a position vs. time graph of motion is a curve, the motion is an accelerated motion.
Let's practice another example to find out ``How to find the slope of a tangent line on a position-time graph?''
Example (5): Find the slope of the tangent line at point $A$ in the following graph.
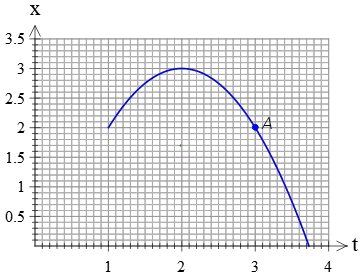
Solution: In this example, we show how to find the slope of a tangent line in a position vs. time graph which yields the instantaneous velocity.
Draw a tangent at point A, such that it intercepts the frame of the graph, as shown in the figure.
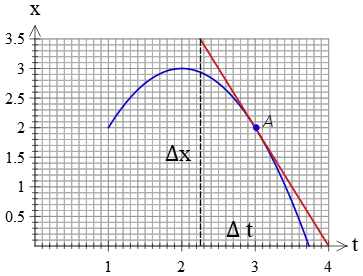
Now, find the change in vertical and horizontal axes. Here, in a paper graph, you see that the change in the vertical axis is $\Delta x=-3.5\,{\rm m}$, and their corresponding horizontal change is $\Delta t=1.75\,{\rm s}$. So, their ratio gives us the slope of tangent line at that point \[slope=\frac{\Delta x}{\Delta t}=\frac{-3.5}{1.75}=-2\quad{\rm m/s}\]
Example (6): A car moves slowly along a straight line according to the following position versus time graph. Interpret the type of motion at different time intervals.

Solution: As the graph shows, the motion is composed of two parts, one is a curve, and the other is a straight line.
In the time interval 0 to $2\,{\rm s}$ seconds, the position-time graph is a curve, indicating a non-uniform motion or an accelerated motion.
In the time interval $2\,{\rm s}$ to $3\,{\rm s}$, we have a straight line, indicating a uniform motion or a motion with a constant velocity.
Thus, a position-time graph tells us about the type of the motion; uniform or accelerated motion.
How to find velocity's direction using a position vs. time graph?
As mentioned, velocity is a vector quantity that has both a magnitude and a direction. Until now, we calculated the magnitude of the velocity, speed, using slopes in a position-time graph.
But is there any way to find out the direction of the velocity from a position versus time graph?
As a rule of thumb, if the angle of slope of the position-time graph is acute, the velocity's direction is positive.
And, if that angle is obtuse or $90^\circ<\alpha<180^\circ$, then the direction of velocity is negative. These angles are shown in the figure below.
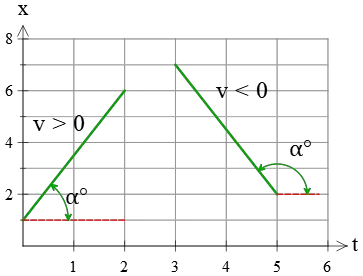
So far, we have obtained valuable information about the velocity of a moving object from the slope of its position-time graph. But, what about the other important kinematics quantity, acceleration?
How to find acceleration from position vs. time graph?
It is difficult to find exactly the acceleration of a moving object from a position-time graph. But, there is an exception, a motion with uniform acceleration.
From kinematics equations, we know that the position of a uniformly accelerating object is written as below \[x=\frac 12 at^2+v_0t+x_0\] where $a$ is its constant acceleration, $v_0$ is initial velocity, and $x_0$ is the initial position relative to a reference.
The plot of this kinematic equation is a quadratic curve (more precise, a parabola) in the position-versus-time graph.
Recall that, acceleration is a vector quantity in physics whose direction, in contrast to its magnitude, is simply found using a position-versus-time graph.
In the following figures, the position-time graphs of two moving objects with different acceleration signs are drawn.
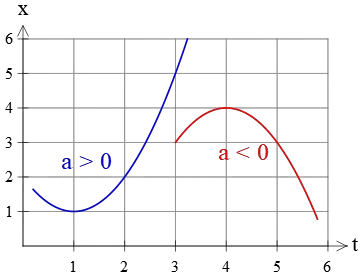
Thus, a curve that bends upward has positive acceleration, and a curve in a position-time graph with a downward bending has negative acceleration.
To learn more about acceleration on a position vs. time graph, read the following related article
How to compute constant acceleration using a position vs. time graph
Example (7): Which of the following diagrams describes a motion in which the object starts from rest and steadily increases its speed?

Solution: Let's start word by word. From rest means that its initial velocity is zero. Steadily increases speed means that its speed is changing but at a constant rate. Thus, we are facing a positive accelerated motion.
Recall that, a tangent line in a position-time graph shows us the instantaneous velocity. And also remember that the position-time graph of a uniform motion (in which velocity is constant), is a straight line.
Thus, from rest translates into a position-time graph as a horizontal tangent line.
The choice IV is incorrect as indicates a constant velocity (uniform) motion.
In choice II, the tangent line (instantaneous velocity) at time $t=0$ is not horizontal, so this item shows a motion having initial velocity.
The choices "I" and "II" both have a horizontal tangent line at time $t=0$, so their initial velocities are zero but there is a difference between them.
Choice II opens upward which means its acceleration is positive, but the choice "I" opens downward which means its acceleration is negative.
In this problem, the moving object's velocity is increasing, so its acceleration must be positive.
Consequently, the correct choice is II.
Example (8): The position vs. time graph of a moving body along a straight line is plotted as below. Find the average velocity in the time interval $t_1=1\,{\rm s}$ and $t_2=4\,{\rm s}$.
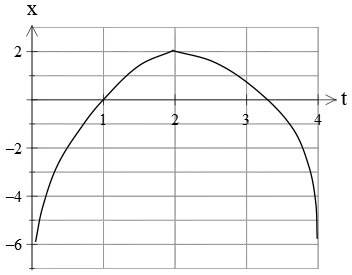
Solution: As the graph shows, the motion is accelerated. Average velocity is also the slope of the line connecting two points on a position vs. time graph.
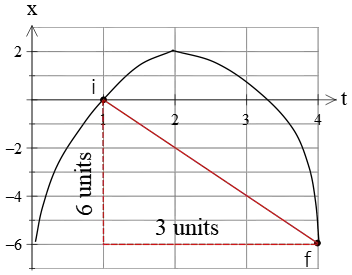
Here, initial and final points have the following coordinate on the position-time graph: initial point $(x_i=0,1\,{\rm s})$ and final point $(x_f=-6\,{\rm m},t_f=4\,{\rm s})$. The slope between these points, average velocity, are computed as below \begin{align*} slope&=\frac{\text{vertical change}}{\text{horizontal change}}\\\\&=\frac{x_f-x_i}{t_f-t_i}\\\\&=\frac{-6-0}{4-1}\\\\&=-2\quad {\rm m/s}\end{align*} Thus, the moving object has an average velocity of 2 m/s toward the negative $x$ axis.
Summary:
Of velocity vs. time graph and position vs. time graph, we can find helpful information about a motion. In this article, we learned how to extract the following information about the type of the motion and its kinematics variables from a $x-t$ graph:
(1) Straight lines indicate a constant velocity motion or uniform motion.
(2) Curve lines show an accelerated motion or a motion with changing velocity.
(3) Displacement between any two points.
(4) Slope of a line segment between any two points on the graph gives average velocity.
(5) Slope of a tangent line at any instant of time gives the instantaneous velocity.
(6) Slopes with acute angle give a positive velocity, and slopes with the obtuse angle a negative velocity.
(7) A curve opening upward has positive acceleration, and a curve opening downward has a negative acceleration.
Author: Ali Nemati
Page Published: 8-12-2021
© 2015 All rights reserved. by Physexams.com
AP® is a trademark registered by the College Board, which is not affiliated with, and does not endorse, this website.
