Work-Energy Theorem Problems and Solutions for High School
In this article, some problems on the work-kinetic energy theorem are presented and solved. In each answer, you can find a detailed tutorial on this topic.
Problems on Work-Energy Theorem:
Problem (1): How much work must be done to stop a $1200-{\rm kg}$ car moving at $99\,{\rm km/h}$ in a straight path.
Solution: The work-kinetic energy theorem states that the net work done on an object is equal to the change in the object's kinetic energy. \[W_{net}=K_2-K_1\] where $K=\frac 12 mv^2$ is the kinetic energy of an object.
In this case, the initial and final velocities of the car are given, so $v_i=99\,{\rm km/h}$ and $v_f=0$. The wanted quantity is the net work done on the car $W_{net}=?$. Applying the work-kinetic energy theorem formula, we have \begin{align*} W_{net}&=\frac 12 m(v_2^2-v_1^2) \\\\ &=\frac 12 (1200)(0-(27.5)^2) \\\\&=453750 \quad {\rm J}\end{align*} where we converted $\rm \frac{km}{h}$ to $\rm \frac{m}{s}$ using the following formula since the SI unit of speed is ${\rm \frac ms}$ \[1\,{\rm \frac{km}{h}}=\frac{1000}{3600}\,{\rm \frac ms}\]
Problem (2): A $6-{\rm kg}$ object has a speed of $2\,{\rm m/s}$ at point A and $4\,{\rm m/s}$ later at point B. Find the total work done on the object as it moves from point A to B.

Solution: The object's mass and its velocities at two different points are given, and we are asked to find the total work done on the object.
All these information guide us to use the work-kinetic energy theorem. Because to find the work done on an object there are two ways, either use the work formula in physics, $W=Fd\cos\theta$, or the work-energy principle. (The first method is a problem on work in physics)
In this case, neither forces acting on the object nor the distance traveled were given, so we are forced to use the work-energy theorem. \begin{align*} W_{net}&=\Delta K\\\\ &=\frac 12 mv_B^2-\frac 12 mv_A^2\\\\ &=\frac 12 (6)(4)^2-\frac 12 (6)(2)^2 \\\\&=36\quad {\rm J}\end{align*}
Problem (3): A $1650-{\rm kg}$ fast car can accelerate from 0 to $27\,{\rm m/s}$ in $2.7\,{\rm s}$.
(a) What is its acceleration?
(b) What is the displacement of the car?
(c) How much work was done on the car?
(d) What is the average force acting on the car over this time interval?
Solution: (a) Initial $v_0=0$ and final velocity $v_f=27\,{\rm m/s}$ are given in a time interval $\Delta t=2.7\,{\rm s}$. Substituting these numerical values into the average acceleration formula, we have \[a=\frac{v_2-v_1}{\Delta t}=\frac{27-0}{2.7}=10\,{\rm m/s^2}\]
(b) This is a kinematics equation problem. To solve this, we use the displacement kinematics equation, $\Delta x=\frac 12 at^2+v_0 t$, so \begin{align*} \Delta x&=\frac 12 at^2+v_0 t\\\\&=\frac 12 (10)(2.7)^2+(0)(2.7) \\\\ &=36.45\quad {\rm m}\end{align*}
(c) This part is related to the work-energy theorem problem. We use this theorem to find the total work done on the car \begin{align*} W_{net}&=\Delta K\\\\ &=\frac 12 m (v_2^2-v_1^2) \\\\&=\frac 12 (1650)(27^2-0) \\\\&=601425\quad {\rm J}\end{align*}
(d) In part (b), the distance traveled by car was found to be $d=36.45\,{\rm m}$. In part (c), the total work over this displacement is obtained, as well.
Use the work formula $W=Fd$, and solve for $F$ to find the average force acting on the car \[F=\frac{W}{d}=\frac{601425}{36.45}=16500\,{\rm N}\]
Further practicing:
Problems on speed, velocity, and acceleration with answers
Problem (4): A $12000-{\rm kg}$ truck initially moving at a constant speed of $126\,{\rm km/h}$ suddenly applies brakes and stops in $12\,{\rm m}$. How much work has been done on the truck over this distance?
Solution: The total work done on the truck involves the work done by the friction force between the truck's tire and the road surface as well as the work done by the brakes to stop the car.
Don't necessary to know the magnitude of these complicated forces to find the total work, instead, as we have the initial and final velocities of the truck, we can use the work-energy theorem to determine the net work done on the truck.
Before using this theorem, first convert the given ${\rm km/h}$ to ${\rm m/s}$ by multiplying it by $\frac{10}{36}$. So, the final velocity in ${\rm m/s}$ is \[126\,{\rm km/h}\times \frac{10}{36}=35\,{\rm m/s}\] Now, applying the work-kinetic energy theorem, we have \begin{align*} W_{net}&=\Delta K\\\\ &=\frac 12 mv_2^2-\frac 12 mv_1^2\\\\ &=\frac 12 (1200)(0)^2-\frac 12 (1200)(35)^2 \\\\&=735\quad {\rm kJ}\end{align*}
Problem (5): A $4600-{\rm kg}$ bus traveling at $26.5\,{\rm m/s}$ can brake and comes to a stop in $46\,{\rm m}$. What is the force applied by the brakes?
Solution: There are two equivalent methods to solve this problem. One is using the work-energy principle, and the other is Newton's second law formula $F=ma$. All information required to use the work-energy theorem is given, so we have \begin{align*} W_{net}&=\Delta K\\\\ &=\frac 12 mv_2^2-\frac 12 mv_1^2\\\\ &=\frac 12 (4600)(0)^2-\frac 12 (4600)(26.5)^2 \\\\&=1615175\quad {\rm J}\end{align*} This is the total work done on the bus by all forces involved. To find the magnitude of those forces we can use the definition of work, $W=Fd$. So, solving for $F$, we have \[F=\frac{W}{d}=\frac{1615175}{46}=35112.5\,{\rm N}\] We can solve this problem from the perspective of a kinematics problem. Using time-independent kinematics equation $v^2-v_0^2=2a\Delta x$, and solving for $a$, we can find the acceleration of the bus \begin{align*} a&=\frac{v^2-v_0^2}{2\Delta x}\\\\&=\frac{0-26.5^2}{2\times 46}\\\\&=7.633\quad {\rm m/s^2}\end{align*} Now, using the formula $F=ma$, the constant force exerts to the car is found to be \[F=(4600)(7.633)=35112.5\] The actual value obtained here is slightly different than the previous result, because we used the rounded value for the acceleration. You can check this out yourself.
Problem (6): A heavy truck of mass $6.5\times 10^4\,{\rm kg}$ is traveling at a constant speed of $12\,{\rm m/s}$.
(a) How much work is required to stop it?
(b) What is the magnitude of the constant force needed to stop it over a displacement of $2.2\,{\rm km}$?
Solution: In all work-energy theorem problems, consider two arbitrary points having known constant velocities and compute the difference in kinetic energies.
According to this principle, the difference in kinetic energies is equal to the total work done on the object to change the object's velocities.
(a) Kinetic energy in physics is defined as $K=\frac 12 mv^2$, so the difference in kinetic energies is determined as below \begin{align*}\Delta K&=\frac 12 m v_2^2-\frac 12 mv_1^2\\\\ &=\frac 12 (65000)(0)^2-\frac 12 (65000)(12)^2\\\\ &=4.68\quad {\rm MJ}\end{align*} where $MJ$ stands for the megajoules which is equal to $10^6\,{\rm J}$. This change in kinetic energy equals the total (net) work done on the truck by all forces acting on it. Hence, \[W_{net}=\Delta K=4.68\quad {\rm MJ}\]
(b) We call all forces involved in stopping the truck, the friction force between the truck's tires and road surface, and the force that the brakes apply, as $F_{tot}$.
In the previous part, the work done by these forces is computed. Assuming that all these forces act on the truck parallel to its displacement, so we can use the work formula $W=Fd$ to find their sum values as below \[F_{net}=\frac{W}{d}=\frac{4.68\times 10^6}{2.6\times 10^3}=1800\,{\rm m}\]
Problem (7): A $2-{\rm kg}$ box is pushed against a slippery surface with a constant force of $50\,{\rm N}$. How far the box must be pushed, starting from rest, so that its final kinetic energy is $380\,{\rm J}$?
Solution: ''from rest'' means initial velocity is zero, $v_0=0$ so its initial kinetic energy is also zero $K_1=0$. The work-energy theorem relates the change in kinetic energy $\Delta K$ of an object to the total (net) work $W_{net}$ done on it. \[W_{net}=\Delta K=K_2-K_1=380\quad {\rm J}\] On the other hand, if we assume all forces involved are constant and parallel to the displacement, then solving the work formula $W_{net}=Fd$ for $d$, gives us the displacement of the box. Thus, \[d=\frac{W_{net}}{F}=\frac{380}{50}=7.6\quad {\rm m}\]
Problem (8): a $2200-{\rm kg}$ car starts its motion from rest at the top of a $20^\circ$ incline. An average friction force of $4000\,{\rm N}$ opposes the car coming down the incline so that the car's speed at the bottom of the path is $4.2\,{\rm m/s}$. How much distance does the car travel down along the incline?
Solution: This is an application of a work-energy problem on an incline. The car starts at rest $v_i=0$, so its initial kinetic energy is $K_i=0$. In the end, the velocity is $v_f=4.2\,{\rm m/s}$ so \[K_f=\frac 12 mv_f^2=19404\,{\rm J}\] The work-kinetic energy principle relates the change in kinetic energy of an object to the work done on all forces acting on it over a displacement $d$. \[\Delta K=\underbrace{F_{net}d}_{W_{net}}\] where we assumed that all forces act in the direction of motion.
Assuming the car starts its motion with the engine off at the top of the inclined plane, then the only forces along the incline are the parallel component of weight, $w_{\parallel}=mg\sin\alpha$, and the friction force $f_k$ (recall these concepts from the section of the inclined plane problems). $\alpha$ is the angle of incline. The free-body diagram of forces is shown below.
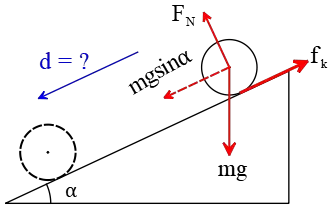
The vector sum of these forces makes the total (net) force act on the car. But what does mean by the vector sum of forces? That is the magnitude of forces along the motion minus the magnitude of forces in the opposite direction of motion.
In this case, the car coming down the incline, so as you can see in the figure, the force $mg\sin 20^\circ$ is along the motion, and the friction force is in the opposite direction.
Thus, adding these forces get the magnitude of total force act on the car \begin{align*} F_{net}&=mg\sin 20^\circ-f_k\\&=(2200)(10)(0.34)-4000\\&=3480\quad {\rm N}\end{align*} After such a long path to find the total force's magnitude, now we apply the work-energy theorem formula and solve for displacement $d$ \begin{align*} \Delta K&=F_{net}d\\\\ 19404-0&=3480\times d\\\\ \Rightarrow d&= 5.57\quad {\rm m}\end{align*}
Practicing more on vectors in physics
Definition of vector in physics
Definition of unit vectors in physics with solved problems
Solved problems on vectors in physics
Problem (9): A $2-{\rm kg}$ crate, from rest, is pulled with a force of $66\,{\rm N}$ by a rope inclined at $32^\circ$. The crate moves through a rough floor, a distance of $10\,{\rm m}$. If an average friction force of $25\,{\rm N}$ opposes the motion, what is the speed of the box at the end of its displacement?
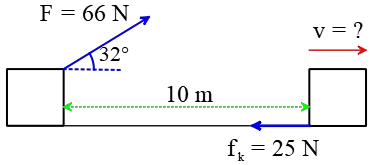
Solution: We want to solve this problem using the work-energy theorem considerations.
To use this principle, we must know the magnitude of total forces acting on the object and its initial and final velocities.
First, draw a free body diagram, and sketch all forces acting on the crate.
As you can see, two forces act on the crate along the motion. One is the parallel component of the pulling force $F_p$, which is obtained by resolving it, and the other is the friction force in the opposite direction of motion.
Algebraic summing these two forces in opposite directions, get the total force acting on the crate. \begin{align*} F_{tot}&=F_{\parallel}-f_k\\\\&=F\cos\alpha-f_k\\\\&=66\cos 32^\circ-25\\\\&=31.1\quad {\rm N}\end{align*} Now, applying the work-energy principle, we have \begin{align*} \Delta K&=W_{net}\\\\K_2-K_1 &=F_{tot}d\\\\K_2-0&=31.1\times 10\\\\ \Rightarrow K_2&=311\quad {\rm J}\end{align*} The kinetic energy in the end of the path is obtained, using kinetic energy formula $K=\frac 12 mv^2$, and solving for $v$, we have \[v=\sqrt{\frac{2K}{m}}=\sqrt{\frac{2\times 311}{2}}=17.6\,{\rm m/s}\] Hence, the care at the end has a speed of about $17.6\,{\rm m/s}$.
Problem (10): A constant force of $100\,{\rm N}$ acts on a $4\,{\rm kg}$ box on a slippery surface. Initially, the speed of the box is $20\,{\rm m/s}$ in the direction of the motion, and $3\,{\rm s}$ later its speed becomes $45\,{\rm m/s}$. Find
(a) the total work done by this external force
(b) The average power delivered by the forces during time interval $3\,{\rm s}$.
Solution: The surface is assumed slippery means the friction force can be neglected. So the only force exerted on the box is the external force $F=100\,{\rm N}$. The box's speed at two different places is given, so its kinetic energies are also known.
(a) The work-kinetic energy principle relates the change in kinetic energy $\Delta K=K_2-K_1$ to the total work done by forces acting on the object. \[\Delta K=W_{tot}=Fd\] So, using this principle, we have \begin{align*} W_{tot}&=K_2-K_1\\\\ &=\frac 12 mv_2^2 -\frac 12 mv_1^2\\\\ &=\frac 12 (4)(45)^2-\frac (4)(20)^2\\\\&=3250\quad {\rm J}\end{align*}
(b) The power delivered by a force is defined as the ratio of the work done by this work to the time taken, so \[P=\frac{W}{t}=\frac{3250}{3}=1083.3\,{\rm W}\]
Problem (11): A $55-{\rm g}$ bullet is fired at a tree trunk. It moves at $575\,{\rm m/s}$ and penetrate the trunk a depth of $4.5\,{\rm cm}$. Find the average frictional forces that act on the bullet to stop it by applying work and energy considerations.
Solution: According to the work and energy theorem, $\Delta K=F_{net}d$, the work done on an object over a distance equals the change in kinetic energy. $F_{net}$ is the total forces that act on the object.
When the bullet is inside the tree trunk, the frictional forces are the only forces that act on it. We call them $f$. Applying the work-energy theorem and solving for $f$, we have \begin{align*} K_2-K_1&=fd\\\\ \frac 12 mv_2^2-\frac 12 mv_1^2 &=fd\\\\ 0-\frac 12 (0.055)(575)^2 &= f\times 4.5 \\\\ \Rightarrow \quad f&=4040\quad {\rm N}\end{align*}
Problem (12): A particle having mass $0.002\,\rm kg$ and charge $-4\,\rm \mu C$ moves from point $A$, where the electric potential is $V_A=+240\,\rm V$, to point $B$, where the electric potential is $+1200\,\rm V$. The particle has speed of $10\,\rm m/s$ at point $A$. What is the particle's speed at point $B$, assuming the only force driving the particle is the electric force?
Solution: This is related to the electric potential problems. According to the work-kinetic energy theorem, $W=\Delta K$, on the other hand, the work done on a charged particle to move it between two points with different potentials is $W=-q\Delta V$, where $\Delta V=V_2-V_1$. Combining these two expressions, we have \[\Delta K=-q\Delta V\] substituting the numerical values into the above expression and solving for the unknown speed $v_B$, we will have \begin{gather*} \frac 12 m(v_B^2-v_A^2)=-q(V_B-V_A) \\\\ \frac{(0.002)(v_B^2-10^2)}{2}=-(-4)(1200-240) \\\\ \Rightarrow \quad \boxed{v_B=1960\,\rm m/s} \end{gather*}
Author: Dr. Ali Nemati
Date Published: 9/23/2021
© 2015 All rights reserved. by Physexams.com
AP® is a trademark registered by the College Board, which is not affiliated with, and does not endorse, this website.
